Pluripotential theory
The natural brand of potential theory in the setting of function theory of several complex variables (cf. also Analytic function). The basic objects are plurisubharmonic functions (cf. also Plurisubharmonic function). These are studied much from the same perspective as subharmonic functions (cf. also Subharmonic function) are studied in potential theory on 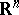 . General references are [a1], [a10], [a16], [a23].
. General references are [a1], [a10], [a16], [a23].
A function  on a domain
on a domain 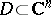 is called plurisubharmonic if it is subharmonic on
is called plurisubharmonic if it is subharmonic on 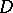 , viewed as a domain in
, viewed as a domain in 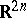 , and if the restriction of
, and if the restriction of  to every complex line in
to every complex line in  is subharmonic (cf. also Plurisubharmonic function; Subharmonic function). If
is subharmonic (cf. also Plurisubharmonic function; Subharmonic function). If  is
is 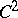 on a domain
on a domain 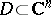 , then
, then  is plurisubharmonic if and only if
is plurisubharmonic if and only if
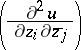 |
is a non-negative Hermitian matrix on 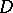 . One denotes the set of plurisubharmonic functions on a domain
. One denotes the set of plurisubharmonic functions on a domain 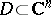 by
by 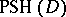 . Plurisubharmonic functions can be defined on domains in complex manifolds via local coordinates (cf. also Analytic manifold).
. Plurisubharmonic functions can be defined on domains in complex manifolds via local coordinates (cf. also Analytic manifold).
Plurisubharmonic functions are precisely the subharmonic functions invariant under a holomorphic change of coordinates. If  is holomorphic on a domain
is holomorphic on a domain 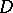 in
in 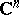 (cf. also Analytic function), then
(cf. also Analytic function), then 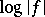 is plurisubharmonic on
is plurisubharmonic on 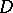 . Moreover, every plurisubharmonic function can locally be written as
. Moreover, every plurisubharmonic function can locally be written as
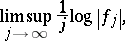 |
for suitable holomorphic functions  , see [a7]. Plurisubharmonic functions were formally introduced by P. Lelong, [a19], and K. Oka, [a22], although related ideas stem from the end of the nineteenth century.
, see [a7]. Plurisubharmonic functions were formally introduced by P. Lelong, [a19], and K. Oka, [a22], although related ideas stem from the end of the nineteenth century.
The analogue of the Laplace operator on domains in 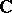 is the Monge–Ampère operator:
is the Monge–Ampère operator:
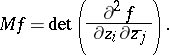 |
This operator is originally only defined for 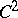 plurisubharmonic functions (cf. also Monge–Ampère equation). Due to the non-linearity of
plurisubharmonic functions (cf. also Monge–Ampère equation). Due to the non-linearity of  it is impossible to extend it to a well-defined operator on all plurisubharmonic functions on a domain
it is impossible to extend it to a well-defined operator on all plurisubharmonic functions on a domain 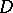 in such a way that
in such a way that 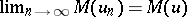 if
if  is a decreasing sequence of plurisubharmonic functions with limit
is a decreasing sequence of plurisubharmonic functions with limit  , see [a9]. Nevertheless, the domain of
, see [a9]. Nevertheless, the domain of 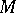 can be enlarged to include all bounded plurisubharmonic functions, [a3]. The most recent result (as of 2000) in this direction is in [a11].
can be enlarged to include all bounded plurisubharmonic functions, [a3]. The most recent result (as of 2000) in this direction is in [a11].
On strongly pseudo-convex domains 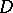 (cf. also Pseudo-convex and pseudo-concave), the following Dirichlet problem for the Monge–Ampère operator was solved by E. Bedford and B.A. Taylor [a3]: Given
(cf. also Pseudo-convex and pseudo-concave), the following Dirichlet problem for the Monge–Ampère operator was solved by E. Bedford and B.A. Taylor [a3]: Given  continuous on
continuous on 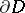 and
and  continuous on
continuous on 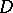 , there exists a continuous plurisubharmonic function
, there exists a continuous plurisubharmonic function  on
on 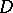 , continuous up to the boundary of
, continuous up to the boundary of 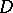 , such that
, such that
 | (a1) |
This result has been extended by weakening the conditions on 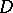 , and replacing
, and replacing 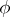 by certain positive measures; see e.g. [a5], [a18]. In [a11], large classes of plurisubharmonic functions on which the Monge–Ampère operator is well defined are determined and necessary and sufficient conditions on a positive measure
by certain positive measures; see e.g. [a5], [a18]. In [a11], large classes of plurisubharmonic functions on which the Monge–Ampère operator is well defined are determined and necessary and sufficient conditions on a positive measure 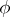 are given, so that the problem (a1) has a solution within such a class.
are given, so that the problem (a1) has a solution within such a class.
The regularity of this Dirichlet problem is quite bad. The following example is due to T. Gamelin and N. Sibony: Let 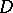 be the unit ball in
be the unit ball in 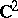 ,
,
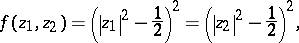 |
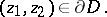 |
Then the function
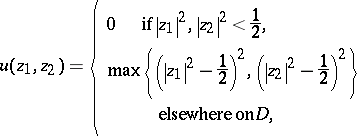 |
satisfies 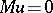 on
on 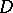 ,
, 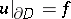 .
.
However, if  and
and  are both smooth and
are both smooth and 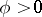 on
on 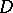 , then was shown in [a8] that there exists a smooth
, then was shown in [a8] that there exists a smooth  satisfying (a1).
satisfying (a1).
There have been defined several capacity functions (cf. also Capacity; Capacity potential) on 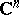 that all share the property that sets of capacity
that all share the property that sets of capacity  are precisely the pluripolar sets, i.e. sets that are locally contained in the
are precisely the pluripolar sets, i.e. sets that are locally contained in the 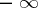 locus of plurisubharmonic functions. See [a4], [a10], [a23], [a24]. Firstly, the classical construction of logarithmic capacity carries over: Let
locus of plurisubharmonic functions. See [a4], [a10], [a23], [a24]. Firstly, the classical construction of logarithmic capacity carries over: Let
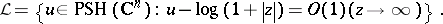 |
For a bounded set 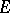 in
in 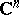 , define the Green function with pole at infinity by
, define the Green function with pole at infinity by
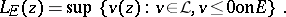 |
Set 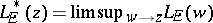 , the upper semi-continuous regularization of
, the upper semi-continuous regularization of  . Then either
. Then either 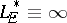 or
or 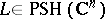 . For
. For 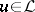 one defines the Robin function on
one defines the Robin function on 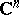 by
by
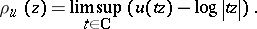 |
Next the logarithmic capacity of 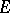 is defined as
is defined as
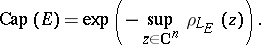 |
It is, however, a non-trivial result that 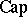 is a Choquet capacity (cf. Capacity), see [a17]. Another important (relative) capacity is the Monge–Ampère capacity introduced by Bedford and Taylor, [a4]. It is defined as follows: Let
is a Choquet capacity (cf. Capacity), see [a17]. Another important (relative) capacity is the Monge–Ampère capacity introduced by Bedford and Taylor, [a4]. It is defined as follows: Let  be a strictly pseudo-convex domain in
be a strictly pseudo-convex domain in 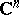 and let
and let 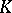 be a compact subset of
be a compact subset of  . The Monge–Ampère capacity of
. The Monge–Ampère capacity of  relative to
relative to  is
is
 |
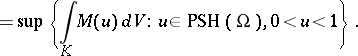 |
If  is an arbitrary subset, one defines
is an arbitrary subset, one defines
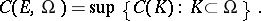 |
It is shown in [a4] that plurisubharmonic functions are quasi-continuous, i.e. continuous outside an open set of arbitrarily small capacity. Another application is a new proof of the following Josefson theorem [a14]: If 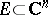 is pluripolar, then there exists a
is pluripolar, then there exists a  with
with 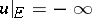 .
.
Although there is no analogue of the Riesz decomposition theorem (cf. also Riesz theorem; Riesz decomposition theorem), there are notions of Green functions.
1) The (Klimek or pluricomplex) Green function on a domain 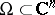 with pole at
with pole at  is the function
is the function
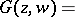 |
 |
If  is hyperconvex, i.e. pseudo-convex and admitting a bounded plurisubharmonic exhaustion function, then
is hyperconvex, i.e. pseudo-convex and admitting a bounded plurisubharmonic exhaustion function, then  is negative and, for
is negative and, for 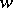 fixed, tends to
fixed, tends to  if
if 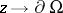 . Moreover,
. Moreover, 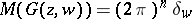 , where
, where 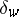 is the Dirac distribution at
is the Dirac distribution at 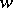 ; see [a12], [a15] for more details.
; see [a12], [a15] for more details.
2) The symmetric Green function on a domain  is the function
is the function
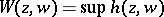 |
where the supremum is taken over
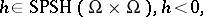 |
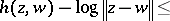 |
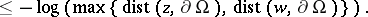 |
Here, 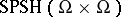 stands for the functions
stands for the functions 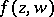 on
on  that are plurisubharmonic in each of the variables
that are plurisubharmonic in each of the variables  ,
, 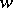 separately, when the other is kept fixed. On strictly pseudo-convex domains
separately, when the other is kept fixed. On strictly pseudo-convex domains  , the symmetric Green function is negative and, for
, the symmetric Green function is negative and, for 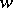 fixed, tends to
fixed, tends to  as
as 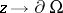 .
.
In general 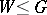 , and there need not be equality, see [a2]. In particular,
, and there need not be equality, see [a2]. In particular, 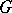 need not be symmetric and
need not be symmetric and 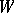 need not be a fundamental solution of
need not be a fundamental solution of 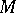 . However, on bounded convex domains
. However, on bounded convex domains 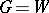 . This is based on work of L. Lempert [a20], [a21] showing that on bounded convex domains in
. This is based on work of L. Lempert [a20], [a21] showing that on bounded convex domains in 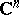 the Kobayashi distance
the Kobayashi distance 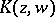 (cf. Hyperbolic metric), the Lempert functional
(cf. Hyperbolic metric), the Lempert functional 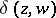 and the Carathéodory distance
and the Carathéodory distance  (cf. also Green function) coincide. The relation between these objects and the Green functions on a domain
(cf. also Green function) coincide. The relation between these objects and the Green functions on a domain  is (see e.g. [a10])
is (see e.g. [a10])
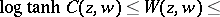 |
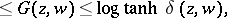 |
where 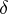 is the Lempert functional
is the Lempert functional
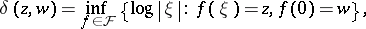 |
with 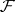 the family of holomorphic mappings from the unit disc in
the family of holomorphic mappings from the unit disc in 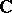 to
to  .
.
The Green function is instrumental in the following result of Z. Błocki and P. Pflug, [a6], which is one of the first applications outside pluripotential theory: Every bounded hyperconvex domain is complete in the Bergman metric (cf. Bergman spaces).
A more elementary proof is given in [a13].
References
| [a1] | E. Bedford, "Survey of pluri-potential theory" , Several Complex Variables (Stockholm, 1987/8) , Math. Notes , 38 , Princeton Univ. Press (1993) pp. 48–97 |
| [a2] | E. Bedford, J.P. Demailly, "Two counterexamples concerning the pluri-complex Green function in 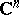 " Indiana Univ. Math. J. , 37 (1988) pp. 865–867 " Indiana Univ. Math. J. , 37 (1988) pp. 865–867 |
| [a3] | E. Bedford, B.A. Taylor, "The Dirichlet problem for a complex Monge–Ampère equation" Invent. Math. , 37 (1976) pp. 1–44 |
| [a4] | E. Bedford, B.A. Taylor, "A new capacity for plurisubharmonic functions" Acta Math. , 149 (1982) pp. 1–40 |
| [a5] | Z. Błocki, "The complex Monge–Ampère equation in hyperconvex domain" Ann. Scuola Norm. Sup. Pisa , 23 (1996) pp. 721–747 |
| [a6] | Z. Błocki, P. Pflug, "Hyperconvexity and Bergman completeness" Nagoya Math. J. , 151 (1998) pp. 221–225 |
| [a7] | H. Bremermann, "On the conjecture of equivalence of plurisubharmonic functions and Hartogs functions" Math. Ann. , 131 (1956) pp. 76–86 |
| [a8] | L. Caffarelli, J.J. Kohn, L. Nirenberg, J. Spruck, "The Dirichlet problem for nonlinear second order elliptic equations. II. Complex Monge–Ampère, and uniform elliptic, equations" Commun. Pure Appl. Math. , 38 (1985) pp. 209–252 |
| [a9] | U. Cegrell, "Discontinuité de l'opérateur de Monge Ampère complexe" C.R. Acad. Sci. Paris Sér. I Math. , 296 (1983) pp. 869–871 |
| [a10] | U. Cegrell, "Capacities in complex analysis" , Vieweg (1988) |
| [a11] | U. Cegrell, "Pluricomplex energy" Acta Math. , 180 (1998) pp. 187–217 |
| [a12] | J.P. Demailly, "Mesures de Monge–Ampère et mesures pluriharmoniques" Math. Z. , 194 (1987) pp. 519–564 |
| [a13] | G. Herbort, "The Bergman metric on hyperconvex domains" Math. Z. , 232 (1999) pp. 183–196 |
| [a14] | B. Josefson, "On the equivalence between locally polar and globally polar sets for plurisubharmonic functions on 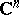 " Ark. Mat. , 16 (1978) pp. 109–115 " Ark. Mat. , 16 (1978) pp. 109–115 |
| [a15] | M. Klimek, "Extremal plurisubharmonic functions and invariant pseudodistances" Bull. Soc. Math. France , 113 (1985) pp. 231–240 |
| [a16] | M. Klimek, "Pluripotential theory" , Clarendon Press/Oxford Univ. Press (1991) |
| [a17] | S. Kołodziej, "The logarithmic capacity in 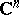 " Ann. Polon. Math. , 48 (1988) pp. 253–267 " Ann. Polon. Math. , 48 (1988) pp. 253–267 |
| [a18] | S. Kołodziej, "The complex Monge–Ampère equation" Acta Math. , 180 (1998) pp. 69–117 |
| [a19] | P. Lelong, "Les fonctions plurisousharmonique" Ann. Sci. École Norm. Sup. , 62 (1945) pp. 301–338 |
| [a20] | L. Lempert, "La métrique de Kobayashi et la représentation des domaines sur la boule" Bull. Soc. Math. France , 109 (1981) pp. 427–474 |
| [a21] | L. Lempert, "Holomorphic retracts and intrinsic metrics in convex domains" Anal. Math. , 8 : 4 (1982) pp. 257–261 |
| [a22] | K. Oka, "Sur les fonctions analytiques de plusieurs variables VI. Domaines pseudoconvexes" Tôhoku Math. J. , 49 (1942) pp. 15–52 |
| [a23] | A. Sadullaev, "Plurisubharmonic measures and capacities on complex manifolds" Russian Math. Surveys , 36 (1981) pp. 61–119 Uspekhi Mat. Nauk. , 36 (1981) pp. 53–105 |
| [a24] | J. Siciak, "Extremal functions and capacities in 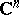 " Sophia Kokyuroku Math. , 14 (1982) " Sophia Kokyuroku Math. , 14 (1982) |
Pluripotential theory. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Pluripotential_theory&oldid=17323