Plücker formulas
Formulas relating external characteristics, i.e. ones corresponding to projective imbeddings, and internal characteristics of algebraic varieties (cf. Algebraic variety). The oldest and best known numerical formulas in algebraic geometry are the Plücker formulas for a planar reduced and irreducible curve 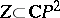 , which has only ordinary double and cuspidal singular points. Let
, which has only ordinary double and cuspidal singular points. Let 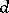 be the degree of the curve
be the degree of the curve  , i.e. the number of points on
, i.e. the number of points on 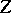 on a straight line in general position in
on a straight line in general position in  , and let
, and let 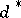 be the class of
be the class of  , i.e. the number of straight lines tangent to
, i.e. the number of straight lines tangent to 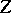 at non-singular points and passing through a given fixed point in general position in
at non-singular points and passing through a given fixed point in general position in 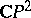 . The two basic Plücker formulas are
. The two basic Plücker formulas are
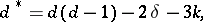 | (1) |
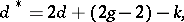 | (2) |
where 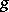 is the genus of the non-singular resolution
is the genus of the non-singular resolution 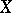 of
of 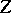 (cf. Resolution of singularities),
(cf. Resolution of singularities), 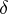 is the number of ordinary double points and
is the number of ordinary double points and 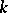 is the number of cuspidal points. Formula (1) reduces to the form
is the number of cuspidal points. Formula (1) reduces to the form 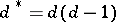 if
if 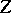 is a non-singular curve.
is a non-singular curve.
Other classical Plücker formulas follow from (1) and (2) by duality. If 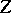 is not a straight line, then the curve
is not a straight line, then the curve 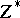 dual to
dual to 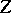 is defined as the closure of the set of tangents to
is defined as the closure of the set of tangents to 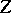 , considered as points in the dual plane
, considered as points in the dual plane  . A theorem due to J. Plücker [3] states that the bi-dual curve
. A theorem due to J. Plücker [3] states that the bi-dual curve 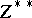 coincides with
coincides with 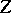 . If it is assumed that
. If it is assumed that 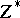 has only
has only  ordinary double points and
ordinary double points and 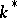 cuspidal singular points, the formulas
cuspidal singular points, the formulas
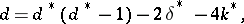 | (1ast) |
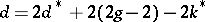 | (2ast) |
are obtained. The number 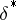 can be interpreted also as the number of bitangents to
can be interpreted also as the number of bitangents to 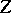 , i.e. straight lines that touch
, i.e. straight lines that touch  at precisely two different non-singular points with contact of order two, while
at precisely two different non-singular points with contact of order two, while 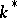 is the number of points of inflection.
is the number of points of inflection.
The four formulas (1), (2), (1ast), and (2ast) are not independent: the fourth follows from any other three. However, any three of them are independent. They also imply the following formulas:
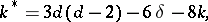 | (3) |
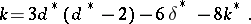 | (3ast) |
These formulas were derived by Plücker together with (1) and (1ast) in 1834–1839.
In the case of a ground field of finite characteristic, the Plücker formulas and the duality theorem are not always correct. For example, in characteristic 2 all the tangents to a conic pass through one point, which is called the strange point on the conic, so the dual curve is a straight line. In characteristic 3 there is a non-singular cubic with only three points of inflection, or even with one (according to the Plücker formulas there should be nine). With the correct interpretation of 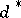 , (1) and (2) remain correct in all characteristics
, (1) and (2) remain correct in all characteristics 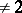 ; in characteristic 2 they must be replaced by
; in characteristic 2 they must be replaced by
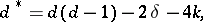 | (1astast) |
 | (2astast) |
There is a generalization of the Plücker formulas to curves in 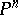 with arbitrary singularities [2], and also to the case of hyperplanes in
with arbitrary singularities [2], and also to the case of hyperplanes in  .
.
References
| [1] | L. Berzolari, "Algebraische Transformationen und Korrespondenzen" , Enzyklopaedie der math. Wissenschaften , 3 : Heft 12 , Teubner (1906) pp. 1787–2218 |
| [2] | P.A. Griffiths, J.E. Harris, "Principles of algebraic geometry" , Wiley (Interscience) (1978) |
| [3] | S.L. Kleiman, "The enumerative theory of singularities" , Real and Complex Singularities (Oslo, 1976). Proc. Nordic Summer School , Sijthoff & Noordhoff (1977) pp. 297–396 |
Comments
See also Plane real algebraic curve for the notions of class, degree, dual, etc. of a curve.
Plücker formulas. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Pl%C3%BCcker_formulas&oldid=13949