Phase space
The totality of all possible instantaneous states of a physical (in the broad sense of the word) system, provided with a definite structure depending on the system being studied and the questions being considered. More specifically, a phase space is a space (a set with an imposed structure) the elements (phase points) of which (conventionally) represent the states of the system (for example, a phase plane). From a mathematical point of view these objects are isomorphic, and therefore one often does not distinguish between the states and the phase points that represent them.
A mathematical formalization of the concept of a "system" of one type or another usually includes as an essential part the definition of the corresponding phase space (or class of phase spaces), which reflects the importance of the concept of a state of a system. The evolution of a system (that is, the change of its states with time) may be strictly deterministic (then it is described by a group or semi-group 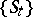 of transformations of the phase space: a state
of transformations of the phase space: a state  goes to
goes to 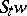 at time
at time 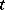 ), or it may have a probabilistic character (be a stochastic process). In the first case it may also be necessary to consider statistical states of systems; for classical (non-quantum) systems they are described by probability distributions on the phase space. The rules that determine the evolution of a system constitute another essential part of the definition of a "system" .
), or it may have a probabilistic character (be a stochastic process). In the first case it may also be necessary to consider statistical states of systems; for classical (non-quantum) systems they are described by probability distributions on the phase space. The rules that determine the evolution of a system constitute another essential part of the definition of a "system" .
In the classical case of a differentiable dynamical system (which includes the main systems considered in analytical mechanics and classical statistical physics), the phase space is a differentiable manifold 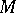 (possibly with singularities and (or) with a boundary). If the dynamical system is given by an autonomous system of ordinary differential equations, then one speaks of the "phase space of the autonomous system" . In this case
(possibly with singularities and (or) with a boundary). If the dynamical system is given by an autonomous system of ordinary differential equations, then one speaks of the "phase space of the autonomous system" . In this case  is that region of a Euclidean or other space where the right-hand sides of the autonomous system are defined. In such a situation the term "phase space" is also used when the solutions are not defined for all
is that region of a Euclidean or other space where the right-hand sides of the autonomous system are defined. In such a situation the term "phase space" is also used when the solutions are not defined for all 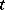 . In addition, there can be given on
. In addition, there can be given on 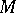 an invariant measure (classically given by a density) or a symplectic structure (the condition that this is preserved under the action of the flow characterizes a Hamiltonian system). In particular, in the dynamics of a system with holonomic, ideal constraints, not explicitly dependent on the time, the phase space is the tangent or cotangent bundle of some manifold — the configuration space. A point of the latter gives a position (configuration) of the system, the tangent vector describes the velocity of its motion (the rate of change of the configuration), and the cotangent vector describes its momentum.
an invariant measure (classically given by a density) or a symplectic structure (the condition that this is preserved under the action of the flow characterizes a Hamiltonian system). In particular, in the dynamics of a system with holonomic, ideal constraints, not explicitly dependent on the time, the phase space is the tangent or cotangent bundle of some manifold — the configuration space. A point of the latter gives a position (configuration) of the system, the tangent vector describes the velocity of its motion (the rate of change of the configuration), and the cotangent vector describes its momentum.
In other areas of the theory of dynamical systems the phase space has the structure of a topological space (in topological dynamics), a measurable space or (more often) a measure space (in ergodic theory). In quantum mechanics the phase space is a complex Hilbert space (though for a quantum system with a classical analogue, the phase space often means the phase space of this analogue). In the theory of stochastic processes the phase space is the measurable space (often with an additional topological, differentiable or vector structure) in which the process takes values. Here one especially talks of a phase space when it is in some sense non-trivial. This is often the case in the theory of Markov processes (cf. Markov process), whereas for those frequently encountered processes with numerical values the phase space reduces simply to 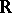 with the standard structure, and it is not especially apt to talk of it. (One should bear in mind that if a stationary stochastic process, in the narrow sense, is interpreted as a dynamical system, then the phase space of the latter is not the same as that of the process.)
with the standard structure, and it is not especially apt to talk of it. (One should bear in mind that if a stationary stochastic process, in the narrow sense, is interpreted as a dynamical system, then the phase space of the latter is not the same as that of the process.)
Comments
See also Dynamical system. A "picture" of the trajectories of a dynamical system in phase space is often referred to as a phase portrait.
References
| [a1] | M.W. Hirsch, S. Smale, "Differential equations, dynamical systems, and linear algebra" , Acad. Press (1974) |
| [a2] | A.G. [A.G. Butkovskii] Butkovsky, "Phase portraits of control dynamical systems" , Kluwer (1990) (Translated from Russian) |
| [a3] | P. Libermann, C.-M. Marle, "Symplectic geometry and analytical mechanics" , Reidel (1987) (Translated from French) |
| [a4] | A.I. Khinchin, "Mathematical foundations of statistical mechanics" , Dover, reprint (1949) (Translated from Russian) |
| [a5] | D. Ruelle, "Statistical mechanics: rigorous results" , Benjamin (1969) |
| [a6] | Y. Choquet-Bruhat, C. DeWitt-Morette, M. Dillard-Bleick, "Analysis, manifolds and physics" , North-Holland (1977) (Translated from French) |
| [a7] | V.I. Arnol'd, "Mathematical methods of classical mechanics" , Springer (1978) (Translated from Russian) |
| [a8] | F.R. [F.R. Gantmakher] Gantmacher, "Lectures in analytical mechanics" , MIR (1975) (Translated from Russian) |
Phase space. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Phase_space&oldid=14737