Pettis integral

An integral of a vector-valued function with respect to a scalar measure, which is a so-called weak integral. It was introduced by B.J. Pettis [1].

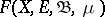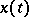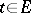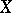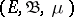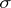Let be the vector space of functions , , with values in the Banach space and given on a set with a countably-additive measure on the -algebra 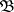of subsets of 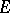. The function is called weakly measurable if for any 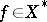the scalar function 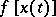is measurable. The function is Pettis integrable over a measurable subset 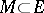if for any 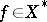the function 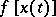is integrable on 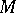and if there exists an element 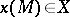such that

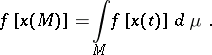Then, by definition,

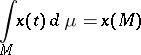is called the Pettis integral. That integral was introduced for the case with the ordinary Lebesgue measure by I.M. Gel'fand [2].

References

[1]	B.J. Pettis, "On integration in vector spaces" Trans. Amer. Math. Soc. , 44 : 2 (1938) pp. 277–304
[2]	I.M. Gel'fand, "Sur un lemme de la théorie des espaces linéaires" Zap. Naukovodosl. Inst. Mat. Mekh. Kharkov. Mat. Tov. , 13 : 1 (1936) pp. 35–40
[3]	T. Hildebrandt, "Integration in abstract spaces" Bull. Amer. Math. Soc. , 59 (1953) pp. 111–139
[4]	E. Hille, R.S. Phillips, "Functional analysis and semi-groups" , Amer. Math. Soc. (1957)

References

[a1]	J. Diestel, J.J. Uhl jr., "Vector measures" , Math. Surveys , 15 , Amer. Math. Soc. (1977)
[a2]	M. Talagrand, "Pettis integral and measure theory" , Mem. Amer. Math. Soc. , 307 , Amer. Math. Soc. (1984)
[a3]	K. Bichteler, "Integration theory (with special attention to vector measures)" , Lect. notes in math. , 315 , Springer (1973)