Perfect compactification

A compactification of a completely-regular space such that the closure in of the boundary of any open set coincides with the boundary of , where is the maximal open set in for which . Equivalent definitions are as follows:

a) for any pair of disjoint open sets ;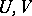

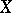b) if a closed set partitions into open sets and , then the closure of in 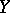partitions into and ;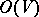

c) does not partition locally at any of its points.

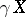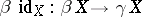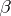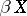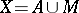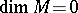A compactification is perfect if and only if the natural mapping is monotone; here is the Stone–Čech compactification of . Also, is the unique perfect compactification of if and only if with a compactum and . The local connectedness of 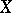implies the local connectedness of any perfect extension 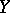of 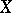satisfying the first axiom of countability (and also the local connectedness of all intermediate extensions). Among all the perfect compactifications of there is a minimal one, , if and only if has at least one compactification with punctiform remainder (cf. Remainder of a space). The remainder in is punctiform and is the maximal such extension among those with punctiform remainder. 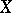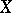Every homeomorphism of extends to a homeomorphism of , and every perfect mapping from onto extends to a mapping from onto (provided 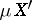exists).

Comments

A space is called punctiform if and only if no compact connected subset contains more than one point.

References

[a1]	A.V. Arkhangel'skii, V.I. Ponomarev, "Fundamentals of general topology: problems and exercises" , Reidel (1984) pp. 232ff (Translated from Russian)