Natural frequencies
Resonances, vibrations, together with natural frequencies, occur everywhere in nature. For example, one associates natural frequencies with musical instruments, with response to dynamic loading of flexible structures, and with spectral lines present in the light originating in a distant part of the Universe.
The simplest case of natural frequencies is illustrated by the vibration of a string. Its deflection  satisfies boundary conditions,
satisfies boundary conditions, 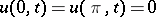 , and an initial condition,
, and an initial condition, 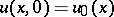 . Its motion is described by the equation
. Its motion is described by the equation 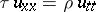 . Separation of variables
. Separation of variables  leads to a pair of equations
leads to a pair of equations 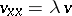 ,
,  .
.
In equations of the type  , where
, where 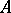 is an operator whose domain is a certain class of functions, the number
is an operator whose domain is a certain class of functions, the number  is called an eigenvalue (cf. Eigen value), and
is called an eigenvalue (cf. Eigen value), and 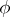 is the corresponding eigenfunction. A (possibly complex) number
is the corresponding eigenfunction. A (possibly complex) number 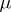 is said to belong to the spectrum
is said to belong to the spectrum 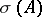 of
of 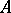 (cf. also Spectrum of an operator) if the "resolvent" operator
(cf. also Spectrum of an operator) if the "resolvent" operator 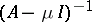 does not exist (cf. also Resolvent).
does not exist (cf. also Resolvent). 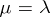 is an eigenvalue if it is a pole of
is an eigenvalue if it is a pole of 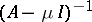 , where
, where 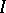 denotes the identity operator. In the equation of a vibrating string, the boundary conditions are satisfied only if
denotes the identity operator. In the equation of a vibrating string, the boundary conditions are satisfied only if 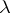 is the square of a natural number
is the square of a natural number  ,
, 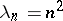 ,
, 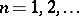 . The natural frequencies
. The natural frequencies  are square roots of the eigenvalues:
are square roots of the eigenvalues:  . The corresponding natural modes
. The corresponding natural modes 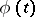 are the trigonometric functions
are the trigonometric functions  . The Euler formula
. The Euler formula 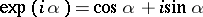 , with
, with 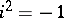 , simplifies many arguments and offers a better insight into vibration and resonance, among others. The eigenvalues are real because in this case the operator
, simplifies many arguments and offers a better insight into vibration and resonance, among others. The eigenvalues are real because in this case the operator 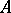 is self-adjoint, meaning that for any pair
is self-adjoint, meaning that for any pair 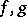 in the domain of
in the domain of 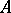 , the inner product has the following symmetry:
, the inner product has the following symmetry:  (cf. also Self-adjoint operator). In simple cases these products can be written as integrals.
(cf. also Self-adjoint operator). In simple cases these products can be written as integrals.
As an example of this abstract theory, consider a free vibration of a membrane occupying a region  . It is modelled by an eigenvalue equation (cf. also Neumann eigenvalue; Rayleigh–Faber–Krahn inequality). Let
. It is modelled by an eigenvalue equation (cf. also Neumann eigenvalue; Rayleigh–Faber–Krahn inequality). Let 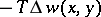 be denoted by
be denoted by  , and
, and 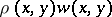 by
by 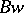 .
.  is the Laplace operator
is the Laplace operator  . The deflection
. The deflection  on the boundary
on the boundary 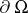 of
of  for all
for all 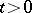 . (Here,
. (Here, 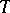 denotes the uniform membrane tension,
denotes the uniform membrane tension, 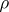 is mass per unit length.) Then
is mass per unit length.) Then  is the disguised equation of motion, with eigenvalue
is the disguised equation of motion, with eigenvalue 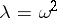 , where
, where 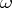 is the natural frequency of vibration.
is the natural frequency of vibration.
One can introduce the following product for arbitrary functions satisfying boundary conditions whose gradients are square integrable in  :
:
 |
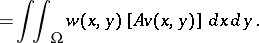 |
Putting 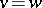 , one obtains an energy equation for a freely vibrating membrane. It connects the two basic energy forms: potential and kinetic. Rayleigh's principle relates the value of the smallest (fundamental) natural frequency of the system to the minimum, attained over all possible forms of vibration, of the ratio of the average kinetic energy over average potential energy, computed over a single cycle of vibration.
, one obtains an energy equation for a freely vibrating membrane. It connects the two basic energy forms: potential and kinetic. Rayleigh's principle relates the value of the smallest (fundamental) natural frequency of the system to the minimum, attained over all possible forms of vibration, of the ratio of the average kinetic energy over average potential energy, computed over a single cycle of vibration.
Note that 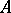 being self-adjoint implies conservation of energy. So the problem with self-adjoint operators is not very realistic: The vibrating string does not know how to stop vibrating. It will go on forever with the same frequency and the same amplitude. However, a real string will insist on dissipating some of its energy and this has to be reflected in the properties of the operator.
being self-adjoint implies conservation of energy. So the problem with self-adjoint operators is not very realistic: The vibrating string does not know how to stop vibrating. It will go on forever with the same frequency and the same amplitude. However, a real string will insist on dissipating some of its energy and this has to be reflected in the properties of the operator.
Generally, a correction is made by inserting first-order differential terms into the differential equation, but not always.
In the example of a vibrating elastic shaft one has (with suitable boundary conditions) a self-adjoint Euler–Bernoulli equation:
 |
S. Timoshenko suggested a fifth-order derivative correction term: 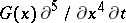 or similar, taking care of small dissipative effects caused by rotational inertia deforming the shape of the cross-sectional area. With this term, and also possible first-order derivative terms included, the operator
or similar, taking care of small dissipative effects caused by rotational inertia deforming the shape of the cross-sectional area. With this term, and also possible first-order derivative terms included, the operator 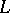 is no longer self-adjoint, and the eigenvalues become complex numbers. Ignoring small damping terms, the equation
is no longer self-adjoint, and the eigenvalues become complex numbers. Ignoring small damping terms, the equation
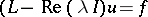 |
can be solved. Here  is an eigenvalue of
is an eigenvalue of 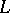 , and
, and 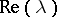 is the real part of
is the real part of 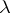 . Then the approximate dissipation-free solution can be written as
. Then the approximate dissipation-free solution can be written as 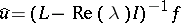 . Observe that the indicated inverse exists, since
. Observe that the indicated inverse exists, since 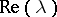 is not an eigenvalue. If
is not an eigenvalue. If 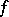 is close to an eigenfunction corresponding to the "true"
is close to an eigenfunction corresponding to the "true" 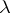 and
and  is very close to the pole of the resolvent, the response
is very close to the pole of the resolvent, the response  may become very large. This is a classical example of natural frequency resonance.
may become very large. This is a classical example of natural frequency resonance.
In the energy-conserving problems described above, the domain of the operator 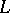 is compact, the inverse
is compact, the inverse 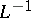 is a compact operator, the spectrum of
is a compact operator, the spectrum of 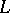 consists of real eigenvalues only, and the only accumulation point for the eigenvalues is at infinity.
consists of real eigenvalues only, and the only accumulation point for the eigenvalues is at infinity.
Complications arise in quantum physics, where, in general, the domain of the operator is not compact and a continuous spectrum is superimposed on the true eigenvalues. Consider the Schrödinger operator 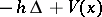 , where
, where  is a potential (cf. also Schrödinger equation). Since boundary values are absent, the spectrum of
is a potential (cf. also Schrödinger equation). Since boundary values are absent, the spectrum of 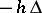 is the positive part of the real line. Since solutions cannot be contained in a compact set, barriers set by the potential which produce a well of minimum energy surrounded by "hills" are not respected. In fact, there is a unique (meromorphic) continuation of the resolvent, whereby the solutions tunnel through the obstacles. This refutes the classical laws of physics, under which particles can be trapped at the bottom of a potential well (corresponding to a minimal energy level).
is the positive part of the real line. Since solutions cannot be contained in a compact set, barriers set by the potential which produce a well of minimum energy surrounded by "hills" are not respected. In fact, there is a unique (meromorphic) continuation of the resolvent, whereby the solutions tunnel through the obstacles. This refutes the classical laws of physics, under which particles can be trapped at the bottom of a potential well (corresponding to a minimal energy level).
References
| [a1] | R. Courant, D. Hilbert, "Methods of mathematical physics" , Interscience (1953) (particularly Chapts. 6–7) |
| [a2] | L.D. Landau, E.M. Lifshitz, "A course in theoretical physics" , 1: Mechanics , Pergamon & Addison-Wesley (1960) (In Russian) |
| [a3] | H. Goldstein, "Classical mechanics" , Addison-Wesley (1950) (particularly Chapt. 10) |
| [a4] | E.C. Titchmarsh, "Eigenfunction expansions associated with second order differential equations" , 1 , Oxford Univ. Press (1958) (Edition: Second) |
| [a5] | J.B. Keller, S. Antman, "Bifurcation theory and nonlinear eigenvalue problesm" , Lecture Notes Courant Inst. Math. Sci. , New York Univ. (1968) |
| [a6] | G. Arfken, "Mathematical methods for physicists" , Acad. Press (1985) (Edition: Third) |
Natural frequencies. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Natural_frequencies&oldid=16484