Menelaus theorem
From Encyclopedia of Mathematics
A theorem on the relations between the lengths of the segments on the sides of a triangle determined by an intersecting straight line. It asserts that if the given line intersects the sides of a triangle  (or their extensions) at the points
(or their extensions) at the points  ,
,  and
and  , then
, then
 |
Menelaus' theorem is a particular case of the Carnot theorem; it can be generalized to the case of a polygon. Thus, suppose that a straight line  intersects the edges
intersects the edges  of a polygon
of a polygon  at the respective points
at the respective points  . Then the following relation is valid:
. Then the following relation is valid:
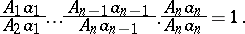 |
The theorem was proved by Menelaus (1st century) and apparently it was known to Euclid (3rd century B.C.).

Figure: m063400a
Comments
References
| [a1] | B.L. van der Waerden, "Science awakening" , 1 , Noordhoff & Oxford Univ. Press (1961) pp. 275 (Translated from Dutch) |
How to Cite This Entry:
Menelaus theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Menelaus_theorem&oldid=19060
Menelaus theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Menelaus_theorem&oldid=19060
This article was adapted from an original article by P.S. Modenov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article