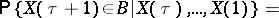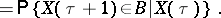for a real-valued stochastic process 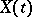 ,
, 
The property that for any set  of times from
of times from  and any Borel set
and any Borel set  ,
,
 | (*) |
with probability 1, that is, the conditional probability distribution of 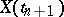 given
given 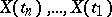 coincides (almost certainly) with the conditional distribution of
coincides (almost certainly) with the conditional distribution of  given
given 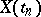 . This can be interpreted as independence of the "future"
. This can be interpreted as independence of the "future"  and the "past"
and the "past"  given the fixed "present"
given the fixed "present"  . Stochastic processes satisfying the property (*) are called Markov processes (cf. Markov process). The Markov property has (under certain additional assumptions) a stronger version, known as the "strong Markov property" . In discrete time
. Stochastic processes satisfying the property (*) are called Markov processes (cf. Markov process). The Markov property has (under certain additional assumptions) a stronger version, known as the "strong Markov property" . In discrete time 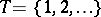 the strong Markov property, which is always true for (Markov) sequences satisfying (*), means that for each stopping time
the strong Markov property, which is always true for (Markov) sequences satisfying (*), means that for each stopping time  (relative to the family of
(relative to the family of 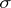 -algebras
-algebras 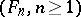 ,
, 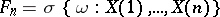 ), with probability one
), with probability one
References
| [1] | I.I. [I.I. Gikhman] Gihman, A.V. [A.V. Skorokhod] Skorohod, "The theory of stochastic processes" , 2 , Springer (1975) (Translated from Russian) |
References
| [a1] | K.L. Chung, "Markov chains with stationary transition probabilities" , Springer (1960) |
| [a2] | J.L. Doob, "Stochastic processes" , Wiley (1953) |
| [a3] | E.B. Dynkin, "Markov processes" , 1 , Springer (1965) (Translated from Russian) |
| [a4] | T.G. Kurtz, "Markov processes" , Wiley (1986) |
| [a5] | W. Feller, "An introduction to probability theory and its applications" , 1–2 , Wiley (1966) |
| [a6] | P. Lévy, "Processus stochastiques et mouvement Brownien" , Gauthier-Villars (1965) |
| [a7] | M. Loève, "Probability theory" , II , Springer (1978) |
How to Cite This Entry:
Markov property. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Markov_property&oldid=11571
This article was adapted from an original article by A.N. Shiryaev (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098.
See original article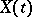 ,
, 
 of times from
of times from  and any Borel set
and any Borel set  ,
,

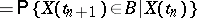
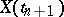 given
given 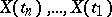 coincides (almost certainly) with the conditional distribution of
coincides (almost certainly) with the conditional distribution of  given
given 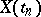 . This can be interpreted as independence of the "future"
. This can be interpreted as independence of the "future"  and the "past"
and the "past"  given the fixed "present"
given the fixed "present"  . Stochastic processes satisfying the property (*) are called Markov processes (cf. Markov process). The Markov property has (under certain additional assumptions) a stronger version, known as the "strong Markov property" . In discrete time
. Stochastic processes satisfying the property (*) are called Markov processes (cf. Markov process). The Markov property has (under certain additional assumptions) a stronger version, known as the "strong Markov property" . In discrete time 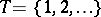 the strong Markov property, which is always true for (Markov) sequences satisfying (*), means that for each stopping time
the strong Markov property, which is always true for (Markov) sequences satisfying (*), means that for each stopping time  (relative to the family of
(relative to the family of 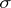 -algebras
-algebras 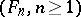 ,
, 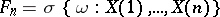 ), with probability one
), with probability one