Loss function
From Encyclopedia of Mathematics
In a problem of statistical decision making, a non-negative function indicating the loss (cost) to an experimenter given a particular state of the world and a particular decision. Let  be a random variable taking values in a sample space
be a random variable taking values in a sample space  ,
,  , and let
, and let 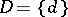 be the space of all possible decisions that can be taken on the basis of an observed
be the space of all possible decisions that can be taken on the basis of an observed  . In the theory of statistical decision functions, any non-negative function
. In the theory of statistical decision functions, any non-negative function  defined on
defined on  is called a loss function. The value of a loss function
is called a loss function. The value of a loss function 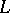 at an arbitrary point
at an arbitrary point  is interpreted as the cost incurred by taking a decision
is interpreted as the cost incurred by taking a decision 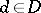 , when the true parameter is
, when the true parameter is 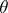 ,
, 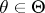 .
.
References
| [1] | A. Wald, "Statistical decision functions" , Wiley (1950) |
| [2] | E.L. Lehmann, "Testing statistical hypotheses" , Wiley (1986) |
Comments
References
| [a1] | J.O. Berger, "Statistical decision theory and Bayesian analysis" , Springer (1985) |
How to Cite This Entry:
Loss function. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Loss_function&oldid=13130
Loss function. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Loss_function&oldid=13130
This article was adapted from an original article by M.S. Nikulin (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article