Logarithmic normal distribution
The continuous probability distribution, concentrated on 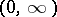 , with density
, with density
 | (*) |
where  ,
,  . A random variable
. A random variable  is subject to the logarithmic normal distribution with density (*) if its logarithm
is subject to the logarithmic normal distribution with density (*) if its logarithm 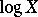 has the normal distribution with parameters
has the normal distribution with parameters  and
and  . Thus,
. Thus,  and
and  . The logarithmic normal distribution is a unimodal distribution and has positive asymmetry. The moments of a random variable
. The logarithmic normal distribution is a unimodal distribution and has positive asymmetry. The moments of a random variable 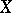 with logarithmic normal distribution with parameters
with logarithmic normal distribution with parameters  and
and  are given by the formula
are given by the formula
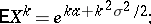 |
hence the mean and variance are, respectively, equal to
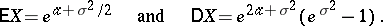 |
The logarithmic normal distribution is one of the simplest examples of a distribution that is not defined uniquely by its moments. The properties of the logarithmic normal distribution are determined by the properties of the corresponding normal distribution. An important property of the logarithmic normal distribution is the following: The product of independent random variables with a logarithmic normal distribution is again subject to a logarithmic normal distribution. There is an analogue of the central limit theorem: The distribution of the product of  independent positive random variables tends, under certain general conditions, to a logarithmic normal distribution as
independent positive random variables tends, under certain general conditions, to a logarithmic normal distribution as  . The logarithmic normal distribution arises as a limit distribution and in certain other schemes (for example, in models of branching of particles, models of growth, etc.).
. The logarithmic normal distribution arises as a limit distribution and in certain other schemes (for example, in models of branching of particles, models of growth, etc.).
References
| [1] | A.N. Kolmogorov, "Ueber das logarithmische normale Verteilungsgesetz der Dimensionen der Teilchen bei Zerstückelung" Dokl. Akad. Nauk SSSR , 31 : 2 (1941) pp. 99–101 |
| [2] | H. Cramér, "Mathematical methods of statistics" , Princeton Univ. Press (1946) |
| [3] | J. Aitchison, J.A.C. Brown, "The lognormal distribution" , Cambridge Univ. Press (1957) |
Comments
The logarithmic normal distribution, or lognormal distribution, is infinitely divisible (cf. Infinitely-divisible distribution), cf. [a2].
References
| [a1] | N.L. Johnson, S. Kotz, "Distributions in statistics" , 1 , Houghton Mifflin (1970) |
| [a2] | O. Thorin, "On the infinite divisibility of the lognormal distribution" Skand. Aktuartidskr. : 3 (1977) pp. 121–148 |
Logarithmic normal distribution. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Logarithmic_normal_distribution&oldid=14299