Locally compact space

A topological space at every point of which there is a neighbourhood with compact closure. A locally compact Hausdorff space is a completely-regular space. The partially ordered set of all its Hausdorff compactifications (cf. Compactification) is a complete lattice. Its minimal element is the Aleksandrov compactification . The class of locally compact Hausdorff spaces coincides with the class of open subsets of Hausdorff compacta. For a locally compact Hausdorff space 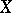its remainder in any Hausdorff compactification is a Hausdorff compactum. Every connected paracompact locally compact space is the sum of countably many compact subsets.

The most important example of a locally compact space is -dimensional Euclidean space. A topological Hausdorff vector space (not reducing to the zero element) over a complete non-discretely normed division ring is locally compact if and only if is locally compact and is finite-dimensional over .

Comments

A product of topological spaces is locally compact if and only if each separate coordinate space is locally compact and all but finitely many are compact.

References

[a1]	J.L. Kelley, "General topology" , v. Nostrand (1955) pp. 146–147