Lobachevskii function
Angle of parallelism
The angle of parallelism in Lobachevskii geometry is a function that expresses the angle $ \alpha $ between the line $ u _ {1} $ (or $ u _ {2} $) (see Fig.) and the segment $ OA $ perpendicular to a line $ a $ parallel to $ u _ {1} $( or $ u _ {2} $) in terms of the length $ l $ of the segment $ OA $:
$$ \alpha = \Pi(l) = 2 { \mathop{\rm arc} \mathop{\rm tan} } e ^ {- l / R } , $$
where $R$ is a positive constant that corresponds to the scale of measurement of distances.
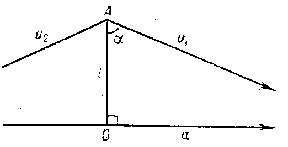
Figure: l060020a
The Lobachevskii function is a continuous monotone decreasing function with values between $\pi/2$ and 0:
$$ \lim\limits _ {l \rightarrow 0 } \Pi ( l) = \frac \pi {2} ,\ \lim\limits _ { l \rightarrow \infty } \Pi ( l) = 0. $$
It was introduced by N.I. Lobachevskii in 1826.
Second meaning
The special function (cf. Special functions) defined for real $x$ by
$$ L(x) = - \int\limits_{0}^{x} \mathop{\rm ln} \cos t dt . $$
The Lobachevskii function can be represented as a series
$$ L(x) = x \mathop{\rm ln} 2 - \frac{1}{2} \sum _ { k= 1} ^ {\infty } (- 1) ^ {k- 1} \frac{\sin 2kx }{k ^ {2} } . $$
The main relations are:
$$ L ( - x ) = - L ( x) ,\ - \frac \pi {2} \leq x \leq \frac \pi {2}, $$
$$ L ( \pi - x ) = \pi \mathop{\rm ln} 2 - L ( x) , $$
$$ L ( \pi + x ) = \pi \mathop{\rm ln} 2 + L ( x) . $$
It was introduced by N.I. Lobachevskii in 1829.
Comments
For the Lobachevskii function in the sense of 1) (i.e. the angle of parallelism) see also [a1]–[a4].
For Lobachevskii's function as defined in 2) see also [a5].
References
| [1] | V.F. Kagan, "Foundations of geometry" , 1 , Moscow-Leningrad (1949) (In Russian) |
| [2] | N.V. Efimov, "Höhere Geometrie" , Deutsch. Verlag Wissenschaft. (1960) (Translated from Russian) |
| [1] | I.M. Ryzhik, I.S. Gradshtein, "Tables of integrals, series, and products" , Acad. Press (1980) (Translated from Russian) |
| [a1] | M. Greenberg, "Euclidean and non-Euclidean geometries" , Freeman (1974) |
| [a2] | H.S.M. Coxeter, "Parallel lines" Canad. Math. Bull. , 21 (1978) pp. 385–397 |
| [a3] | H.S.M. Coxeter, "Non-Euclidean geometry" , Univ. Toronto Press (1957) |
| [a4] | R. Bonola, "Non-Euclidean geometry" , Dover, reprint (1955) (Translated from Italian) |
| [a5] | H.S.M. Coxeter, "Twelve geometric esays" , Carbondale (1968) pp. Chapt. 1 |
Lobachevskii function. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Lobachevskii_function&oldid=53908