Liouville equation

The Liouville equation
 | (a1) |
is a non-linear partial differential equation (cf. Differential equation, partial) that can be linearized and subsequently solved. Namely, it can be transformed into the linear wave equation
 | (a2) |
by any of the following two differential substitutions (see [a1], formulas (4) and (2)):
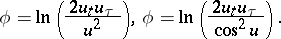 | (a3) |
In other words, the formulas (a3) provide the general solution to the Liouville equation, in terms of the well-known general solution  of the wave equation (a2).
of the wave equation (a2).
The Liouville equation appears also in Lie's classification [a2] of second-order differential equations of the form
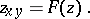 | (a4) |
For the complete classification, see [a4].
The Liouville equation (a1) is invariant under the infinite group of point transformations
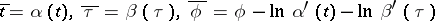 | (a5) |
with arbitrary invertible differentiable functions 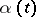 and
and  . The infinitesimal generator of this group is:
. The infinitesimal generator of this group is:
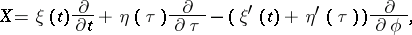 |
where 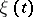 ,
, 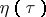 are arbitrary functions and
are arbitrary functions and  ,
, 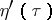 are their first derivatives. It is shown in [a2] that the equation (a4), and in particular the Liouville equation, does not admit non-trivial (i.e. non-point) Lie tangent transformations.
are their first derivatives. It is shown in [a2] that the equation (a4), and in particular the Liouville equation, does not admit non-trivial (i.e. non-point) Lie tangent transformations.
In addition to the transformations (a3), it is known (see, e.g., [a3]) that the Liouville equation is related with the wave equation (a2) by the following Bäcklund transformation:
 |
By letting 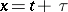 ,
, 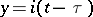 in (a1), (a2) and (a3), where
in (a1), (a2) and (a3), where  , one can transform the elliptic Liouville equation
, one can transform the elliptic Liouville equation 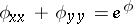 into the Laplace equation
into the Laplace equation 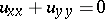 .
.
References
| [a1] | J. Liouville, "Sur l'équation aux différences partielles 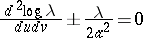 " J. Math. Pures Appl. , 8 (1853) pp. 71–72 " J. Math. Pures Appl. , 8 (1853) pp. 71–72 |
| [a2] | S. Lie, "Discussion der Differentialgleichung 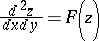 " Arch. for Math. , 6 (1881) pp. 112–124 (Reprinted as: S. Lie: Gesammelte Abhandlungen, Vol. 3, pp. 469–478) " Arch. for Math. , 6 (1881) pp. 112–124 (Reprinted as: S. Lie: Gesammelte Abhandlungen, Vol. 3, pp. 469–478) |
| [a3] | N.H. Ibragimov, "Transformation groups applied to mathematical physics" , Reidel (1985) (In Russian) |
| [a4] | "CRC Handbook of Lie group analysis of differential equations" N.H. Ibragimov (ed.) , 1 , CRC (1994) pp. Chapt. 12.3 |
Liouville equation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Liouville_equation&oldid=18774