Likelihood-ratio test

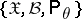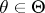A statistical test based on the ratio of the greatest values of the likelihood functions under the hypothesis being tested and under all possible states of nature. Let a random variable have values in the sample space , , let the family of measures be absolutely continuous with respect to a 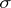-finite measure and let 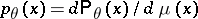. 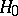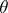Suppose it is necessary, via a realization of the random variable , to test the composite hypothesis according to which the unknown true value of the parameter belongs to the set 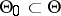, against the composite alternative 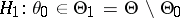. According to the likelihood-ratio test with significance level 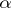, , the hypothesis 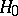has to be rejected if as a result of the experiment it turns out that 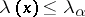, where is the statistic of the likelihood-ratio test, defined by:

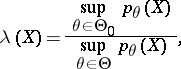while 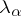is the critical level determined by the condition that the size of the test,

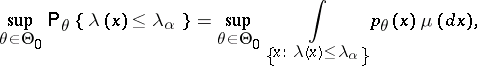is equal to . In particular, if the set contains only two points 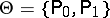, with densities 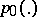and respectively, corresponding to the concurrent hypotheses which, in this case, are simple, then the statistic of the likelihood-ratio test is simply

According to the likelihood-ratio test with significance level 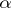, the hypothesis 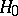has to be rejected if 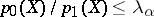, where the number 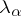, , is determined by the condition

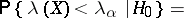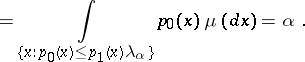The (generalized) likelihood-ratio test was proposed by J. Neyman and E.S. Pearson in 1928. They also proved (1933) that of all level- tests for testing one simple hypothesis against another, the likelihood-ratio test is the most powerful (see Neyman–Pearson lemma).

References

[1]	J. Neyman, E.S. Pearson, "Joint statistical papers" , Cambridge Univ. Press (1967)
[2]	E.L. Lehmann, "Testing statistical hypotheses" , Wiley (1986)

Comments

This test is also called the generalized likelihood-ratio test, or the Wald test.