Lie-admissible algebra
A (non-associative) algebra (cf. Non-associative rings and algebras) whose commutator algebra becomes a Lie algebra. It was first introduced by A.A. Albert in 1948 and originated from one of the defining identities for standard algebras [a1]. For an algebra 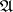 over a field
over a field 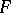 , the commutator algebra
, the commutator algebra 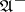 of
of 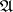 is the anti-commutative algebra with multiplication
is the anti-commutative algebra with multiplication 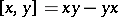 defined on the vector space
defined on the vector space  . If
. If  is a Lie algebra, i.e.
is a Lie algebra, i.e.  satisfies the Jacobi identity
satisfies the Jacobi identity 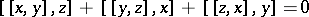 , then
, then 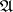 is called Lie-admissible (LA). Much of the structure theory of Lie-admissible algebras has been carried out initially under additional conditions such as the flexible identity
is called Lie-admissible (LA). Much of the structure theory of Lie-admissible algebras has been carried out initially under additional conditions such as the flexible identity 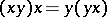 or power-associativity (i.e. every element generates an associative subalgebra), or both. An algebra
or power-associativity (i.e. every element generates an associative subalgebra), or both. An algebra 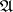 is flexible Lie-admissible (FLA) if and only if it satisfies the identity
is flexible Lie-admissible (FLA) if and only if it satisfies the identity
 | (a1) |
if and only if the mapping 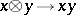 is a Lie module homomorphism of
is a Lie module homomorphism of 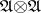 to
to 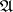 for
for 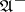 under the adjoint action. For this reason, representations of Lie algebras play a main role in the structure theory of FLA algebras [a2]. Lie and associative algebras are examples of FLA algebras.
under the adjoint action. For this reason, representations of Lie algebras play a main role in the structure theory of FLA algebras [a2]. Lie and associative algebras are examples of FLA algebras.
Beginning with Albert's problem of classifying all power-associative FLA algebras 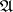 with
with 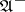 semi-simple [a1], a common theme of the structure theory in various mathematical, physical and geometrical settings has been to focus on the case of a prescribed Lie algebra structure on
semi-simple [a1], a common theme of the structure theory in various mathematical, physical and geometrical settings has been to focus on the case of a prescribed Lie algebra structure on 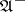 . Albert's problem was first solved in 1962 for finite-dimensional algebras
. Albert's problem was first solved in 1962 for finite-dimensional algebras 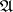 over an algebraically closed field
over an algebraically closed field 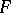 of characteristic 0, and such algebras turned out to be Lie algebras [a3]. This result was extended to the case of
of characteristic 0, and such algebras turned out to be Lie algebras [a3]. This result was extended to the case of 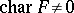 when
when 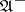 is a classical Lie algebra or a generalized Witt algebra [a2], [a4] (cf. Witt algebra). In 1981, these algebras were classified without the assumption of power-associativity [a5], [a6]: When
is a classical Lie algebra or a generalized Witt algebra [a2], [a4] (cf. Witt algebra). In 1981, these algebras were classified without the assumption of power-associativity [a5], [a6]: When  is simple over the base field
is simple over the base field 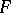 as above, the multiplication
as above, the multiplication  in
in 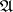 is given by
is given by
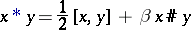 | (a2) |
for a fixed scalar 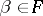 , where
, where  for
for 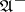 not of type
not of type 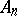 (
(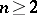 ), and for
), and for 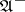 of type
of type 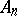 (
(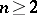 ),
), 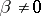 and
and 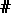 is defined on
is defined on 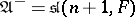 by
by
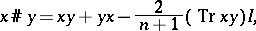 |
where  denotes the matrix product of
denotes the matrix product of  and
and 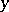 and
and  is the identity matrix. Thus, the algebra
is the identity matrix. Thus, the algebra 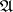 with
with 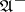 of type
of type 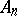 (
(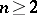 ) can not be power-associative. If
) can not be power-associative. If 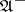 is semi-simple,
is semi-simple, 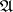 is a direct sum of simple algebras given by (a2). The classification was extended to the case where the solvable radical (cf. Radical of rings and algebras) of
is a direct sum of simple algebras given by (a2). The classification was extended to the case where the solvable radical (cf. Radical of rings and algebras) of 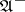 is a direct summand of
is a direct summand of 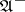 or Abelian [a2]. In 1984, the algebras
or Abelian [a2]. In 1984, the algebras 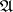 in Albert's problem were determined in the absence of flexibility [a7]: If
in Albert's problem were determined in the absence of flexibility [a7]: If 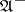 is semi-simple with decomposition
is semi-simple with decomposition 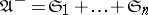 (
(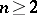 ), where the
), where the 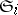 are simple ideals of
are simple ideals of 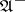 , then the multiplication
, then the multiplication  in
in 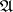 has the form
has the form 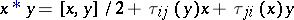 for
for 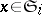 ,
, 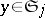 , where the
, where the 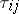 are linear functionals on the
are linear functionals on the 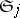 and satisfy certain conditions prescribed in terms of graphs having 2, 3 or 4 vertices.
and satisfy certain conditions prescribed in terms of graphs having 2, 3 or 4 vertices.
R.M. Santilli in 1978 obtained LA algebras (brackets) from a modified form of Hamilton's equations with external terms which represent a general non-self-adjoint Newtonian system in classical mechanics [a8]. Such a form leads to a time evolution
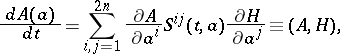 | (a3) |
where 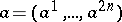 is a local chart in a manifold of dimension
is a local chart in a manifold of dimension 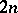 ,
, 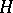 is a Hamiltonian, and
is a Hamiltonian, and 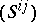 a non-singular
a non-singular 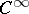 -tensor in a region with decomposition
-tensor in a region with decomposition 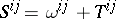 for
for 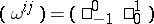 and
and 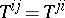 (
( ). The symmetric tensor
). The symmetric tensor 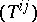 represents the presence of non-self-adjoint forces in the system. The commutator
represents the presence of non-self-adjoint forces in the system. The commutator 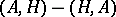 is given by
is given by 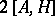 in terms of the classical Poisson brackets
in terms of the classical Poisson brackets 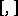 and thus, by (a3),
and thus, by (a3), 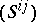 defines a Lie-admissible product
defines a Lie-admissible product 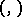 on the
on the 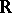 -space of
-space of 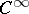 -functions in
-functions in 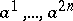 , where
, where 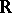 denotes the field of real numbers. The bracket
denotes the field of real numbers. The bracket 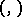 , or
, or 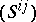 , is called a fundamental Lie-admissible bracket, or tensor. More generally, if
, is called a fundamental Lie-admissible bracket, or tensor. More generally, if  for a skew-symmetric non-singular
for a skew-symmetric non-singular 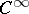 -tensor
-tensor 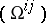 in a region, Lie-admissibility of
in a region, Lie-admissibility of 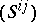 or the bracket (a3) is described by partial differential equations of first order in
or the bracket (a3) is described by partial differential equations of first order in 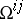 . The general solution
. The general solution 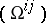 , called the general cosymplectic tensor, to these equations exists under certain conditions and plays a central role in Birkhoffian mechanics (a generalization of Hamiltonian mechanics) [a9]. In this case
, called the general cosymplectic tensor, to these equations exists under certain conditions and plays a central role in Birkhoffian mechanics (a generalization of Hamiltonian mechanics) [a9]. In this case 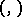 , or
, or 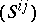 , is called a general Lie-admissible bracket, or tensor. A quantum mechanical version of this leads to a time-development equation
, is called a general Lie-admissible bracket, or tensor. A quantum mechanical version of this leads to a time-development equation 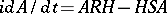 in an associative algebra
in an associative algebra 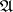 of operators in a physical system, where
of operators in a physical system, where 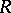 and
and 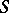 are in general non-Hermitian non-singular operators in
are in general non-Hermitian non-singular operators in 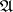 which represent non-self-adjoint forces [a8]. From this equation, regarded as a generalization of the Heisenberg equation, one obtains an LA algebra
which represent non-self-adjoint forces [a8]. From this equation, regarded as a generalization of the Heisenberg equation, one obtains an LA algebra 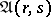 , called the
, called the 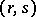 -mutation of
-mutation of 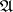 , with product
, with product 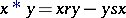 defined on an associative algebra with identity for fixed invertible
defined on an associative algebra with identity for fixed invertible 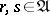 .
. 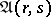 is not in general flexible or power-associative. In fact, any one of these conditions is equivalent to the relation
is not in general flexible or power-associative. In fact, any one of these conditions is equivalent to the relation 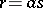 for some invertible
for some invertible  in the centre of
in the centre of 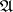 [a10]. A special case of the above approach has been investigated by Santilli in 1967 [a11], where he first introduced LA algebras into physics: For real numbers
[a10]. A special case of the above approach has been investigated by Santilli in 1967 [a11], where he first introduced LA algebras into physics: For real numbers 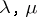 , the bracket (a3) with
, the bracket (a3) with 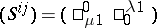 and the algebra
and the algebra 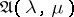 were considered for a generalization of Hamiltonian and quantum mechanics. According to Santilli [a8], the aim of this Lie-admissible approach is to make a transition from contemporary physical models based on Lie algebras or their graded-supersymmetric extensions to the general Lie-admissible models, which transition essentially permits the treatment of particles as being extended and therefore admits additional contact, non-potential and non-Hamiltonian interactions.
were considered for a generalization of Hamiltonian and quantum mechanics. According to Santilli [a8], the aim of this Lie-admissible approach is to make a transition from contemporary physical models based on Lie algebras or their graded-supersymmetric extensions to the general Lie-admissible models, which transition essentially permits the treatment of particles as being extended and therefore admits additional contact, non-potential and non-Hamiltonian interactions.
From a different point of view, S. Okubo [a12] in 1978 used FLA algebras 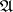 to generalize the framework of the consistent canonical quantization procedure based on the associative law. A quantization is called consistent if the Hamiltonian equation of motion
to generalize the framework of the consistent canonical quantization procedure based on the associative law. A quantization is called consistent if the Hamiltonian equation of motion 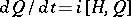 can reproduce the original Lagrange equation. Such a quantization can be done in
can reproduce the original Lagrange equation. Such a quantization can be done in 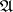 based only on the canonical commutation relation and the identity (a1). If
based only on the canonical commutation relation and the identity (a1). If 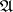 consists of operators in a physical system, then using (a1) it can be shown that the Heisenberg equation
consists of operators in a physical system, then using (a1) it can be shown that the Heisenberg equation 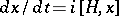 for some
for some 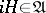 is essentially the most general time-development equation in
is essentially the most general time-development equation in 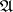 , where
, where 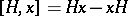 is the commutator in
is the commutator in  . If the Hamiltonian
. If the Hamiltonian 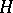 is power-associative in
is power-associative in 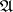 , then the time-development operator
, then the time-development operator  is well defined for the Schrödinger formulation in
is well defined for the Schrödinger formulation in 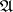 with state vector
with state vector 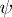 satisfying
satisfying 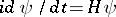 . If, in addition,
. If, in addition, 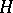 is weakly associative in
is weakly associative in 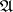 , i.e.
, i.e. 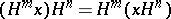 for all positive integers
for all positive integers 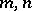 and
and 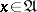 , then the solution to the Heisenberg equation in
, then the solution to the Heisenberg equation in 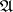 has the form
has the form 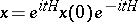 , as in the usual quantum mechanics. An example of such an algebra is the real pseudo-octonion algebra
, as in the usual quantum mechanics. An example of such an algebra is the real pseudo-octonion algebra 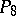 , which has the multiplication
, which has the multiplication 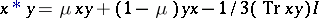 defined on the
defined on the 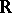 -space of
-space of 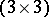 Hermitian matrices of trace 0, where
Hermitian matrices of trace 0, where 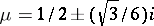 [a2].
[a2]. 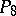 is an FLA division algebra, with
is an FLA division algebra, with  isomorphic to
isomorphic to 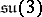 , and has some relevance to
, and has some relevance to 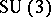 particle physics. It also plays an important role in the structure theory of real division algebras [a2].
particle physics. It also plays an important role in the structure theory of real division algebras [a2].
An algebra 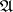 over a field
over a field 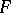 is called Mal'tsev-admissible (MA) if its commutator algebra
is called Mal'tsev-admissible (MA) if its commutator algebra 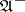 becomes a Mal'tsev algebra, i.e.,
becomes a Mal'tsev algebra, i.e.,  satisfies the Mal'tsev identity
satisfies the Mal'tsev identity
 |
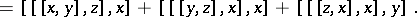 |
It arises as a natural generalization of LA algebras as well as Mal'tsev algebras, and its structure theory is parallel to that of LA algebras [a2]. Alternative algebras (cf. Alternative rings and algebras) are examples of flexible Mal'tsev-admissible (FMA) algebras, and octonion algebras (also called Cayley–Dickson algebras, cf. Cayley–Dickson algebra) are FMA but not LA. For an octonion algebra 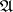 over a field
over a field 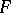 of characteristic
of characteristic 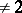 with standard involution
with standard involution  , one obtains an algebra
, one obtains an algebra  with multiplication
with multiplication 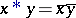 defined on
defined on 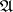 . The algebra
. The algebra 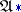 , called a para-octonion algebra, is a simple FMA algebra without identity and so not alternative [a2]. An algebra
, called a para-octonion algebra, is a simple FMA algebra without identity and so not alternative [a2]. An algebra 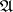 (not necessarily with identity) is called a composition algebra if there exists a non-degenerate quadratic form
(not necessarily with identity) is called a composition algebra if there exists a non-degenerate quadratic form 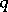 on
on 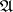 such that
such that 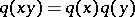 for all
for all 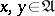 . Any finite-dimensional flexible composition algebra (
. Any finite-dimensional flexible composition algebra (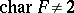 ) is an MA algebra of dimension 1, 2, 4, or 8, and for dimension 8 octonion, pseudo-octonion and para-octonion algebras are the only such algebras [a13]. For an MA algebra
) is an MA algebra of dimension 1, 2, 4, or 8, and for dimension 8 octonion, pseudo-octonion and para-octonion algebras are the only such algebras [a13]. For an MA algebra 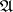 , let
, let 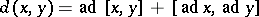 , where
, where  is the adjoint mapping
is the adjoint mapping 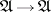 given by
given by  . Then
. Then 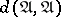 is a Lie subalgebra of the derivation algebra
is a Lie subalgebra of the derivation algebra 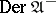 of
of 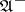 (cf. also Derivation in a ring), and if
(cf. also Derivation in a ring), and if 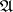 is FMA, then
is FMA, then 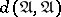 is also a subalgebra of
is also a subalgebra of 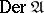 and the mapping
and the mapping 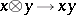 is a Lie module homomorphism of
is a Lie module homomorphism of  to
to 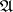 for
for 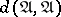 [a2]. Let
[a2]. Let 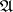 be finite dimensional over a field
be finite dimensional over a field 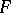 of characteristic 0. If
of characteristic 0. If 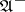 is semi-simple, then so is
is semi-simple, then so is  . Because of this, virtually all results about FLA algebras can be extended to FMA algebras [a2]. If
. Because of this, virtually all results about FLA algebras can be extended to FMA algebras [a2]. If 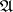 is FMA with
is FMA with 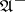 central simple, non-Lie over
central simple, non-Lie over 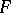 , then
, then 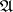 is a Mal'tsev algebra isomorphic to a
is a Mal'tsev algebra isomorphic to a 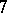 -dimensional simple Mal'tsev algebra obtained from an octonion algebra (cf. Mal'tsev algebra). If
-dimensional simple Mal'tsev algebra obtained from an octonion algebra (cf. Mal'tsev algebra). If 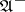 is semi-simple and
is semi-simple and 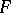 is algebraically closed, then
is algebraically closed, then 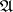 is the direct sum of simple algebras given by (a2) and simple Mal'tsev algebras. Some of the work on MA algebras was motivated by algebraic formalisms in physics aimed at generalizing both the Lie-admissible and the octonionic approach in quantum mechanics.
is the direct sum of simple algebras given by (a2) and simple Mal'tsev algebras. Some of the work on MA algebras was motivated by algebraic formalisms in physics aimed at generalizing both the Lie-admissible and the octonionic approach in quantum mechanics.
LA and MA algebras also arise from differential geometry on Lie groups and reductive homogeneous spaces. For a (connected) Lie group  with Lie algebra
with Lie algebra 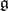 , the determination of all (left) invariant affine connections (cf. Affine connection)
, the determination of all (left) invariant affine connections (cf. Affine connection)  on
on  reduces to the problem of classifying all algebras
reduces to the problem of classifying all algebras 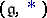 with a multiplication
with a multiplication  defined on
defined on  ; the relation is given by
; the relation is given by 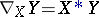 for
for 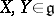 . In this case,
. In this case, 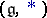 is called the connection algebra of
is called the connection algebra of  . Those connections which are torsion free correspond to the LA algebras
. Those connections which are torsion free correspond to the LA algebras  with
with  (i.e.,
(i.e., 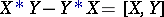 ) [a14]. If, in addition, the curvature tensor of
) [a14]. If, in addition, the curvature tensor of  is zero (i.e.,
is zero (i.e.,  is flat), then
is flat), then 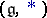 satisfies the left-symmetric identity
satisfies the left-symmetric identity
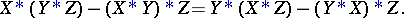 |
The classification of left-invariant affine structures on 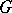 reduces to that of left-symmetric algebras
reduces to that of left-symmetric algebras  with
with 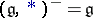 [a15]. Other geometrical properties of
[a15]. Other geometrical properties of  on
on 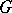 , such as geodesic, holonomy, pseudo-Riemannian structure, and infinitesimal generator, can be described in terms of
, such as geodesic, holonomy, pseudo-Riemannian structure, and infinitesimal generator, can be described in terms of 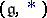 . For example, if every vector field in
. For example, if every vector field in 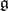 is an infinitesimal generator for a one-parameter group of affine diffeomorphisms on
is an infinitesimal generator for a one-parameter group of affine diffeomorphisms on 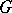 for
for  , then the connection algebra
, then the connection algebra 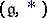 of
of  is FLA [a14].
is FLA [a14].
Let 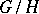 be a reductive homogeneous space with a fixed decomposition
be a reductive homogeneous space with a fixed decomposition 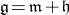 (direct sum), where
(direct sum), where 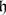 is the Lie algebra of a closed Lie subgroup
is the Lie algebra of a closed Lie subgroup 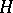 of
of 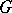 and
and 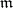 is a subspace of
is a subspace of 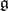 such that
such that 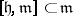 (or, equivalently,
(or, equivalently, 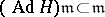 ). There is a one-one correspondence between the set of
). There is a one-one correspondence between the set of 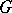 -invariant affine connections
-invariant affine connections  on
on 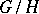 and the set of algebras
and the set of algebras 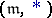 with
with 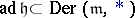 , i.e.,
, i.e.,  , the automorphism group of
, the automorphism group of 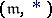 . The projection
. The projection 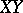 of
of 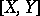 onto
onto  for
for 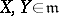 converts
converts 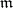 into an anti-commutative algebra
into an anti-commutative algebra 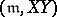 , called a reductive algebra. More generally, an algebra
, called a reductive algebra. More generally, an algebra  is called reductive-admissible if
is called reductive-admissible if 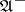 is isomorphic to
is isomorphic to 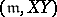 for some reductive decomposition
for some reductive decomposition 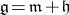 of a Lie algebra
of a Lie algebra 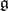 . Those connections on
. Those connections on 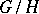 which are torsion free correspond to the reductive-admissible algebras
which are torsion free correspond to the reductive-admissible algebras 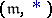 such that
such that 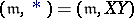 and
and 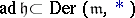 or
or 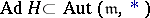 . Any MA algebra
. Any MA algebra  is reductive-admissible with
is reductive-admissible with 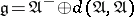 , where
, where 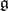 is a Lie algebra with multiplication
is a Lie algebra with multiplication  for
for  and
and 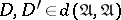 . Geometrical properties of
. Geometrical properties of  on
on 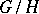 such as those above are described in terms of the connection algebra
such as those above are described in terms of the connection algebra 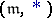 . For a detailed account of these, see [a15]–[a17].
. For a detailed account of these, see [a15]–[a17].
References
| [a1] | A.A. Albert, "Power associative rings" Trans. Amer. Math. Soc. , 64 (1948) pp. 552–593 |
| [a2] | H.C. Myung, "Malcev-admissible algebras" , Birkhäuser (1986) |
| [a3] | P.J. Laufer, M.L. Tomber, "Some Lie admissible algebras" Canad. J. Math. , 14 (1962) pp. 287–292 |
| [a4] | H.C. Myung, "Some classes of flexible Lie-admissible algebras" Trans. Amer. Math. Soc. , 167 (1972) pp. 79–88 |
| [a5] | S. Okubo, H.C. Myung, "Adjoint operators in Lie algebras and the classification of simple flexible Lie-admissible algebras" Trans. Amer. Math. Soc. , 264 (1981) pp. 459–472 |
| [a6] | G.M. Benkart, J.M. Osborn, "Flexible Lie-admissible algebras" J. of Algebra , 71 (1981) pp. 11–31 |
| [a7] | G.M. Benkart, "Power-associative Lie-admissible algebras" J. of Algebra , 90 (1984) pp. 37–58 |
| [a8] | R.M. Santilli, "Lie-admissible approach to the hadronic structure" , II , Hadronic Press (1982) |
| [a9] | R.M. Santilli, "Foundations of theoretical mechanics" , II , Springer (1982) |
| [a10] | J.M. Osborn, "The Lie-admissible mutation 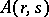 of an associative algebra of an associative algebra 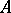 " Hadronic J. , 5 (1982) pp. 904–930 " Hadronic J. , 5 (1982) pp. 904–930 |
| [a11] | R.M. Santilli, "Imbedding of Lie algebras in nonassociative structures" Nuovo Cimento A (10) , 51 (1967) pp. 570–576 |
| [a12] | S. Okubo, "Non-associative quantum mechanics via flexible Lie-admissible algebras" R. Casabuoni (ed.) G. Domokos (ed.) S. Koveski-Domokos (ed.) , Proc. 3-rd Workshop Current Problems in High Energy Physics , Johns Hopkins Univ. Press (1979) pp. 103–120 |
| [a13] | S. Okubo, "Classification of flexible composition algebras, I, II" Hadronic J. , 5 (1982) pp. 1564–1612 |
| [a14] | H.C. Myung, A.A. Sagle, "Lie-admissible algebras and affine connections on Lie groups" S.A. Park (ed.) , Proc. Workshops in Pure Math. , 7. Algebraic Structures , Pure Math. Res. Assoc. (1988) pp. 115–148 |
| [a15] | H. Kim, "Complete left-invariant affine structures on nilpotent Lie groups" J. Differential Geom. , 24 (1986) pp. 373–394 |
| [a16] | A.A. Sagle, "Invariant Lagrangian mechanics, connections, and non-associative algebras" Algebras, Groups Geom. , 3 (1986) pp. 199–263 |
| [a17] | H.C. Myung, A.A. Sagle, "On the construction of reductive Lie-admissible algebras" J. Pure Appl. Algebra , 53 (1988) pp. 75–91 |
Lie-admissible algebra. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Lie-admissible_algebra&oldid=14803