Lebesgue constants of multi-dimensional partial Fourier sums
Let 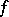 be an integrable function on
be an integrable function on  ,
, 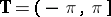 ,
, 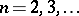 ,
, 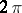 -periodic in each variable. Consider its Fourier series
-periodic in each variable. Consider its Fourier series 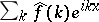 , where
, where  ,
, 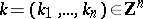 , the lattice of points in
, the lattice of points in 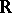 with integer coordinates,
with integer coordinates, 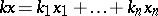 , while
, while
 |
is the 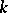 th Fourier coefficient of
th Fourier coefficient of 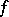 . No natural ordering of Fourier coefficients exists, thus the definition of a multi-dimensional partial Fourier sum presents many problems and points of interest intimately connected to geometry and number theory.
. No natural ordering of Fourier coefficients exists, thus the definition of a multi-dimensional partial Fourier sum presents many problems and points of interest intimately connected to geometry and number theory.
To indicate that the partial sum corresponds to a certain summation domain 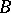 , one denotes it by
, one denotes it by
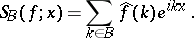 |
Frequently, sums 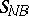 are considered, where
are considered, where 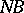 is the
is the 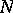 th dilatation of a fixed set
th dilatation of a fixed set 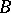 ; in many cases this is the most natural way of summation. An example of partial Fourier sums that are not of this kind are the rectangular partial sums. By
; in many cases this is the most natural way of summation. An example of partial Fourier sums that are not of this kind are the rectangular partial sums. By 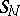 one denotes the partial Fourier sum when the dependence on the parameter
one denotes the partial Fourier sum when the dependence on the parameter 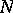 , either scalar or vectorial, is of primary importance. As is well-known, if the Fourier series of a continuous function fails to converge at each point, then the sequence of norms of the operators
, either scalar or vectorial, is of primary importance. As is well-known, if the Fourier series of a continuous function fails to converge at each point, then the sequence of norms of the operators 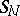 ,
,
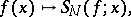 |
taking 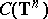 into
into  (or, equivalently,
(or, equivalently, 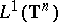 into
into 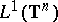 ) is unbounded and measures the rate of divergence of the Fourier series.
) is unbounded and measures the rate of divergence of the Fourier series.
This is strongly related to the behaviour of the Fourier transform of the indicator function of the summation domain 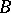 . For known results on this subject, see, e.g., [a11], [a13], [a18].
. For known results on this subject, see, e.g., [a11], [a13], [a18].
For the spherical partial Fourier sums 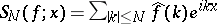 , where
, where 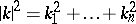 , the following order estimate holds: There exist positive constants
, the following order estimate holds: There exist positive constants 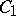 , and
, and  ,
,  , depending only on
, depending only on  , such that
, such that
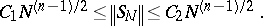 | (a1) |
The estimate from below has first appeared in [a14]; the method used there is the main tool for obtaining lower bounds for Lebesgue constants. Nothing is known about existence or non-existence of the limit of 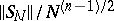 as
as 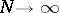 ; this is the main open problem in the subject (as of 2000).
; this is the main open problem in the subject (as of 2000).
More general summation domains 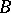 possessing properties of the spherical partial Fourier sums have been considered. E.g., in Yudin's estimate from above [a25], the summation domains
possessing properties of the spherical partial Fourier sums have been considered. E.g., in Yudin's estimate from above [a25], the summation domains 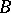 are balanced (i.e., along with each point
are balanced (i.e., along with each point  the whole set
the whole set 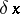 ,
,  , belongs to
, belongs to 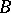 ), with finite upper Minkowski measure, that is,
), with finite upper Minkowski measure, that is,
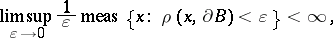 |
where  . These natural assumptions provide the bound
. These natural assumptions provide the bound 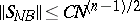 . It turns out that to satisfy the same estimate from below, only local information is needed ([a17]): Let the boundary of a domain
. It turns out that to satisfy the same estimate from below, only local information is needed ([a17]): Let the boundary of a domain 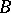 contain a simple (non-intersecting) piece of a surface of smoothness
contain a simple (non-intersecting) piece of a surface of smoothness  in which there is at least one point with non-vanishing principal curvatures (cf. also Principal curvature). Then there exists a positive constant
in which there is at least one point with non-vanishing principal curvatures (cf. also Principal curvature). Then there exists a positive constant  , depending only on
, depending only on 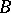 , such that
, such that 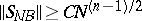 for
for 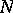 large.
large.
The estimates in the spherical case and its generalizations are the worst possible if 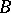 is compact. Once
is compact. Once 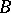 has a point with non-vanishing principal curvatures, the Lebesgue constants are that "bad" . The other side of the scale is called "polyhedral" and is of "logarithmic nature" . Only some natural restrictions have to be put on polyhedra
has a point with non-vanishing principal curvatures, the Lebesgue constants are that "bad" . The other side of the scale is called "polyhedral" and is of "logarithmic nature" . Only some natural restrictions have to be put on polyhedra 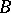 , for example, the hyperplanes that define the sides of the polyhedron do not contain the origin. In that case there exist two positive constants
, for example, the hyperplanes that define the sides of the polyhedron do not contain the origin. In that case there exist two positive constants 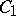 and
and 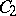 ,
, 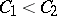 , such that for each such polyhedron
, such that for each such polyhedron 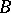 :
:
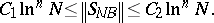 | (a2) |
Actually, this was proved by E. Belinsky [a6].
There are two important problems concerning the polyhedral case. The first is: Can partial Fourier sums have Lebesgue constants with an intermediate rate of growth (i.e. between (a1) and (a2))? Some trivial solutions were suggested in [a26], where an intermediate growth is achieved by the product of the two mentioned situations. Of course, this is possible only for dimension three and greater. Thus, the problem is to find one for dimension two. It is clear that in this case the boundary can possess no point with non-vanishing curvature. On the other hand, any polyhedron matches (a2). Thus, the solution can only be a (convex) "polyhedron" with infinitely many specially located sides. Such a solution was constructed by A. Podkorytov [a21].
The next question also seems very natural: Is it possible to have a certain asymptotic relation instead of the order estimate (a2)? For rectangular partial sums some special cases were investigated by I. Daugavet [a9] and O. Kuznetsova [a15]. For the sequence of dilated summation domains, an unexpected result was obtained by Podkorytov [a22]. Here 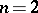 also causes the main difficulties. There are two main cases. In the first one, the polygons
also causes the main difficulties. There are two main cases. In the first one, the polygons 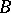 with sides of rational slopes are dealt with — then the estimates change insignificantly if only one considers instead of sums the corresponding integrals, that is, the Fourier transform
with sides of rational slopes are dealt with — then the estimates change insignificantly if only one considers instead of sums the corresponding integrals, that is, the Fourier transform 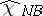 of the indicator function of the
of the indicator function of the 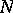 -dilation of the corresponding set
-dilation of the corresponding set  . This allows one to obtain logarithmic asymptotics; namely, the values
. This allows one to obtain logarithmic asymptotics; namely, the values 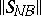 ,
,  and
and 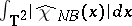 are equivalent. When at least one of the slopes is irrational, the situation changes qualitatively: The upper limit and the lower limit of the ratio
are equivalent. When at least one of the slopes is irrational, the situation changes qualitatively: The upper limit and the lower limit of the ratio 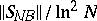 , as
, as 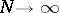 , may differ. In [a22] and [a20], quantitative estimates of this phenomenon as well as open problems are given.
, may differ. In [a22] and [a20], quantitative estimates of this phenomenon as well as open problems are given.
The paper [a4] started the interest in various questions of approximation theory and Fourier analysis in 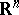 connected with the study of hyperbolic cross partial Fourier sums (see, e.g., [a24] and Hyperbolic cross). The exact order of growth of their Lebesgue constants,
connected with the study of hyperbolic cross partial Fourier sums (see, e.g., [a24] and Hyperbolic cross). The exact order of growth of their Lebesgue constants, 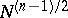 , the same as in the spherical case, was established in the two-dimensional case independently in [a5] and in [a27], and afterwards was generalized to the case of arbitrary dimension in [a16].
, the same as in the spherical case, was established in the two-dimensional case independently in [a5] and in [a27], and afterwards was generalized to the case of arbitrary dimension in [a16].
Step hyperbolic crosses  were introduced by B. Mityagin [a19] and are defined as follows (cf. also Step hyperbolic cross):
were introduced by B. Mityagin [a19] and are defined as follows (cf. also Step hyperbolic cross): 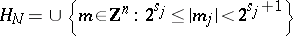 for
for  such that
such that 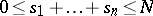 . These have many important applications too. Belinsky [a8] proved that there exist two positive constants
. These have many important applications too. Belinsky [a8] proved that there exist two positive constants 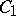 and
and 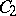 ,
, 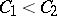 , such that
, such that  .
.
When  is unbounded, it may happen that the operator
is unbounded, it may happen that the operator 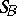 is unbounded even for fixed
is unbounded even for fixed  . It is proved in [a3] that the Lebesgue constants
. It is proved in [a3] that the Lebesgue constants 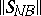 are either of the usual order of growth or infinite for all values of the parameter
are either of the usual order of growth or infinite for all values of the parameter 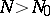 , where
, where 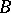 is a hyperbolic cross, depending on whether the hyperbolic cross is turned at a rational or irrational angle, respectively. For
is a hyperbolic cross, depending on whether the hyperbolic cross is turned at a rational or irrational angle, respectively. For 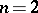 , this was earlier obtained in [a7], which also contains similar results for the strip to be a summation domain.
, this was earlier obtained in [a7], which also contains similar results for the strip to be a summation domain.
For other results on Lebesgue constants and related topics, see [a1], [a2], [a10], [a12], [a18], [a23], [a28], [a29].
The ideas used to prove many of the results discussed above have also been applied to estimates of the Lebesgue constants of linear means of multiple Fourier series.
Some results are known for Lebesgue constants in more abstract settings, e.g., for spherical harmonics expansions or Fourier series on compact Lie groups.
References
| [a1] | Sh.A. Alimov, R.R. Ashurov, A.K. Pulatov, "Multiple Fourier series and Fourier integrals" V.P. Khavin (ed.) N.K. Nikolskii (ed.) , Commutative Harmonic Analysis IV , Enc. Math. Sci. , 42 , Springer (1992) pp. 1–95 Itogi Nauki i Tekhn. VINITI Akad. Nauk. SSSR , 42 (1989) pp. 7–104 |
| [a2] | Sh.A. Alimov, V.A. Ilyin, E.M. Nikishin, "Convergence problems of multiple Fourier series and spectral decompositions, I, II" Russian Math. Surveys , 31/32 (1976/77) pp. 29–86; 115–139 Uspekhi Mat. Nauk. , 31/32 (1976/77) pp. 28–83; 107–130 |
| [a3] | E.S. Belinskii, E.R. Liflyand, "Behavior of the Lebesgue constants of hyperbolic partial sums" Math. Notes , 43 (1988) pp. 107–109 Mat. Zametki , 43 (1988) pp. 192–196 |
| [a4] | K.I. Babenko, "Approximation by trigonometric polynomials in a certain class of periodic functions of several variables" Soviet Math. Dokl. , 1 (1960) pp. 672–675 Dokl. Akad. Nauk. SSSR , 132 (1960) pp. 982–985 |
| [a5] | E.S. Belinsky, "Behavior of the Lebesgue constants of certain methods of summation of multiple Fourier series" , Metric Questions of the Theory of Functions and Mappings , Nauk. Dumka, Kiev (1977) pp. 19–39 (In Russian) |
| [a6] | E.S. Belinsky, "Some properties of hyperbolic partial sums" , Theory of Functions and Mappings , Nauk. Dumka, Kiev (1979) pp. 28–36 (In Russian) |
| [a7] | E.S. Belinsky, "On the growth of Lebesgue constants of partial sums generated by certain unbounded sets" , Theory of Mappings and Approximation of Functions , Nauk. Dumka, Kiev (1983) pp. 18–20 (In Russian) |
| [a8] | E.S. Belinsky, "Lebesgue constants of step hyperbolic partial sums" , Theory of Mappings and Approximation of Functions , Nauk. Dumka, Kiev (1989) pp. 23–27 (In Russian) |
| [a9] | I.K. Daugavet, "On the Lebesgue constants for double Fourier series" Meth. Comput., Leningrad Univ. , 6 (1970) pp. 8–13 (In Russian) |
| [a10] | M. Dyachenko, "Some problems in the theory of multiple trigonometric series" Russian Math. Surveys , 47 : 5 (1992) pp. 103–171 Uspekhi Mat. Nauk. , 47 : 5 (1992) pp. 97–162 |
| [a11] | I.M. Gelfand, M.I. Graev, N.Ya. Vilenkin, "Generalized functions 5: Integral geometry and problems of representation theory" , Acad. Press (1966) |
| [a12] | B.I. Golubov, "Multiple Fourier series and integrals" J. Soviet Math. , 24 (1984) pp. 639–673 Itogi Nauki i Tekhn. VINITI Akad. Nauk. SSSR , 19 (1982) pp. 3–54 |
| [a13] | C.S. Herz, "Fourier transforms related to convex sets" Ann. of Math. , 2 : 75 (1962) pp. 81–92 |
| [a14] | V.A. Ilyin, "Problems of localization and convergence for Fourier series in fundamental systems of the Laplace operator" Russian Math. Surveys , 23 (1968) pp. 59–116 Uspekhi Mat. Nauk. , 23 (1968) pp. 61–120 |
| [a15] | O.I. Kuznetsova, "The asymptotic behavior of the Lebesgue constants for a sequence of triangular partial sums of double fourier series" Sib. Math. J. , 18 (1977) pp. 449–454 Sibirsk. Mat. Zh. , XVIII (1977) pp. 629–636 |
| [a16] | E.R. Liflyand, "Exact order of the Lebesgue constants of hyperbolic partial sums of multiple Fourier series" Math. Notes , 39 (1986) pp. 369–374 Mat. Zametki , 39 (1986) pp. 674–683 |
| [a17] | E.R. Liflyand, "Sharp estimates of the Lebesgue constants of partial sums of multiple Fourier series" Proc. Steklov Inst. Math. , 180 (1989) pp. 176–177 Trudy Mat. Inst. V.A. Steklov. , 180 (1987) pp. 151–152 |
| [a18] | E.R. Liflyand, A.G. Ramm, A.I. Zaslavsky, "Estimates from below for Lebesgue constants" J. Fourier Anal. Appl. , 2 (1996) pp. 287–301 |
| [a19] | B.S. Mityagin, "Approximation of functions in 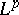 and and 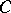 spaces on the torus" Mat. Sb. (N.S.) , 58 (100) (1962) pp. 397–414 (In Russian) spaces on the torus" Mat. Sb. (N.S.) , 58 (100) (1962) pp. 397–414 (In Russian) |
| [a20] | F. Nazarov, A. Podkorytov, "On the behavior of the Lebesgue constants for two-dimensional Fourier sums over polygons" St.-Petersburg Math. J. , 7 (1995) pp. 663–680 Algebra i Anal. , 7 (1995) pp. 214–238 |
| [a21] | A.N. Podkorytov, "Intermediate rates of growth of Lebesgue constants in the two–dimensional case" J. Soviet Math. , 36 (1987) pp. 276–282 Numerical Methods and Questions on the Organization of Calculations, Part 7 Notes Sci. Sem. Steklov Inst. Math. Leningrad. Branch Acad. Sci. USSR, Nauka, Leningrad , 139 (1984) pp. 148–155 |
| [a22] | A.N. Podkorytov, "Asymptotic behavior of the Dirichlet kernel of Fourier sums with respect to a polygon" J. Soviet Math. , 42 (1988) pp. 1640–1646 Zap. Nauchn. Sem. LOMI , 149 (1986) pp. 142–149 |
| [a23] | E.M. Stein, G. Weiss, "Introduction to Fourier analysis on Euclidean spaces" , Princeton Univ. Press (1971) |
| [a24] | V.N. Temlyakov, "Approximation of periodic functions" , Nova Sci. (1993) |
| [a25] | V.A. Yudin, "Behavior of Lebesgue constants" Math. Notes , 17 (1975) pp. 369–374 Mat. Zametki , 17 (1975) pp. 401–405 |
| [a26] | V.A. Yudin, "A lower bound for Lebesgue constants" Math. Notes , 25 (1979) pp. 63–65 Mat. Zametki , 25 (1979) pp. 119–122 |
| [a27] | A.A. Yudin, V.A. Yudin, "Discrete imbedding theorems and Lebesgue constants" Math. Notes , 22 (1977) pp. 702–711 Mat. Zametki , 22 (1977) pp. 381–394 |
| [a28] | L.V. Zhizhiashvili, "Some problems in the theory of simple and multiple trigonometric and orthogonal series" Russian Math. Surveys , 28 (1973) pp. 65–127 Uspekhi Mat. Nauk. , 28 (1973) pp. 65–119 |
| [a29] | L.V. Zhizhiashvili, "Some problems of multidimensional harmonic analysis" , Tbilisi State Univ. (1996) (In Russian) (Edition: Second) |
Lebesgue constants of multi-dimensional partial Fourier sums. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Lebesgue_constants_of_multi-dimensional_partial_Fourier_sums&oldid=14045