Kronecker theorem
Given  ,
,  , and
, and  ; then for any
; then for any  there exist integers
there exist integers  ,
,  , and
, and  ,
,  , such that
, such that
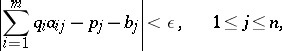 |
if and only if for any  such that
such that
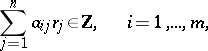 |
the number
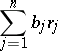 |
is also an integer. This theorem was first proved in 1884 by L. Kronecker (see [1]).
Kronecker's theorem is a special case of the following theorem [2], which describes the closure of the subgroup of the torus  generated by the elements
generated by the elements 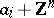 ,
,  : The closure is precisely the set of all classes
: The closure is precisely the set of all classes 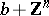 such that, for any numbers
such that, for any numbers 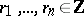 with
with
 |
one has also
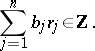 |
(Cf. [2].) Under the assumptions of Kronecker's theorem, this closure is simply  . This means that the subgroup of all elements of the form
. This means that the subgroup of all elements of the form
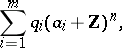 |
where  , is dense in
, is dense in  , while the subgroup of vectors
, while the subgroup of vectors
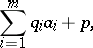 |
where  , is dense in
, is dense in 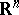 . Kronecker's theorem can be derived from the duality theory for commutative topological groups (cf. Topological group), [3].
. Kronecker's theorem can be derived from the duality theory for commutative topological groups (cf. Topological group), [3].
In the case 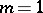 , Kronecker's theorem becomes the following proposition: A class
, Kronecker's theorem becomes the following proposition: A class  , where
, where  , generates
, generates  as a topological group if and only if the numbers
as a topological group if and only if the numbers  are linearly independent over the field
are linearly independent over the field 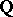 of rational numbers. In particular, the torus
of rational numbers. In particular, the torus  as a topological group is monothetic, i.e. is generated by a single element.
as a topological group is monothetic, i.e. is generated by a single element.
References
| [1] | L. Kronecker, "Näherungsweise ganzzahlige Auflösung linearer Gleichungen" , Werke , 3 , Chelsea, reprint (1968) pp. 47–109 |
| [2] | N. Bourbaki, "Elements of mathematics. General topology" , Addison-Wesley (1966) (Translated from French) |
| [3] | L.S. Pontryagin, "Topological groups" , Princeton Univ. Press (1958) (Translated from Russian) |
Comments
The last statement above can be rephrased as: If  are linearly independent over
are linearly independent over 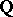 , then the set
, then the set  is dense in
is dense in  . Here
. Here  denotes the fractional part of
denotes the fractional part of  (cf. Fractional part of a number). In fact, the set
(cf. Fractional part of a number). In fact, the set  is even uniformly distributed, cf. Uniform distribution.
is even uniformly distributed, cf. Uniform distribution.
References
| [a1] | G.H. Hardy, E.M. Wright, "An introduction to the theory of numbers" , Oxford Univ. Press (1979) pp. Chapt. 23 |
| [a2] | J.W.S. Cassels, "An introduction to diophantine approximation" , Cambridge Univ. Press (1957) |
Kronecker theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Kronecker_theorem&oldid=19181