KP-equation
Kadomtsev–Petviashvili equation
The equation
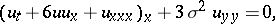 |
with  . It arose in applied mathematics [a4] but was soon recognized to be related to problems of algebraic geometry and representation theory, besides spectral theory.
. It arose in applied mathematics [a4] but was soon recognized to be related to problems of algebraic geometry and representation theory, besides spectral theory.
The general (formal) solution was found by M. Sato, cf. [a10], and is given as:
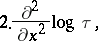 |
by the tau-function, the section of a determinant line bundle over an infinite-dimensional Grassmannian; Sato proved that the KP-equation is equivalent to the Plücker relations for this Grassmannian.
The algebro-geometric solution was expressed by I.M. Krichever [a5] in terms of theta-functions on the Jacobi variety of a complex curve (cf. also Complex manifold) and T. Shiota [a11] settled the Novikov conjecture by proving that, conversely, if a theta-function satisfies the KP-equation, then its period matrix comes from a Riemann surface (cf. Schottky problem).
The spectral theory, an inverse scattering in two space and one time dimensions, was attacked most effectively by R. Beals and R.R. Coifman by designing the Neumann  -problem, cf. [a2].
-problem, cf. [a2].
All these methods treat also the KP-hierarchy, of which the KP-equation is the first non-trivial member. This hierarchy can be formulated for functions of infinitely many variables  as the identity for the coefficients of
as the identity for the coefficients of
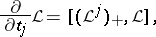 |
where  is a (formal) pseudo-differential operator, the variables of the KP-equation are taken to be
is a (formal) pseudo-differential operator, the variables of the KP-equation are taken to be  ,
, 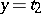 ,
,  ,
,  is abbreviated as
is abbreviated as  and
and  denotes deletion of the terms involving negative powers of
denotes deletion of the terms involving negative powers of 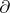 . From this point of view, the curve associated to the algebro-geometric solutions is the spectral curve of the ring of differential operators in
. From this point of view, the curve associated to the algebro-geometric solutions is the spectral curve of the ring of differential operators in  that commute with
that commute with  and the times
and the times  are the isospectral deformations of the problem, which are linear flows on the Jacobi variety, a complex torus
are the isospectral deformations of the problem, which are linear flows on the Jacobi variety, a complex torus 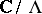 ; this theory was developed by J.L. Burchnall and T.W. Chaundy in the 1920s, cf. [a7].
; this theory was developed by J.L. Burchnall and T.W. Chaundy in the 1920s, cf. [a7].
The celebrated Korteweg–de Vries equation (respectively, the Boussinesq equation, see also Turbulence, mathematical problems in; Soliton; Oberbeck–Boussinesq equations) is but a reduction of the KP-equation, in the sense that the solution 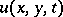 is independent of
is independent of  (respectively,
(respectively,  ), as are other soliton equations ( cf. also Soliton), obtained by possibly modifying the group whose representation theory yields the tau-function (
), as are other soliton equations ( cf. also Soliton), obtained by possibly modifying the group whose representation theory yields the tau-function ( in the KP-case), cf. [a3].
in the KP-case), cf. [a3].
Numerous issues related to the KP-equation are still under active investigation:
1) reality conditions (cf. [a9]), for the aforementioned solutions are given over the complex numbers;
2) solutions that belong to special classes of functions (cf. [a1]), such as rational, solitonic, elliptic, bispectral;
3) construction of solutions from vector bundles of rank  over a curve (cf. [a6]), which generalize the Jacobian case where
over a curve (cf. [a6]), which generalize the Jacobian case where 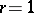 ;
;
4) construction of analogous hierarchies for commuting matrix differential operators (cf. [a8]), whose spectral variety has dimension greater than  ; and
; and
5) connections with matrix models and quantum field theory (cf. [a12]).
References
| [a1] | M.J. Ablowitz, P.A. Clarkson, "Solitons, nonlinear evolution equations and inverse scattering" , Cambridge Univ. Press (1991) |
| [a2] | R. Beals, P. Deift, C. Tomei, "Direct and inverse scattering on the line" , Amer. Math. Soc. (1988) |
| [a3] | E. Date, M. Kashiwara, M. Jimbo, T. Miwa, "Transformation groups for soliton equation" , Nonlinear Integrable Systems - Classical Theory and Quantum Theory Proc. RIMS Symp., Kyoto 1981 (1983) pp. 39–119 |
| [a4] | B.B. Kadomtsev, V.J. Petviashvili, "On the stability of solitary waves in weakly dispersive media" Soviet Phys. Dokl. , 15 (1970) pp. 539–541 |
| [a5] | I.M. Krichever, "Methods of algebraic geometry in the theory of non-linear equations" Russian Math. Surveys , 32 : 6 (1977) pp. 185–213 |
| [a6] | I.M. Krichever, S.P. Novikov, "Holomorphic bundles over algebraic curves and nonlinear equations" Russian Math. Surveys , 35 : 6 (1980) pp. 53–79 Uspekhi Mat. Nauk , 35 : 6 (216) (1980) pp. 47–68 |
| [a7] | M. Mulase, "Algebraic theory of the KP equations" R. Penner (ed.) et al. (ed.) , Perspectives in Mathematical Physics. Proc. Conf. Interface Math. And Physics, Taiwan summer 1992 , Conf. Proc. Math. Phys. , 3 , Internat. Press (1994) pp. 151–217 (Also: Special Session On Topics In Geometry And Physics, Los Angeles, Winter 1992) |
| [a8] | A. Nakayashiki, "Structure of Baker–Akhiezer modules of principally polarized abelian varieties, commuting partial differential operators and associated integrable systems" Duke Math. J. , 62 : 2 (1991) pp. 315–358 |
| [a9] | S.M. Natanzon, "Real nonsingular finite zone solutions of soliton equations" S.P. Novikov (ed.) , Topics in Topol. Math. Physics , Transl. Ser. 2 , 170 , Amer. Math. Soc. (1995) pp. 153–183 |
| [a10] | M. Sato, "The KP hierarchy and infinite-dimensional Grassmann manifolds" , Theta functions. Proc. 35th Summer Res. Inst. Bowdoin Coll., Brunswick/ME 1987 , Proc. Symp. Pure Math. , 49:1 , Amer. Math. Soc. (1989) pp. 51–66 |
| [a11] | T. Shiota, "Characterization of Jacobian varieties in terms of soliton equations" Invent. Math. , 83 (1986) pp. 333–382 |
| [a12] | E. Witten, "Two-dimensional gravity and intersection theory on moduli space" J. Diff. Geom. , Suppl. 1 (1991) pp. 243–310 |
KP-equation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=KP-equation&oldid=11256