Kähler metric
Kählerian metric
A Hermitian metric on a complex manifold whose fundamental form 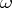 is closed, i.e. satisfies the condition
is closed, i.e. satisfies the condition 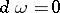 . Examples: the Hermitian metric
. Examples: the Hermitian metric  in
in  ; the Fubini–Study metric on the complex projective space
; the Fubini–Study metric on the complex projective space  ; and the Bergman metric (see Bergman kernel function) in a bounded domain in
; and the Bergman metric (see Bergman kernel function) in a bounded domain in  . A Kähler metric on a complex manifold induces a Kähler metric on any submanifold. Any Hermitian metric on a one-dimensional manifold is a Kähler metric.
. A Kähler metric on a complex manifold induces a Kähler metric on any submanifold. Any Hermitian metric on a one-dimensional manifold is a Kähler metric.
The concept was first studied by E. Kähler [1]. At the same time, in algebraic geometry systematic use was made of a metric on projective algebraic varieties induced by the Fubini–Study metric (see [5]). This is a Hodge metric, i.e. its fundamental form has integral periods.
A Hermitian metric 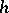 on a complex manifold is a Kähler metric if and only if it satisfies any one of the following conditions: parallel transfer along any curve (relative to the Levi-Civita connection) is a complex linear mapping, i.e. it commutes with the complex structure operator; the complex Laplacian
on a complex manifold is a Kähler metric if and only if it satisfies any one of the following conditions: parallel transfer along any curve (relative to the Levi-Civita connection) is a complex linear mapping, i.e. it commutes with the complex structure operator; the complex Laplacian  corresponding to the metric
corresponding to the metric  on differential forms satisfies the condition
on differential forms satisfies the condition 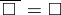 , i.e. the Laplace operator
, i.e. the Laplace operator  is precisely
is precisely 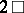 ; local coordinates can be introduced in a neighbourhood of any point, relative to which the matrix of
; local coordinates can be introduced in a neighbourhood of any point, relative to which the matrix of 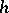 coincides with the identity matrix up to second-order quantities (see [3], [6]).
coincides with the identity matrix up to second-order quantities (see [3], [6]).
References
| [1] | E. Kähler, "Ueber eine bemerkenswerte Hermitesche Metrik" Abh. Math. Sem. Univ. Hamburg , 9 (1933) pp. 173–186 |
| [2] | A. Weil, "Introduction à l'Aeetude des variétés kahlériennes" , Hermann (1958) |
| [3] | A. Lichnerowicz, "Global theory of connections and holonomy groups" , Noordhoff (1976) (Translated from French) |
| [4] | R.O. Wells jr., "Differential analysis on complex manifolds" , Springer (1980) |
| [5] | W.V.D. Hodge, "The theory and application of harmonic integrals" , Cambridge Univ. Press (1952) |
| [6] | P. Deligne, P. Griffiths, J. Morgan, D. Sullivan, "The real homology of Kaehler manifolds" Invent. Math. , 29 (1975) pp. 245–274 |
Comments
On a complex manifold a Hermitian metric  can be expressed in local coordinates by a Hermitian symmetric tensor:
can be expressed in local coordinates by a Hermitian symmetric tensor:
 |
where  is a positive-definite Hermitian (symmetric) matrix (i.e.
is a positive-definite Hermitian (symmetric) matrix (i.e.  and
and  for all
for all 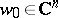 ). The associated fundamental form is then
). The associated fundamental form is then
 |
Kähler metric. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=K%C3%A4hler_metric&oldid=15820