Isometric operator
A mapping  of a metric space
of a metric space 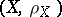 into a metric space
into a metric space  such that
such that
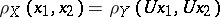 |
for all 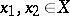 . If
. If  and
and  are real normed linear spaces,
are real normed linear spaces,  and
and 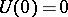 , then
, then 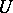 is a linear operator.
is a linear operator.
An isometric operator 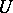 maps
maps  one-to-one onto
one-to-one onto 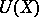 , so that the inverse operator
, so that the inverse operator 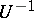 exists, and this is also an isometric operator. The conjugate of a linear isometric operator from some normed linear space into another is also isometric. A linear isometric operator mapping
exists, and this is also an isometric operator. The conjugate of a linear isometric operator from some normed linear space into another is also isometric. A linear isometric operator mapping 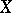 onto the whole of
onto the whole of  is said to be a unitary operator. The condition for a linear operator
is said to be a unitary operator. The condition for a linear operator 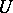 acting on a Hilbert space
acting on a Hilbert space 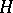 to be unitary is the equation
to be unitary is the equation 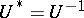 . The spectrum of a unitary operator (cf. Spectrum of an operator) lies on the unit circle, and
. The spectrum of a unitary operator (cf. Spectrum of an operator) lies on the unit circle, and 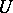 has a representation
has a representation
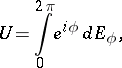 |
where 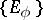 is the corresponding resolution of the identity. An isometric operator defined on a subspace of a Hilbert space and taking values in that space can be extended to a unitary operator if the orthogonal complement of its domain of definition and its range have the same dimension.
is the corresponding resolution of the identity. An isometric operator defined on a subspace of a Hilbert space and taking values in that space can be extended to a unitary operator if the orthogonal complement of its domain of definition and its range have the same dimension.
With every symmetric operator 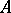 with domain of definition
with domain of definition 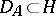 is associated the isometric operator
is associated the isometric operator
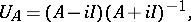 |
called the Cayley transform of 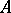 . If
. If 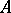 is self-adjoint, then
is self-adjoint, then 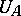 is unitary.
is unitary.
Two operators 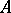 and
and 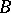 with the same domain of definition
with the same domain of definition 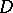 are said to be metrically equal if
are said to be metrically equal if 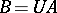 , where
, where 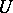 is an isometric operator, that is, if
is an isometric operator, that is, if 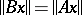 for all
for all  . Such operators have a number of properties in common. For every bounded linear operator
. Such operators have a number of properties in common. For every bounded linear operator 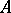 acting on a Hilbert space there exists one and only one positive operator metrically equal to it, namely that defined by the equality
acting on a Hilbert space there exists one and only one positive operator metrically equal to it, namely that defined by the equality 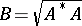 .
.
References
| [1] | N.I. Akhiezer, I.M. Glazman, "Theory of linear operators on a Hilbert space" , 1–2 , Pitman (1981) (Translated from Russian) |
| [2] | A.I. Plesner, "Spectral theory of linear operators" , F. Ungar (1965) (Translated from Russian) |
| [3] | B. Mazur, S. Ulam, "Sur les transformations isométriques d'espaces vectoriels normés" C.R. Acad. Sci. Paris , 194 (1932) pp. 946–948 |
Isometric operator. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Isometric_operator&oldid=16897