Involution
An endomorphism of order two, that is, a mapping of an object onto itself whose square is the identity morphism (see also Category with involution). A periodic mapping, that is, a morphism some non-zero power of which is the identity morphism, is also sometimes called an involution. The minimum of such powers is called the period of the involution.
Often, by the involutions of a group  one means the elements of order two in
one means the elements of order two in  .
.
An involution in an algebra  over the field of real or complex numbers is a mapping
over the field of real or complex numbers is a mapping  of
of  onto itself satisfying the following involution axioms: 1)
onto itself satisfying the following involution axioms: 1)  for all
for all  ; 2)
; 2)  for all
for all  ; 3)
; 3) 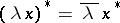 for all
for all 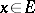 and for all
and for all  in the corresponding field; and 4)
in the corresponding field; and 4) 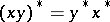 for all
for all 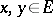 . An algebra
. An algebra  over the complex field endowed with an involution is called a symmetric algebra or involution algebra.
over the complex field endowed with an involution is called a symmetric algebra or involution algebra.
References
| [1] | P.E. Conner, E.E. Floyd, "Differentiable periodic maps" , Springer (1964) |
An involution in projective geometry is a projective transformation whose square is the identity transformation. A non-identity involution of the real projective line has just two fixed points (a hyperbolic involution) or has no fixed points (an elliptic involution). If  and
and  are the fixed points of a hyperbolic involution, then the points
are the fixed points of a hyperbolic involution, then the points 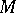 and
and  corresponding to them harmonically divide the pair
corresponding to them harmonically divide the pair 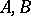 . Every involution on the projective plane is a hyperbolic homology.
. Every involution on the projective plane is a hyperbolic homology.
References
| [1] | N.V. Efimov, "Höhere Geometrie" , Deutsch. Verlag Wissenschaft. (1960) (Translated from Russian) |
An involution of an algebraic variety is an automorphism of the variety of order two. If 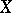 is a non-singular projective algebraic variety over an algebraically closed field
is a non-singular projective algebraic variety over an algebraically closed field 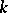 and
and 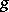 is an involution of
is an involution of  , then the quotient variety
, then the quotient variety  with respect to the action of the cyclic group
with respect to the action of the cyclic group 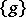 is a projective variety, called the quotient under the involution
is a projective variety, called the quotient under the involution 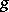 . The set of fixed points
. The set of fixed points  of
of 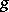 forms a non-singular subvariety of
forms a non-singular subvariety of  . If
. If 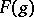 has codimension 1 at each point, the image of
has codimension 1 at each point, the image of  is a non-singular variety. The numerical invariants of a non-singular model
is a non-singular variety. The numerical invariants of a non-singular model  of the variety
of the variety  can be calculated by means of the Lefschetz formula.
can be calculated by means of the Lefschetz formula.
References
| [1] | M.F. Atiyah, I.M. Singer, "The index of elliptic operators III" Ann. of Math. (2) , 87 : 1 (1968) pp. 546–604 |
| [2] | I.V. Dolgachev, V.A. Iskovskikh, "Geometry of algebraic varieties" J. Soviet Math. , 5 : 6 (1976) pp. 803–864 Itogi Nauk. Algebra Topol. Geom. , 12 (1975) pp. 77–170 |
| [3] | L. Godeaux, "Les involutions cycliques appartenant à une surface algébrique" , Hermann (1963) |
Involution. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Involution&oldid=47428