Invariant set
of the phase space 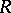 of a dynamical system
of a dynamical system 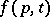
A set 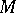 which is the union of entire trajectories, that is, a set satisfying the condition
which is the union of entire trajectories, that is, a set satisfying the condition
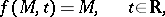 |
where 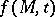 is the image of
is the image of 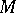 under the transformation
under the transformation 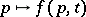 corresponding to a given
corresponding to a given  .
.
The invariant set 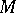 may possess a definite topological structure as a set of the metric space
may possess a definite topological structure as a set of the metric space 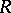 ; for example, it can be a topological or smooth manifold, a surface, a closed Jordan curve, or an isolated point. One then says that the invariant set
; for example, it can be a topological or smooth manifold, a surface, a closed Jordan curve, or an isolated point. One then says that the invariant set 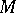 is an invariant manifold, an invariant surface, an invariant curve, or an invariant point.
is an invariant manifold, an invariant surface, an invariant curve, or an invariant point.
An invariant point is usually called a stationary point of the dynamical system, since for this point the motion reduces to rest: 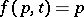 for all values of
for all values of  . A closed invariant curve not containing any invariant points of the dynamical system is always formed by the trajectory of a periodic motion, that is, a motion satisfying the condition
. A closed invariant curve not containing any invariant points of the dynamical system is always formed by the trajectory of a periodic motion, that is, a motion satisfying the condition
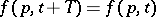 |
for all 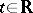 and some
and some 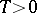 . For this reason it is called a periodic trajectory. Examples which occur as invariant manifolds are a sphere, a torus and a disc; invariant surfaces — a cone, a Möbius strip and a sphere with handles; invariant sets — the set of all stationary points, the sets
. For this reason it is called a periodic trajectory. Examples which occur as invariant manifolds are a sphere, a torus and a disc; invariant surfaces — a cone, a Möbius strip and a sphere with handles; invariant sets — the set of all stationary points, the sets 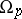 and
and 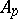 of all
of all 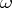 - and
- and 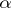 -limit points of the motion
-limit points of the motion 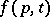 , respectively, and also the set
, respectively, and also the set 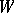 of all wandering points or the set
of all wandering points or the set 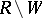 of all non-wandering points (cf. Wandering point; Non-wandering point).
of all non-wandering points (cf. Wandering point; Non-wandering point).
An invariant point of the dynamical system in the plane
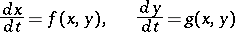 | (1) |
belongs to one of the following four types, according to the nature of the behaviour of the trajectories in a neighbourhood of it: a node, (Fig.a) a focus, (Fig.b) a saddle, (Fig.c) or a centre (Fig.d).
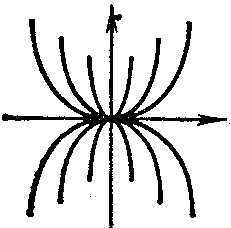
Figure: i052280a
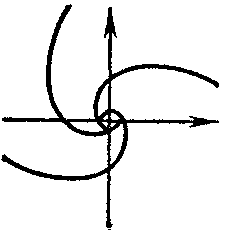
Figure: i052280b

Figure: i052280c
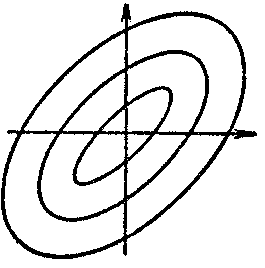
Figure: i052280d
The node and focus are asymptotically stable or unstable, the saddle is unstable, and the centre is stable (cf. Asymptotically-stable solution). The Poincaré index of the node, centre and focus is equal to 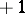 ; that of the saddle is
; that of the saddle is 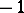 .
.
In the case when the Jacobi matrix
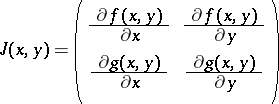 |
of the right-hand side of (1) has at the stationary point  eigen values
eigen values 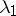 ,
,  with non-zero real parts, the invariant point is: a node if
with non-zero real parts, the invariant point is: a node if  and
and  are real and of the same sign; a saddle if
are real and of the same sign; a saddle if 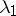 and
and 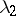 are real with different signs; or a focus if
are real with different signs; or a focus if 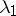 and
and 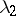 are complex conjugates.
are complex conjugates.
In all these cases, the type of the singular point of the system (1) is the same as for the linear system obtained from (1) by expanding its right-hand side in a Taylor series at the point 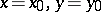 , that is, it has the same type as the point
, that is, it has the same type as the point 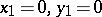 of the system
of the system
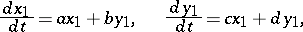 | (2) |
whose matrix 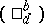 is equal to
is equal to 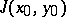 . There is a deeper relation between the trajectories of (1) in a neighbourhood of a singular point of one of the above types and the trajectories of (2) than that already mentioned. Namely, whenever the functions
. There is a deeper relation between the trajectories of (1) in a neighbourhood of a singular point of one of the above types and the trajectories of (2) than that already mentioned. Namely, whenever the functions 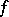 and
and 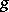 are analytic in a neighbourhood of the invariant point
are analytic in a neighbourhood of the invariant point  and the matrix
and the matrix 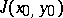 has eigen values with non-zero real parts, there exists a continuously-differentiable change of variables in a neighbourhood
has eigen values with non-zero real parts, there exists a continuously-differentiable change of variables in a neighbourhood 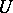 of the point
of the point 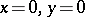 ,
,
 |
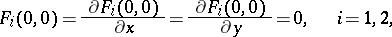 |
that reduces the system (1) to the system (2).
If 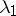 ,
, 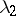 are imaginary, then the invariant point
are imaginary, then the invariant point  can either be a focus or a centre. In this case, the question of the classification of the type of the singular point is a separate and difficult problem, namely the centre and focus problem, and requires more refined criteria for distinguishing between the centre and the focus (see [1], [7]). Similar difficulties occur in the determination of the type of the singular point in the case when the matrix
can either be a focus or a centre. In this case, the question of the classification of the type of the singular point is a separate and difficult problem, namely the centre and focus problem, and requires more refined criteria for distinguishing between the centre and the focus (see [1], [7]). Similar difficulties occur in the determination of the type of the singular point in the case when the matrix 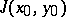 is singular.
is singular.
References
| [1] | V.V. Nemytskii, V.V. Stepanov, "Qualitative theory of differential equations" , Princeton Univ. Press (1960) (Translated from Russian) |
| [2] | G.D. Birkhoff, "Dynamical systems" , Amer. Math. Soc. (1927) |
| [3] | W.W. [V.V. Stepanov] Stepanow, "Lehrbuch der Differentialgleichungen" , Deutsch. Verlag Wissenschaft. (1956) (Translated from Russian) |
| [4] | P. Hartman, "Ordinary differential equations" , Birkhäuser (1982) |
| [5] | I.G. Malkin, "Theorie der Stabilität einer Bewegung" , R. Oldenbourg , München (1959) (Translated from Russian) |
| [6] | A.M. Lyapunov, "Collected works" , 2 , Moscow-Leningrad (1956) (In Russian) |
| [7] | K.S. Sibirskii, "Algebraic invariance of differential equations and matrices" , Kishinev (1976) (In Russian) |
Comments
The reduction of the system (1) to the system (2) in a neighbourhood of a singular point is often called linearization of the equation. If 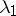 and
and 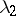 have non-zero real parts (in that case the singular point
have non-zero real parts (in that case the singular point 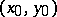 is said to be of hyperbolic type) such a linearization can always be performed by means of a topological (non-smooth) coordinate transformation (i.e., the transformation and its converse are merely continuous), provided
is said to be of hyperbolic type) such a linearization can always be performed by means of a topological (non-smooth) coordinate transformation (i.e., the transformation and its converse are merely continuous), provided 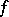 and
and 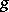 in (1) are of class
in (1) are of class 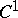 . This result holds also for autonomous differential equations in
. This result holds also for autonomous differential equations in 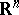 for
for 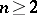 . If certain "non-resonance" conditions between the eigen values are fulfilled, then one can also obtain smooth (under additional conditions even
. If certain "non-resonance" conditions between the eigen values are fulfilled, then one can also obtain smooth (under additional conditions even 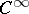 or analytic) transformations (work of H. Poincaré, C.L. Siegel, S. Sternberg). See [4], pp. 256-271 and the references given in [4] on pp. 271-272. These results are related to the theory of normal forms for differential equations (cf. Normal form) and involve problems on small denominators.
or analytic) transformations (work of H. Poincaré, C.L. Siegel, S. Sternberg). See [4], pp. 256-271 and the references given in [4] on pp. 271-272. These results are related to the theory of normal forms for differential equations (cf. Normal form) and involve problems on small denominators.
For a description of the Poincaré index see Singular point.
Invariant set. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Invariant_set&oldid=13413