Integral operator
A mapping 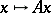 in which the law of the correspondence
in which the law of the correspondence 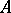 is given by an integral. An integral operator is sometimes called an integral transformation. Thus, for Urysohn's integral operator (cf. Urysohn equation)
is given by an integral. An integral operator is sometimes called an integral transformation. Thus, for Urysohn's integral operator (cf. Urysohn equation)  , the law of the correspondence
, the law of the correspondence 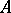 is given by the integral (or the operator
is given by the integral (or the operator 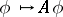 is generated by the integral)
is generated by the integral)
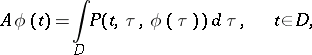 | (1) |
where 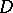 is a given measurable set of finite Lebesgue measure in a finite-dimensional space and
is a given measurable set of finite Lebesgue measure in a finite-dimensional space and 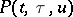 ,
, 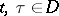 ,
,  , is a given measurable function. It is assumed that
, is a given measurable function. It is assumed that 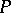 and
and 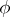 are functions satisfying conditions that ensure the existence of the integral in (1) in the sense of Lebesgue. If
are functions satisfying conditions that ensure the existence of the integral in (1) in the sense of Lebesgue. If 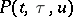 is a non-linear function in
is a non-linear function in  , then (1) is an example of a non-linear integral operator. If, on the other hand,
, then (1) is an example of a non-linear integral operator. If, on the other hand, 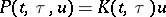 , then (1) takes the form
, then (1) takes the form
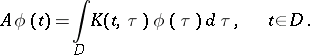 | (2) |
The operator generated by the integral in (2), or simply the operator (2), is called a linear integral operator, and the function  is called its kernel (cf. also Kernel of an integral operator).
is called its kernel (cf. also Kernel of an integral operator).
The kernel 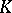 is called a Fredholm kernel if the operator (2) corresponding to
is called a Fredholm kernel if the operator (2) corresponding to 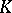 is completely continuous (compact) from a given function space
is completely continuous (compact) from a given function space 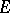 into another function space
into another function space 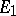 . In this case, the operator (2) is called a Fredholm integral operator from
. In this case, the operator (2) is called a Fredholm integral operator from 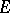 into
into 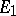 .
.
Linear integral operators are often considered in the following function spaces: 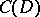 , the space of continuous functions on a bounded closed set
, the space of continuous functions on a bounded closed set 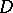 , and
, and 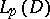 , the space of functions on
, the space of functions on 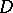 whose
whose 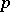 -th powers are integrable. In the first case (2) is called a Fredholm operator in
-th powers are integrable. In the first case (2) is called a Fredholm operator in 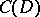 (i.e. from
(i.e. from  into
into 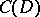 ) if
) if 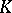 is continuous on
is continuous on  (
(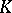 is then called a continuous kernel). The operator (2) is a Fredholm operator in
is then called a continuous kernel). The operator (2) is a Fredholm operator in 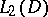 (from
(from 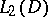 into
into 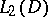 ) if
) if 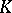 is measurable on
is measurable on 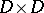 and if
and if
 | (3) |
Such a kernel is called an 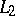 -kernel.
-kernel.
The adjoint of (2), in the complex function space 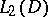 , with kernel satisfying (3), is the integral operator
, with kernel satisfying (3), is the integral operator
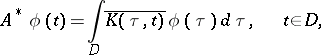 | (4) |
where the bar means transition to the complex conjugate. If 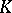 is a Hermitian (symmetric) kernel (i.e.
is a Hermitian (symmetric) kernel (i.e. 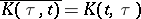 ), then the corresponding Fredholm operator (2) coincides with its adjoint (4). Operators with this property are called self-adjoint (cf. Self-adjoint operator). A Fredholm operator with a symmetric kernel is called a Hilbert–Schmidt integral operator.
), then the corresponding Fredholm operator (2) coincides with its adjoint (4). Operators with this property are called self-adjoint (cf. Self-adjoint operator). A Fredholm operator with a symmetric kernel is called a Hilbert–Schmidt integral operator.
If  denotes the distance between two points
denotes the distance between two points 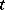 and
and  in the
in the  -dimensional Euclidean space and if
-dimensional Euclidean space and if 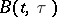 is a bounded measurable function on
is a bounded measurable function on 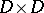 , then a kernel of the form
, then a kernel of the form
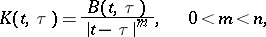 | (5) |
is called a kernel of potential type, and an operator (2) with such a kernel is called an integral operator of potential type. The kernel (5) is also called a polar kernel, or a kernel with weak singularity, while the corresponding operator (2) is called an integral operator with weak singularity.
If 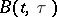 is a continuous function on
is a continuous function on 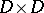 , the corresponding integral operator with weak singularity is completely continuous in
, the corresponding integral operator with weak singularity is completely continuous in  , while if
, while if  is a bounded measurable function on
is a bounded measurable function on 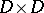 , the corresponding operator is completely continuous in
, the corresponding operator is completely continuous in  .
.
If the kernel 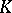 and the
and the 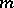 -dimensional set
-dimensional set 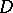 are such that the integral (2) does not exist in the sense of Lebesgue, but does exist in the sense of the Cauchy principal value, then the integral (2) is called an
are such that the integral (2) does not exist in the sense of Lebesgue, but does exist in the sense of the Cauchy principal value, then the integral (2) is called an 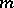 -dimensional singular integral (cf. also Singular integral). The operator generated by it is called an
-dimensional singular integral (cf. also Singular integral). The operator generated by it is called an 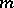 -dimensional singular integral operator, or a one-dimensional
-dimensional singular integral operator, or a one-dimensional  or multi-dimensional
or multi-dimensional 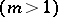 singular integral operator.
singular integral operator.
If a curve 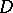 lies in the complex
lies in the complex 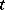 -plane, then
-plane, then
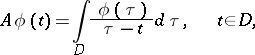 | (6) |
where the integral is understood in the sense of the Cauchy principal value, generates a continuous integral operator 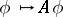 in the space of functions satisfying a Hölder condition (if
in the space of functions satisfying a Hölder condition (if  is a simple closed curve) or in
is a simple closed curve) or in 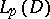 ,
, 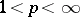 (if
(if 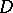 is a Lyapunov curve, cf. also Lyapunov surfaces and curves). The operator (6) is called a singular Cauchy operator.
is a Lyapunov curve, cf. also Lyapunov surfaces and curves). The operator (6) is called a singular Cauchy operator.
Suppose that two Lebesgue-measurable functions 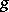 and
and 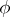 are given on the real axis. If for almost-all
are given on the real axis. If for almost-all 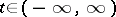 the integral
the integral
 |
exists, then one can define the function
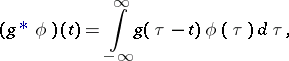 | (7) |
called the convolution of 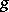 and
and 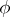 . If
. If 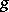 is fixed, (7) defines an operator
is fixed, (7) defines an operator
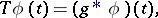 | (8) |
which is called the convolution integral operator (or convolution integral transform, cf. also Convolution transform) with kernel  .
.
If 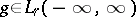 ,
,  , and
, and 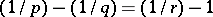 , then (8) is a continuous operator from
, then (8) is a continuous operator from 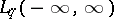 into
into 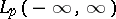 . Under corresponding conditions, convolution integral operations are used on a semi-axis as well as on a finite interval.
. Under corresponding conditions, convolution integral operations are used on a semi-axis as well as on a finite interval.
Apart from the integral operators above, concrete classes of integral operators have been studied, e.g. the integral transforms of Fourier, Laplace, Bessel, Mellin, Hilbert, etc.
References
| [1] | V.I. Smirnov, "A course of higher mathematics" , 5 , Addison-Wesley (1964) (Translated from Russian) |
| [2] | P.P. Zabreiko (ed.) A.I. Koshelev (ed.) M.A. Krasnoselskii (ed.) S.G. Mikhlin (ed.) L.S. Rakovshchik (ed.) V.Ya. Stet'senko (ed.) T.O. Shaposhnikova (ed.) R.S. Anderssen (ed.) , Integral equations - a reference text , Noordhoff (1975) (Translated from Russian) |
| [3] | M.A. Krasnosel'skii, et al., "Integral operators in spaces of summable functions" , Noordhoff (1976) (Translated from Russian) |
| [4] | V.A. Ditkin, A.P. Prudnikov, "Integral transforms and operational calculus" , Pergamon (1965) (Translated from Russian) |
| [5] | N.I. Muskhelishvili, "Singular integral equations" , Wolters-Noordhoff (1953) (Translated from Russian) |
| [6] | S.G. Mikhlin, "Multi-dimensional singular integrals and integral equations" , Pergamon (1965) (Translated from Russian) |
| [7] | E.C. Titchmarsh, "Introduction to the theory of Fourier integrals" , Oxford Univ. Press (1948) |
| [8] | R.E. Edwards, "Functional analysis: theory and applications" , Holt, Rinehart & Winston (1965) |
Comments
References
| [a1] | I.C. Gohberg, S. Goldberg, "Basic operator theory" , Birkhäuser (1981) |
| [a2] | P.R. Halmos, V.S. Sunder, "Bounded integral operators on 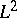 spaces" , Springer (1978) spaces" , Springer (1978) |
| [a3] | K. Jörgens, "Lineare Integraloperatoren" , Teubner (1970) |
| [a4] | A.C. Zaanen, "Linear analysis" , North-Holland (1956) |
Integral operator. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Integral_operator&oldid=15387