Integral invariant
of degree (order)  , of a smooth dynamical system
, of a smooth dynamical system
An absolute integral invariant is an exterior differential form  of degree
of degree  that is transformed into itself by the transformations generated by this system.
that is transformed into itself by the transformations generated by this system.
A relative integral invariant is an exterior differential form 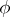 of degree
of degree  whose exterior differential is an absolute integral invariant (of degree
whose exterior differential is an absolute integral invariant (of degree  ).
).
One usually speaks of integral invariants of a flow (continuous-time dynamical system)  defined by a system of ordinary linear differential equations
defined by a system of ordinary linear differential equations  , where
, where  is a smooth vector field given on some domain in a Euclidean space (or on a manifold). In coordinates (local coordinates in the case of a manifold) this system has the form
is a smooth vector field given on some domain in a Euclidean space (or on a manifold). In coordinates (local coordinates in the case of a manifold) this system has the form
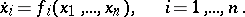 | (1) |
An important example of an integral invariant is a volume form  (where
(where  is a positive locally integrable (often even continuous or smooth) function in the coordinates). For smooth
is a positive locally integrable (often even continuous or smooth) function in the coordinates). For smooth 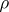 this form is an absolute invariant of (1) if
this form is an absolute invariant of (1) if
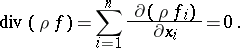 |
In this case the flow has an invariant measure 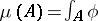 , which is given in (local) coordinates by its density
, which is given in (local) coordinates by its density 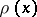 (the latter is often called an integral invariant, allowing for some ambiguity of speech).
(the latter is often called an integral invariant, allowing for some ambiguity of speech).
A Hamiltonian system with (generalized) momenta and coordinates  ,
,  , has the relative integral invariant
, has the relative integral invariant
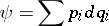 |
and the absolute integral invariant
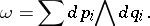 |
This fact may be put at the basis of the definition of a Hamiltonian system and may be used to develop the theory of Hamiltonian systems, since many specific properties of such systems are directly related to these integral invariants (cf. [4], [5]). The exterior powers 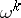 (including the volume form
(including the volume form 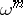 ) are absolute, while the products
) are absolute, while the products 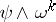 are relative integral invariants of any Hamiltonian system. Therefore, they are called universal integral invariants of Hamiltonian systems. Up to a multiplier, all universal integral invariants of Hamiltonian systems can be reduced to the ones indicated (cf. [4], [7]).
are relative integral invariants of any Hamiltonian system. Therefore, they are called universal integral invariants of Hamiltonian systems. Up to a multiplier, all universal integral invariants of Hamiltonian systems can be reduced to the ones indicated (cf. [4], [7]).
If (1) has an absolute integral invariant  of degree
of degree 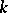 , then for any
, then for any 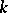 -dimensional smooth chain
-dimensional smooth chain  (e.g., for a smooth
(e.g., for a smooth 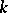 -dimensional manifold),
-dimensional manifold),
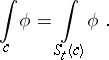 | (2) |
If (1) has a relative integral invariant, then (2) holds, generally speaking, only when the chain is the boundary of a chain of dimension  . Sometimes relative integral invariants are defined by the stronger condition that (2) holds for all cycles
. Sometimes relative integral invariants are defined by the stronger condition that (2) holds for all cycles  . Initially, integral invariants were defined by H. Poincaré (cf. [1], [2]) as integrals of the type above that remain invariant under the action of the flow on the domain of integration.
. Initially, integral invariants were defined by H. Poincaré (cf. [1], [2]) as integrals of the type above that remain invariant under the action of the flow on the domain of integration.
All that has been said can easily be generalized to non-autonomous systems 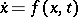 . The modification given by E. Cartan (cf. [3]) appears to be most essential. It involves the transition (even in the autonomous case) to an extended phase space (one adds time to the ordinary phase coordinates), in which the integral curves (cf. Integral curve) of the system of differential equations considered form a certain family of lines (a congruence, cf. Congruence of lines). Cartan requires that the integral of a form
. The modification given by E. Cartan (cf. [3]) appears to be most essential. It involves the transition (even in the autonomous case) to an extended phase space (one adds time to the ordinary phase coordinates), in which the integral curves (cf. Integral curve) of the system of differential equations considered form a certain family of lines (a congruence, cf. Congruence of lines). Cartan requires that the integral of a form 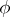 over a chain
over a chain  (or over a cycle, if one discusses a relative integral invariant) remains invariant if each point
(or over a cycle, if one discusses a relative integral invariant) remains invariant if each point  moves along the integral curve passing through this point; different points may move in different ways as long as this gives a smooth deformation of
moves along the integral curve passing through this point; different points may move in different ways as long as this gives a smooth deformation of  . (E.g., in the new sense it is not
. (E.g., in the new sense it is not 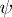 that is a relative integral invariant of Hamiltonian systems, but the extremely useful Cartan–Poincaré integral invariant
that is a relative integral invariant of Hamiltonian systems, but the extremely useful Cartan–Poincaré integral invariant  , where
, where 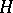 is the Hamiltonian, cf. [3]–[5].) A related definition is given in [6].
is the Hamiltonian, cf. [3]–[5].) A related definition is given in [6].
References
| [1] | H. Poincaré, "Sur le problème des trois corpses et les équations de la dynamique" Acta Math. , 13 (1890) pp. 1–270 |
| [2] | H. Poincaré, "Les méthodes nouvelles de la mécanique céleste" , 3 , Gauthier-Villars (1899) pp. Chapt. 26 |
| [3] | E. Cartan, "Leçons sur les invariants intégraux" , Hermann (1922) |
| [4] | F.R. [F.R. Gantmakher] Gantmacher, "Lectures in analytical mechanics" , MIR (1975) (Translated from Russian) |
| [5] | V.I. Arnol'd, "Mathematical methods of classical mechanics" , Springer (1978) (Translated from Russian) |
| [6] | C. Godbillon, "Géométrie différentielle et mécanique analytique" , Hermann (1969) |
| [7] | Hwa-Chung Lee, "The universal invariants of Hamilton systems and applications to the theory of canonical transformations" Proc. Roy. Soc. Edinburgh Ser. A , 62 (1947) pp. 237–246 |
Integral invariant. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Integral_invariant&oldid=12165