Hyperboloid
A non-closed central surface of the second order. One distinguishes between two types of hyperboloids: the one-sheet and the two-sheet hyperboloid.

Figure: h048360a

Figure: h048360b
In a suitable coordinate system (see Fig. a, Fig. b) the equation of a one-sheet hyperboloid is
 |
while that of a two-sheet hyperboloid is
 |
The numbers  ,
,  and
and  (and segments of such lengths) are known as the semi-axes of the hyperboloid. Sections of a hyperboloid by planes passing through the
(and segments of such lengths) are known as the semi-axes of the hyperboloid. Sections of a hyperboloid by planes passing through the  -axis are hyperbolas. Sections of a hyperboloid by planes perpendicular to the
-axis are hyperbolas. Sections of a hyperboloid by planes perpendicular to the  -axis are ellipses. The section of a one-sheet hyperboloid by the plane
-axis are ellipses. The section of a one-sheet hyperboloid by the plane  is said to be a gorge ellipse. A hyperboloid has three planes of symmetry. The cone defined by the equation
is said to be a gorge ellipse. A hyperboloid has three planes of symmetry. The cone defined by the equation
 |
is called the asymptotic cone. If 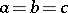 , the hyperboloid is said to be regular. A hyperboloid with two equal semi-axes is said to be a hyperboloid of rotation. A one-sheet hyperboloid is a ruled surface; the equations of the rectilinear generators passing through a given point
, the hyperboloid is said to be regular. A hyperboloid with two equal semi-axes is said to be a hyperboloid of rotation. A one-sheet hyperboloid is a ruled surface; the equations of the rectilinear generators passing through a given point  have the form
have the form
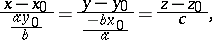 |
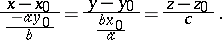 |
Comments
References
| [a1] | M. Berger, "Geometry" , 1–2 , Springer (1987) (Translated from French) |
| [a2] | D. Hilbert, S.E. Cohn-Vossen, "Geometry and the imagination" , Chelsea (1952) (Translated from German) |
Hyperboloid. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Hyperboloid&oldid=14677