Hyperbola
A planar curve which is obtained as the intersection of a circular cone with a plane not passing through the apex of the cone and intersecting both its sheets. A hyperbola is the set of points  of the surface (see Fig.) for which the modulus of the difference of the distance to two given points
of the surface (see Fig.) for which the modulus of the difference of the distance to two given points  and
and  (the foci of the hyperbola) is constant and equal to
(the foci of the hyperbola) is constant and equal to  . The distance between the foci of the hyperbola, known as its focal distance, is denoted by
. The distance between the foci of the hyperbola, known as its focal distance, is denoted by  .
.

Figure: h048230a
The midpoint of the segment  is known as the centre of the hyperbola. The straight line including the location of the foci of the hyperbola is said to be the real (or focal) axis of the hyperbola. The straight line through the centre of the hyperbola perpendicular to the real axis is called the imaginary axis of the hyperbola. The imaginary and real axes of the hyperbola are its axes of symmetry. The number
is known as the centre of the hyperbola. The straight line including the location of the foci of the hyperbola is said to be the real (or focal) axis of the hyperbola. The straight line through the centre of the hyperbola perpendicular to the real axis is called the imaginary axis of the hyperbola. The imaginary and real axes of the hyperbola are its axes of symmetry. The number  is said to be its eccentricity. A diameter of a hyperbola is any straight line passing through its centre. Midpoints of parallel chords of a hyperbola lie on a diameter of it. The directrix of a hyperbola corresponding to a given focus
is said to be its eccentricity. A diameter of a hyperbola is any straight line passing through its centre. Midpoints of parallel chords of a hyperbola lie on a diameter of it. The directrix of a hyperbola corresponding to a given focus  is the straight line
is the straight line  , perpendicular to the real axis, whose distance from the centre is
, perpendicular to the real axis, whose distance from the centre is  and which lies on the same side of the centre as
and which lies on the same side of the centre as  . A hyperbola has two directrices; it also has two asymptotes:
. A hyperbola has two directrices; it also has two asymptotes:
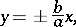 |
where  .
.
A hyperbola is a second-order curve. Its canonical equation has the form
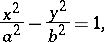 |
where  and
and 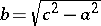 are the semi-axes of the hyperbola while
are the semi-axes of the hyperbola while  and
and 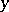 are the running coordinates. The equation of the tangent to the hyperbola at a point
are the running coordinates. The equation of the tangent to the hyperbola at a point 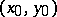 is
is
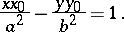 |
The focal parameter of a hyperbola (the half-length of the chord passing through the focus perpendicularly to the focal axis of the hyperbola) is equal to  . The equation of a hyperbola may be written in terms of the focal parameter
. The equation of a hyperbola may be written in terms of the focal parameter 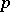 as
as
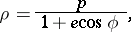 |
where 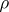 ,
, 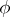 are polar coordinates and
are polar coordinates and 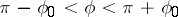 , where
, where 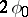 is the angle between the asymptotes.
is the angle between the asymptotes.
If 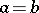 , the hyperbola is called equilateral. The asymptotes of an equilateral hyperbola are mutually perpendicular; if they are taken as the coordinate axes, then the equation of an equilateral hyperbola is
, the hyperbola is called equilateral. The asymptotes of an equilateral hyperbola are mutually perpendicular; if they are taken as the coordinate axes, then the equation of an equilateral hyperbola is
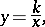 |
i.e. an equilateral hyperbola is the graph of an inversely-proportional relationship.
Comments
A definition involving only affine notions is as follows: A hyperbola is a conic with two real infinite points.
References
| [a1] | M. Berger, "Geometry" , 1–2 , Springer (1987) pp. Chapt. 17 (Translated from French) |
| [a2] | H.S.M. Coxeter, "Introduction to geometry" , Wiley (1961) |
Hyperbola. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Hyperbola&oldid=16761