Hunt-Stein theorem
A theorem stating conditions under which there exists a maximin invariant test in a problem of statistical hypothesis testing.
Suppose that based on the realization of a random variable 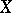 taking values in a sampling space
taking values in a sampling space 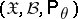 ,
, 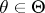 , it is necessary to test a hypothesis
, it is necessary to test a hypothesis 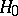 :
:  against an alternative
against an alternative 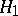 :
: 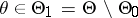 , and it is assumed that the family
, and it is assumed that the family 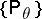 is dominated by a certain
is dominated by a certain  -finite measure
-finite measure 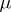 (cf. Domination). Next, suppose that on
(cf. Domination). Next, suppose that on 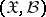 a transformation group
a transformation group 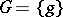 acts that leaves invariant the problem of testing the hypothesis
acts that leaves invariant the problem of testing the hypothesis 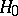 against
against 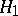 , and let
, and let 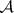 be the Borel
be the Borel  -field of subsets of
-field of subsets of  . The Hunt–Stein theorem asserts that if the following conditions hold:
. The Hunt–Stein theorem asserts that if the following conditions hold:
1) the mapping  is
is 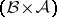 -measurable and
-measurable and 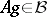 for every set
for every set 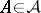 and any element
and any element 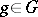 ;
;
2) on 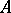 there exists an asymptotically right-invariant sequence of measures
there exists an asymptotically right-invariant sequence of measures 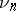 in the sense that for any
in the sense that for any  and
and 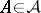 ,
,
 |
then for any statistical test intended for testing  against
against 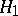 and with critical function
and with critical function 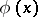 , there is an (almost-) invariant test with critical function
, there is an (almost-) invariant test with critical function  such that for all
such that for all 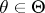 ,
,
 |
where 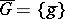 is the group induced by
is the group induced by 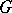 .
.
The Hunt–Stein theorem implies that if there exists a statistical test of level 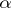 with critical function
with critical function 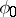 that maximizes
that maximizes 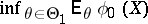 , then there also exists an (almost-) invariant test with the same property.
, then there also exists an (almost-) invariant test with the same property.
Condition 2) holds necessarily when  is a locally compact group on which a right-invariant Haar measure is given. The Hunt–Stein theorem shows that if
is a locally compact group on which a right-invariant Haar measure is given. The Hunt–Stein theorem shows that if 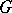 satisfies the conditions of the theorem, then in any problem of statistical hypothesis testing that is invariant relative to
satisfies the conditions of the theorem, then in any problem of statistical hypothesis testing that is invariant relative to 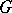 and on which there exists a uniformly most-powerful test, this test is a maximin test.
and on which there exists a uniformly most-powerful test, this test is a maximin test.
Conversely, suppose that in some problem of statistical hypotheses testing that is invariant under a group 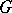 it is established that a uniformly most-powerful test is not a maximin test. This means that the conditions of the Hunt–Stein theorem are violated. In this connection there arises the question: Can a given test be maximin in another problem of hypothesis testing that is invariant under the same group
it is established that a uniformly most-powerful test is not a maximin test. This means that the conditions of the Hunt–Stein theorem are violated. In this connection there arises the question: Can a given test be maximin in another problem of hypothesis testing that is invariant under the same group  ? The answer to this question depends not only on the group
? The answer to this question depends not only on the group 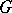 , but also on the family of distributions
, but also on the family of distributions 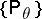 itself.
itself.
The theorem was obtained by G. Hunt and C. Stein in 1946, see [1].
References
| [1] | E.L. Lehmann, "Testing statistical hypotheses" , Wiley (1986) |
| [2] | S. Zachs, "The theory of statistical inference" , Wiley (1971) |
Hunt-Stein theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Hunt-Stein_theorem&oldid=16720