Holomorphic mapping
A mapping  of a domain
of a domain  into a domain
into a domain  under which
under which
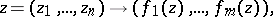 |
where all coordinate functions 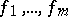 are holomorphic in
are holomorphic in 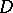 . If
. If 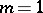 , a holomorphic mapping coincides with a holomorphic function (cf. Analytic function).
, a holomorphic mapping coincides with a holomorphic function (cf. Analytic function).
A holomorphic mapping is called non-degenerate at a point 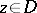 if the rank of the Jacobian matrix
if the rank of the Jacobian matrix 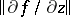 is maximal at
is maximal at  (and hence equals
(and hence equals 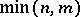 ). A holomorphic mapping is said to be non-degenerate in the domain
). A holomorphic mapping is said to be non-degenerate in the domain  if it is non-degenerate at all points
if it is non-degenerate at all points 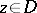 . If
. If  , the non-degeneracy of
, the non-degeneracy of 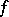 is equivalent to the condition
is equivalent to the condition
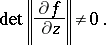 |
If 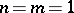 , a non-degenerate holomorphic mapping is a conformal mapping. If
, a non-degenerate holomorphic mapping is a conformal mapping. If 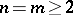 , a non-degenerate holomorphic mapping does not, in general, preserve angles between directions. If a holomorphic mapping
, a non-degenerate holomorphic mapping does not, in general, preserve angles between directions. If a holomorphic mapping 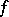 is non-degenerate at a point
is non-degenerate at a point  and if
and if  , then
, then 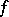 is locally invertible, i.e., then there exist neighbourhoods
is locally invertible, i.e., then there exist neighbourhoods  ,
, 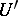 ,
, 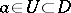 ,
,  , and a holomorphic mapping
, and a holomorphic mapping 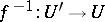 such that
such that 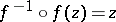 for all
for all  . If a holomorphic mapping
. If a holomorphic mapping 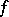 maps
maps 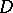 onto
onto 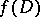 in a one-to-one correspondence and if
in a one-to-one correspondence and if  , then
, then 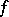 is non-degenerate in
is non-degenerate in 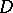 ; if
; if  , this is not true, e.g.
, this is not true, e.g. 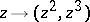 ,
, 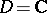 ,
, 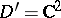 . If
. If 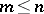 and if
and if 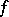 is non-degenerate in
is non-degenerate in  , then the image of
, then the image of  is also a domain in
is also a domain in  ; if
; if  , the principle of invariance of domain does not hold for mappings that are degenerate at certain points, e.g.
, the principle of invariance of domain does not hold for mappings that are degenerate at certain points, e.g. 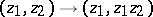 ,
, 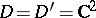 .
.
If 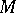 and
and 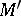 are complex manifolds,
are complex manifolds, 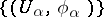 and
and 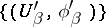 are atlases of their local coordinate systems (
are atlases of their local coordinate systems (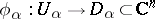 ,
,  are homeomorphisms; cf. Manifold), then a mapping
are homeomorphisms; cf. Manifold), then a mapping 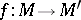 is said to be holomorphic if
is said to be holomorphic if 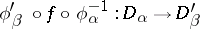 is a holomorphic mapping for all
is a holomorphic mapping for all  and
and 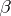 . Holomorphic mappings of complex spaces are defined in a similar manner (cf. Analytic mapping). See also Biholomorphic mapping.
. Holomorphic mappings of complex spaces are defined in a similar manner (cf. Analytic mapping). See also Biholomorphic mapping.
References
| [1] | R.C. Gunning, H. Rossi, "Analytic functions of several complex variables" , Prentice-Hall (1965) |
Comments
A non-degenerate mapping is also called non-singular.
References
| [a1] | W. Rudin, "Function theory in the unit ball in  " , Springer (1980) pp. Chapt. 15 " , Springer (1980) pp. Chapt. 15 |
| [a2] | S.G. Krantz, "Function theory of several complex variables" , Wiley (1982) |
Holomorphic mapping. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Holomorphic_mapping&oldid=14057