Hardy theorem

in the theory of functions of a complex variable

If is a regular analytic function in the disc 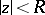, 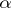is a positive number, and if

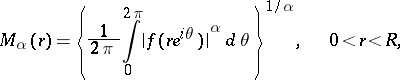is the average value, then is a non-decreasing function of that is logarithmically convex relative to 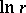(cf. Convexity, logarithmic). The theorem was established by G.H. Hardy [1].

The assertion on the logarithmic convexity remains valid for a function that is regular in an annulus 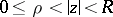(see [1]).

Hardy's theorem generalizes to subharmonic functions (cf. Subharmonic function) in a ball of 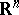, 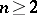(see also [2]).

References

[1]	G.H. Hardy, "The mean value of the modulus of an analytic function" Proc. London. Math. Soc. (2) , 14 (1915) pp. 269–277
[2]	I.I. Privalov, "Subharmonic functions" , Moscow-Leningrad (1937) (In Russian)

References

[a1]	T. Radó, "Subharmonic functions" , Springer (1937)
[a2]	P.L. Duren, "Theory of 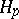spaces" , Acad. Press (1970)
[a3]	J.L. Doob, "Classical potential theory and its probabilistic counterpart" , Springer (1984) pp. 390