Gram determinant
A determinant of the form
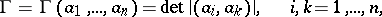 |
where  are elements of a (pre-)Hilbert space and
are elements of a (pre-)Hilbert space and  are their scalar products. A Gram determinant is equal to the square of the
are their scalar products. A Gram determinant is equal to the square of the  -dimensional volume of the parallelotope constructed on
-dimensional volume of the parallelotope constructed on  .
.
A Gram determinant is the determinant of a non-negative Hermitian form
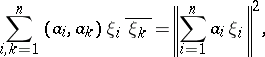 |
which determines its basic properties:
1) A Gram determinant is non-negative, i.e.  . The equality
. The equality  is valid if and only if the vectors are linearly dependent. This property can be regarded as a generalization of the Cauchy inequality:
is valid if and only if the vectors are linearly dependent. This property can be regarded as a generalization of the Cauchy inequality:
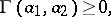 |
or
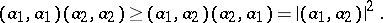 |
In particular, a Gram determinant is equal to zero if any of its principal minors (which is also a Gram determinant) is zero.
2)  , equality holding if and only if the subspaces
, equality holding if and only if the subspaces  and
and 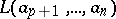 are orthogonal or if one of the determinants
are orthogonal or if one of the determinants 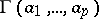 ,
,  is equal to zero. The geometrical meaning of this inequality is that the volume of the parallelotope is not larger than the product of the volumes of complementary faces. In particular,
is equal to zero. The geometrical meaning of this inequality is that the volume of the parallelotope is not larger than the product of the volumes of complementary faces. In particular,
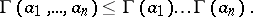 |
3)  , where
, where
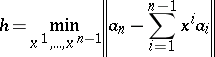 |
is the distance from the element  to the subspace
to the subspace  , i.e. the best quadratic approximation (cf. Best approximation) to the element
, i.e. the best quadratic approximation (cf. Best approximation) to the element  by polynomials of the type
by polynomials of the type 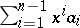 .
.
If  are
are  -dimensional vectors,
-dimensional vectors,  , then
, then
 |
Gram determinants were introduced by J.P. Gram [1] and, independently, by K.A. Andreev [2] in the context of problems of expansion of functions into orthogonal series and the best quadratic approximation to functions.
The Gram determinant is used in many problems of linear algebra and function theory: studies of linear dependence of systems of vectors or functions, orthogonalization of systems of functions, construction of projections, and also in studies on the properties of systems of functions. See also Gram matrix.
The Gram determinant is a special case of determinants of the type
 |
which are Hermitian and bilinear with respect to vectors  and
and 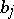 . If all
. If all  are of class
are of class  , then the following formula is valid:
, then the following formula is valid:
 |
 |
References
| [1] | J.P. Gram, "On Raekkeudviklinger bestemmte ved Hjaelp of de mindste Kvadraters Methode" , Copenhagen (1879) |
| [2] | K.A. Andreev, , Selected work , Khar'kov (1955) (In Russian) |
| [3] | F.R. [F.R. Gantmakher] Gantmacher, "The theory of matrices" , 1 , Chelsea, reprint (1977) (Translated from Russian) |
Comments
Using Gram determinants and their properties one can prove Hadamard's determinant theorem (cf. Hadamard theorem).
References
| [a1] | P.J. Davis, "Interpolation and approximation" , Dover, reprint (1975) pp. 108–126 |
Gram determinant. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Gram_determinant&oldid=18442