Ginzburg-Landau equation
A modulation (or amplitude/envelope equation) that describes the evolution of small perturbations of a marginally unstable basic state of a system of non-linear partial differential equations on an unbounded domain (cf. also Perturbation theory; Perturbation of a linear system; Linear partial differential equation). The stationary problem associated to the Ginzburg–Landau equation with real coefficients also has a different background as the Euler–Lagrange equation associated to the Ginzburg–Landau functional (see below). To obtain the Ginzburg–Landau equation as modulation equation, one lets
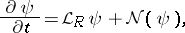 | (a1) |
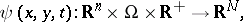 |
describe an underlying problem (with certain boundary conditions), where  is an elliptic linear operator,
is an elliptic linear operator,  a non-linear operator of order less than
a non-linear operator of order less than  ,
,  a (bifurcation) parameter and
a (bifurcation) parameter and  a bounded domain
a bounded domain  . The linearized stability of the basic solution
. The linearized stability of the basic solution  of (a1) is determined by setting
of (a1) is determined by setting 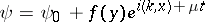 and solving an eigenvalue problem for
and solving an eigenvalue problem for 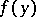 on
on  for any pair
for any pair 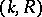 (
( ). Under certain conditions on the eigenvalue problem, one can define
). Under certain conditions on the eigenvalue problem, one can define  as the critical eigenvalue (i.e.
as the critical eigenvalue (i.e. 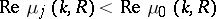 for all
for all 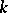 ,
, 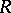 and
and 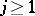 ) and
) and 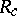 as the critical value of
as the critical value of 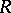 (
( for all
for all  and
and 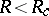 , i.e. the neutral manifold
, i.e. the neutral manifold  has a minimum at
has a minimum at  in
in 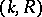 -space:
-space: 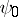 is linearly stable for
is linearly stable for 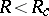 ). Introduce
). Introduce  ,
,  by
by 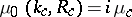 and
and  as the critical eigenfunction at
as the critical eigenfunction at 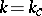 ,
, 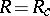 . For
. For 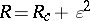 ,
,  ,
, 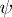 can be expanded both as an asymptotic series and as a Fourier series:
can be expanded both as an asymptotic series and as a Fourier series:
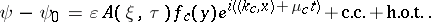 |
Here,  is an unknown amplitude and
is an unknown amplitude and  and
and  are rescaled variables:
are rescaled variables:
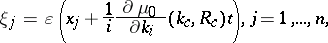 |
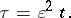 |
The Ginzburg–Landau equation describes the evolution of 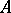 :
:
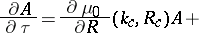 | (a2) |
 |
(at leading order). The equation is obtained (formally) by inserting the above expansion into (a1) and applying an orthogonality condition. The second-order differential operator is elliptic when 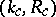 is an isolated non-degenerate minimum of the neutral manifold. The Landau constant
is an isolated non-degenerate minimum of the neutral manifold. The Landau constant 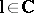 can be expressed in terms of information obtained from the linear eigenvalue problem and its adjoint. In most cases studied in the literature,
can be expressed in terms of information obtained from the linear eigenvalue problem and its adjoint. In most cases studied in the literature,  and
and  ; (a2) can then be rescaled into
; (a2) can then be rescaled into
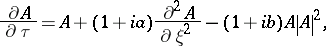 | (a3) |
with 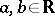 . Historically, the Ginzburg–Landau equation was first derived as a modulation equation for two classical hydrodynamic stability problems: Rayleigh–Bénard convection [a4] and Poiseuille flow [a5]. Several aspects of the mathematical validity of this formal approximation scheme have been studied in [a2], [a7], [a6].
. Historically, the Ginzburg–Landau equation was first derived as a modulation equation for two classical hydrodynamic stability problems: Rayleigh–Bénard convection [a4] and Poiseuille flow [a5]. Several aspects of the mathematical validity of this formal approximation scheme have been studied in [a2], [a7], [a6].
By its nature, the Ginzburg–Landau equation appears as leading-order approximation in many systems. Therefore, there is much literature on the behaviour of its solutions. Its most simple solutions are periodic: 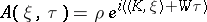 . Under certain conditions on the coefficients there is a subfamily of stable periodic solutions; it is called the Eckhaus band in (a3). The existence and stability of more complicated "localized" (homoclinic, heteroclinic) solutions to (a3) (also with more general non-linear terms) is considered in [a8] (with mostly formal results). Up to now (1998), there is no mathematical text (book or survey paper) that gives an overview of what is known about the behaviour of solutions of the Ginzburg–Landau equation as evolution equation on
. Under certain conditions on the coefficients there is a subfamily of stable periodic solutions; it is called the Eckhaus band in (a3). The existence and stability of more complicated "localized" (homoclinic, heteroclinic) solutions to (a3) (also with more general non-linear terms) is considered in [a8] (with mostly formal results). Up to now (1998), there is no mathematical text (book or survey paper) that gives an overview of what is known about the behaviour of solutions of the Ginzburg–Landau equation as evolution equation on 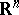 or even on
or even on  .
.
However, much is known about the solutions of the stationary (elliptic) Ginzburg–Landau equation with real coefficients on bounded domains  [a1]. The Ginzburg–Landau equation with real coefficients has a variational structure: the Ginzburg–Landau functional
[a1]. The Ginzburg–Landau equation with real coefficients has a variational structure: the Ginzburg–Landau functional
 |
The Ginzburg–Landau functional appears in various parts of science; in general, it is not related to the above sketched modulation equation interpretation of the Ginzburg–Landau equation [a1]. The name "Ginzburg–Landau" , both of the equation and of the functional, comes from a paper on superconductivity [a3]. However, in this context the (real, stationary) equation and/or the functional is part of a larger system of equations/functionals.
References
| [a1] | F. Bethuel, H. Brezis, F. Hélein, "Ginzburg–Landau vortices" , Birkhäuser (1994) |
| [a2] | P. Collet, J.P. Eckmann, "The time-dependent amplitude equation for the Swift–Hohenberg problem" Comm. Math. Phys. , 132 (1990) pp. 139–153 |
| [a3] | V.L. Ginzburg, L.D. Landau, "On the theory of superconductivity" Zh. Eksper. Teor. Fiz. , 20 (1950) pp. 1064–1082 (In Russian) (English transl.: Men of Physics: L.D. Landau (D. ter Haar, ed.), Pergamon, 1965,138-167) |
| [a4] | A.C. Newell, J.A. Whitehead, "Finite bandwidth, finite amplitude convection" J. Fluid Mech. , 38 (1969) pp. 279–303 |
| [a5] | K. Stewartson, J.T. Stuart, "A non-linear instability theory for a wave system in plane Poiseuille flow" J. Fluid Mech. , 48 (1971) pp. 529–545 |
| [a6] | G. Schneider, "Global existence via Ginzburg–Landau formalism and pseudo-orbits of the Ginzburg–Landau approximations" Comm. Math. Phys. , 164 (1994) pp. 159–179 |
| [a7] | A. van Harten, "On the validity of the Ginzburg–Landau's equation" J. Nonlinear Sci. , 1 (1991) pp. 397–422 |
| [a8] | W. van Saarloos, P.C. Hohenberg, "Fronts, pulses, sources and sinks in generalised complex Ginzburg–Landau equations" Physica D , 56 (1992) pp. 303–367 |
Ginzburg-Landau equation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Ginzburg-Landau_equation&oldid=16717