Geometry of numbers
geometric number theory
The branch of number theory that studies number-theoretical problems by the use of geometric methods. Geometry of numbers in its proper sense was formulated by H. Minkowski in 1896 in his fundamental monograph [1]. The starting point of this science, which subsequently became an independent branch of number theory, is the fact (already noted by Minkowski) that certain assertions which seem evident in the context of figures in an  -dimensional Euclidean space have far-reaching consequences in number theory.
-dimensional Euclidean space have far-reaching consequences in number theory.
A fundamental and typical task of the geometry of numbers is the problem to determine the arithmetical minimum  of some real function
of some real function
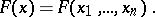 |
Here 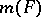 is the infimum of the values of
is the infimum of the values of 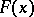 when
when  runs through all the integral points (i.e. points with integer coordinates) that satisfy some supplementary condition (e.g.
runs through all the integral points (i.e. points with integer coordinates) that satisfy some supplementary condition (e.g. 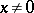 ). In the most important special cases information on
). In the most important special cases information on 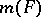 can be obtained from Minkowski's convex-body theorem, which may be formulated as follows. Let
can be obtained from Minkowski's convex-body theorem, which may be formulated as follows. Let 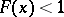 be an
be an  -dimensional convex body of volume
-dimensional convex body of volume 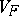 and let
and let 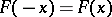 and
and 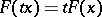 for
for 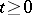 ; then
; then
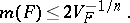 |
The quantity  is useful in considering conditions of existence of solutions of the Diophantine inequality (cf. Diophantine approximations)
is useful in considering conditions of existence of solutions of the Diophantine inequality (cf. Diophantine approximations)
 |
This is a problem to which many problems in number theory can be reduced. The geometry of quadratic forms (cf. Quadratic form) forms a separate chapter in the geometry of numbers.
Two general types of problems are distinguished in the geometry of numbers: the homogeneous and the inhomogeneous problem.
The homogeneous problem, which forms the subject of most studies in the geometry of numbers, deals with the homogeneous minima 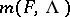 of a distance function (cf. Ray function)
of a distance function (cf. Ray function) 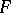 on a lattice of points
on a lattice of points  . The concept of a lattice (of points) is a fundamental one in the geometry of numbers. Let
. The concept of a lattice (of points) is a fundamental one in the geometry of numbers. Let  be linearly independent vectors in an
be linearly independent vectors in an  -dimensional Euclidean space. The set of points
-dimensional Euclidean space. The set of points
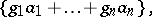 |
when  each run through all the integers in an independent manner, is known as the lattice (of points)
each run through all the integers in an independent manner, is known as the lattice (of points) 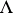 with basis
with basis  and determinant
and determinant
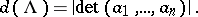 |
Let a distance function 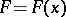 and a lattice
and a lattice 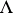 with determinant
with determinant  be given in
be given in 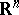 . The greatest lower bound
. The greatest lower bound
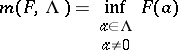 |
of the values of 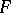 over the points
over the points 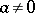 of
of  is called the minimum of
is called the minimum of  on
on  (or, more accurately, the homogeneous arithmetical minimum). The greatest lower bound
(or, more accurately, the homogeneous arithmetical minimum). The greatest lower bound  , which may or may not be attained, is known to be attained by a bounded star body (cf. Star-like domain), which is defined by the inequality
, which may or may not be attained, is known to be attained by a bounded star body (cf. Star-like domain), which is defined by the inequality
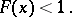 |
In order to estimate 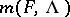 from above one must calculate (or estimate) the constant of Hermite
from above one must calculate (or estimate) the constant of Hermite 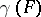 of the distance function
of the distance function 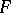 , defined by
, defined by
 |
where the supremum is taken over the set  of all
of all  -dimensional lattices
-dimensional lattices 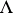 . There are relations between
. There are relations between 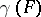 , the critical determinant (see below)
, the critical determinant (see below)  of the set
of the set 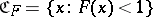 and (if
and (if 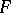 is a convex symmetric distance function) the density
is a convex symmetric distance function) the density 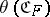 of the densest lattice packing of the body
of the densest lattice packing of the body  .
.
Let a set 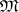 and a lattice
and a lattice 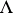 with determinant
with determinant 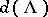 be given in
be given in 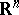 . The lattice
. The lattice 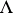 is called admissible for
is called admissible for 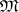 , or
, or 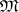 -admissible, if
-admissible, if 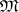 contains no non-zero points from
contains no non-zero points from  . A set
. A set 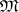 with at least one admissible lattice is called a set of finite type; otherwise
with at least one admissible lattice is called a set of finite type; otherwise 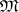 is called a set of infinite type. Let
is called a set of infinite type. Let  be a set of finite type; the infimum
be a set of finite type; the infimum
 |
of the set of determinants 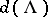 of all
of all 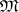 -admissible lattices
-admissible lattices 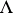 is called the critical determinant
is called the critical determinant 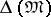 of
of 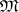 . Any
. Any  -admissible lattice
-admissible lattice 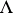 that satisfies the condition
that satisfies the condition
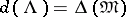 |
is called a critical lattice of 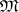 . For a set
. For a set 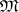 of infinite type one defines
of infinite type one defines  .
.
The calculation of the constant of Hermite 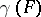 of a distance function
of a distance function 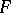 is reduced to the computation of the critical determinant
is reduced to the computation of the critical determinant 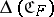 of the star body
of the star body 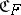 defined by
defined by 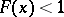 :
:
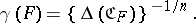 |
The connection between the critical determinant and the density of the densest lattice packing is established by the following theorem of Blichfeldt. Let 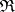 be an arbitrary set, let
be an arbitrary set, let 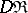 be the corresponding set of differences (i.e. the set of points
be the corresponding set of differences (i.e. the set of points  , where
, where  ) and let
) and let 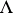 be a lattice. For the arrangement
be a lattice. For the arrangement  , i.e. for the family of sets
, i.e. for the family of sets 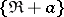 , where
, where  , to be a packing it is necessary and sufficient that
, to be a packing it is necessary and sufficient that 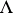 be
be 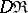 -admissible.
-admissible.
The density  of the densest lattice packing of a bounded Lebesgue-measurable set
of the densest lattice packing of a bounded Lebesgue-measurable set 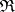 of measure
of measure  is defined by
is defined by
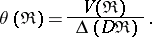 |
For an arbitrary set 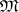 and a Lebesgue-measurable set
and a Lebesgue-measurable set 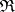 of measure
of measure 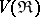 that satisfies the condition
that satisfies the condition 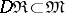 the following inequality (another formulation of Blichfeldt's theorem) is valid:
the following inequality (another formulation of Blichfeldt's theorem) is valid:
 |
If 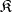 is a convex body that is symmetric with respect to a point
is a convex body that is symmetric with respect to a point 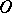 , then
, then
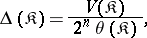 |
where 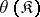 is the density of the densest lattice packing of
is the density of the densest lattice packing of  . This means that in the case of a symmetric distance function
. This means that in the case of a symmetric distance function 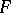 the computation of
the computation of  is reduced to the computation of the densest lattice packing of the body
is reduced to the computation of the densest lattice packing of the body 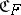 defined by
defined by 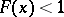 .
.
A very important statement in the geometry of numbers is Minkowski's convex-body theorem. Let 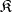 be a convex body that is symmetric with respect to the coordinate origin and of volume
be a convex body that is symmetric with respect to the coordinate origin and of volume 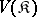 . Then
. Then
 | (1) |
In other words, a lattice  for which
for which
 |
has a point distinct from zero in  .
.
Inequality (1) is known as the Minkowski inequality; it gives an estimate from below for the critical determinant  of a convex body
of a convex body 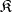 that is symmetric with respect to 0. In the general case this estimate cannot be improved. Equality is attained if and only if
that is symmetric with respect to 0. In the general case this estimate cannot be improved. Equality is attained if and only if 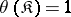 . Convex bodies
. Convex bodies 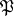 that satisfy the condition
that satisfy the condition 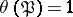 are known as parallelohedra. They play an important role in the geometry of numbers and in mathematical crystallography (cf. Crystallography, mathematical).
are known as parallelohedra. They play an important role in the geometry of numbers and in mathematical crystallography (cf. Crystallography, mathematical).
All applications of Minkowski's convex-body theorem are based on the fact that for a convex symmetric distance function  and an arbitrary lattice
and an arbitrary lattice 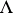 of determinant
of determinant 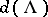 the following inequality is valid:
the following inequality is valid:
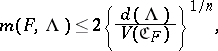 |
where
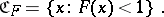 |
In particular, for the lattice 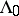 of integral points and the distance function
of integral points and the distance function
 |
Minkowski's theorem on linear homogeneous forms is valid: Let 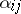 ,
, 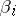 be real numbers,
be real numbers,  ;
; 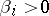 ,
, 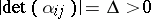 . If
. If
 |
then there exist integers  , not all equal to zero, satisfying the system of linear inequalities
, not all equal to zero, satisfying the system of linear inequalities
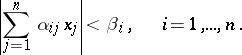 |
Geometry of numbers also studies the successive minima of a distance function on a lattice. Let 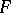 be a distance function, let
be a distance function, let 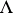 be a lattice and let there be given an index
be a lattice and let there be given an index 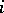 ,
, 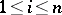 ; then the infimum of the numbers
; then the infimum of the numbers 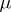 for which the set
for which the set 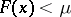 contains at least
contains at least 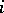 linearly independent points of
linearly independent points of 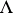 is said to be the
is said to be the 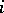 -th successive minimum
-th successive minimum 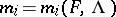 of
of  on
on  . Here
. Here
 |
The estimate
 |
is valid. It is more difficult to estimate the magnitude
 |
from above; to do this, one must be able to compute, or to estimate from above, the quantity
 |
where the supremum is over all  -dimensional lattices
-dimensional lattices 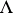 . The quantity
. The quantity 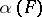 is called the anomaly of the distance function
is called the anomaly of the distance function  , or the anomaly of the set
, or the anomaly of the set 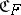 . The inequality
. The inequality  is valid. The following theorem [4] gives an estimate from above for
is valid. The following theorem [4] gives an estimate from above for 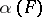 . Let
. Let 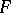 be an
be an  -dimensional distance function with anomaly
-dimensional distance function with anomaly 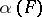 , then
, then
 |
Examples have been constructed to show that this estimate cannot, generally speaking, be improved.
If 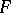 is a convex symmetric distance function, it has been conjectured (the hypothesis on the anomaly of a convex body) that
is a convex symmetric distance function, it has been conjectured (the hypothesis on the anomaly of a convex body) that
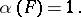 |
Minkowski's second theorem on a convex body, making precise the first theorem, is valid. If  is a convex symmetric distance function and if
is a convex symmetric distance function and if 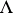 is a lattice, then
is a lattice, then
 |
where the convex body 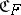 is defined by the condition
is defined by the condition  . Minkowski's second theorem is valid [4] independently of the hypothesis on the anomaly of a convex body.
. Minkowski's second theorem is valid [4] independently of the hypothesis on the anomaly of a convex body.
The concept of successive minima and the fundamental results relevant to it (except for the last-named theorem) can be generalized from star bodies  to arbitrary sets
to arbitrary sets 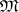 [9].
[9].
The following statement is an estimate from above of the critical determinant of a given set: For any Lebesgue-measurable set 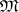 of measure
of measure 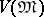 ,
,
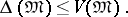 | (2) |
If 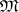 is a star body that is symmetric with respect to zero, then
is a star body that is symmetric with respect to zero, then
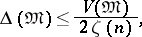 | (3) |
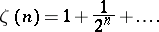 |
All proofs of this theorem include some averaging of some function given on the space of lattices. The most natural proof is given by Siegel's mean-value theorem (see, e.g., [12]). Let 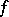 be a Lebesgue-integrable function on the
be a Lebesgue-integrable function on the  -dimensional Euclidean space
-dimensional Euclidean space 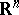 , and let
, and let  be an invariant measure on the space of lattices
be an invariant measure on the space of lattices 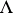 with determinant 1. Let
with determinant 1. Let 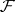 be the fundamental domain of this space, then
be the fundamental domain of this space, then
 |
As distinct from the estimate from below (1), estimates (2) and (3) are not the best possible (for more precise estimates see [13]).
Estimates of the critical determinant  of a given set
of a given set 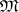 from below and from above yield estimates of
from below and from above yield estimates of 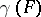 from above and from below, i.e. the solution (in a certain sense) of the homogeneous problem in the geometry of numbers. However, it is often important to know the exact value of the critical determinant
from above and from below, i.e. the solution (in a certain sense) of the homogeneous problem in the geometry of numbers. However, it is often important to know the exact value of the critical determinant 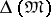 for a given set
for a given set 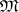 (e.g., for a norm body of a given algebraic number field). If
(e.g., for a norm body of a given algebraic number field). If  is a given bounded star body, then it is possible, in principle, to find an algorithm which permits one to reduce the problem of finding all critical lattices of
is a given bounded star body, then it is possible, in principle, to find an algorithm which permits one to reduce the problem of finding all critical lattices of 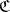 (and hence
(and hence 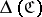 as well) to a finite number of ordinary problems on the extrema of certain functions of several variables. However, this algorithm is realizable (in the present state of knowledge) only for convex bodies
as well) to a finite number of ordinary problems on the extrema of certain functions of several variables. However, this algorithm is realizable (in the present state of knowledge) only for convex bodies 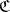 when the dimension
when the dimension 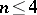 [4].
[4].
Generally speaking, finding 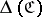 is much more difficult for unbounded star bodies
is much more difficult for unbounded star bodies 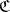 ; this is clear by the isolation phenomenon of homogeneous arithmetical minima, which may be described as follows. Let
; this is clear by the isolation phenomenon of homogeneous arithmetical minima, which may be described as follows. Let 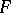 be a distance function in
be a distance function in 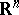 , and let the functional
, and let the functional
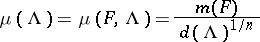 |
be given on the set  of all lattices
of all lattices 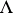 . The set
. The set 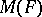 of possible values of
of possible values of 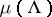 for all
for all 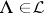 is called the Markov spectrum of
is called the Markov spectrum of 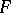 . One says that
. One says that  has the isolation phenomenon if the set
has the isolation phenomenon if the set 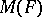 has isolated points. The set
has isolated points. The set  lies in the interval
lies in the interval 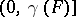 . If the star body
. If the star body 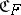 ,
, 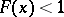 , is bounded, then
, is bounded, then
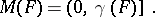 |
For this reason the isolation phenomenon is possible for unbounded star bodies only (cf. [4], Chapt. X). The most intensively studied case is 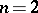 ,
,
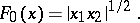 | (4) |
A.N. Korkin and E.I. Zolotarev [14] were the first to note the isolation phenomenon in this case (which was also the first case of the isolation phenomenon ever noted). A.A. Markov (see [14]) proved in 1879 that the part of the spectrum 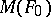 to the right of
to the right of 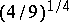 is discrete, and has the form
is discrete, and has the form
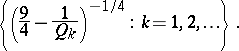 | (5) |
Here  is an increasing sequence of positive integers with the following property: It is possible to find integers
is an increasing sequence of positive integers with the following property: It is possible to find integers  ,
, 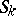 such that
such that
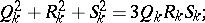 |
to each point of the spectrum (5) (the "Markov spectrum" in the narrow sense) there corresponds a unique (up to automorphisms [4]) lattice  . The indefinite form
. The indefinite form 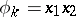 ,
, 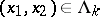 , is sometimes called the Markov form, while the sequence
, is sometimes called the Markov form, while the sequence 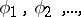 is called a Markov chain. It is also known that to the left of some number
is called a Markov chain. It is also known that to the left of some number 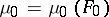 the spectrum
the spectrum 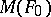 coincides with the segment
coincides with the segment 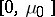 . The isolation phenomenon can be described in terms of admissible lattices (cf. [9]), which generalizes this concept somewhat.
. The isolation phenomenon can be described in terms of admissible lattices (cf. [9]), which generalizes this concept somewhat.
The inhomogeneous problem comprises the inhomogeneous Diophantine problems which play an important role in number theory; it forms an important branch of the geometry of numbers.
Let 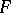 be a distance function in
be a distance function in 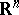 , let
, let  be a lattice of determinant
be a lattice of determinant 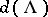 in
in  and let
and let 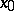 be a point in
be a point in  . Consider the quantities
. Consider the quantities
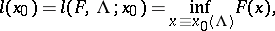 |
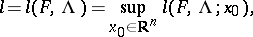 |
where the infimum is over all points of the form 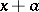 ,
, 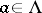 , while the supremum is over all points
, while the supremum is over all points 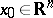 . The quantity
. The quantity  is called the inhomogeneous arithmetical minimum of
is called the inhomogeneous arithmetical minimum of 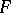 on
on 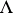 ; this "minimum" need not be attained.
; this "minimum" need not be attained.  is the greatest lower bound of the real numbers
is the greatest lower bound of the real numbers  having the following property: The arrangement
having the following property: The arrangement 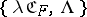 of the set
of the set 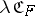 , where
, where 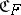 satisfies the condition
satisfies the condition 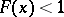 , over the lattice
, over the lattice 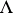 is a covering, i.e.
is a covering, i.e.
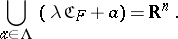 |
For the distance function 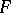 one considers the following analogues of the Hermite constant:
one considers the following analogues of the Hermite constant:
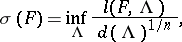 |
 |
where the infimum (supremum) is over all  -dimensional lattices
-dimensional lattices  . The quantity
. The quantity 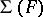 is usually trivial (cf. [4]); if the set
is usually trivial (cf. [4]); if the set 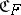 ,
, 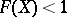 , has a finite volume, then
, has a finite volume, then
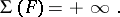 |
However, the inhomogeneous problem is connected with 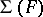 in one particular instance of the function
in one particular instance of the function  which is of interest.
which is of interest.
The hypothesis on the product of inhomogeneous linear forms may be stated as follows. Let
 |
then
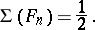 |
Studies on this hypothesis and its analogues account for more than one half of all studies on the inhomogeneous problem in the geometry of numbers (cf. Minkowski hypothesis).
In the general case,  is more informative than
is more informative than 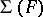 . It is closely related to the value of the density
. It is closely related to the value of the density 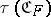 of the most economical covering by the body
of the most economical covering by the body 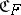 [7], [10]. In fact, if
[7], [10]. In fact, if 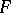 is a distance function and if the set
is a distance function and if the set 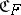 is bounded, then
is bounded, then
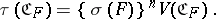 |
An important chapter of the inhomogeneous problems in the geometry of numbers is constituted by the so-called transference theorems for a given distance function  , which are inequalities connecting the inhomogeneous minimum
, which are inequalities connecting the inhomogeneous minimum 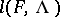 with the successive homogeneous minima
with the successive homogeneous minima 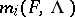 (or with the minima of the reciprocal function
(or with the minima of the reciprocal function 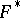 with respect to the reciprocal lattice
with respect to the reciprocal lattice 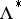 , etc., see [4]). Example. Let
, etc., see [4]). Example. Let 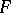 be a convex symmetric distance function and let
be a convex symmetric distance function and let 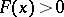 for
for 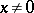 ; then, for any lattice
; then, for any lattice  ,
,
 |
There exist generalizations of the geometry of numbers to include spaces more general than 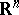 and also to discrete sets more general than
and also to discrete sets more general than 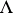 [15], [10].
[15], [10].
References
| [1] | H. Minkowski, "Geometrie der Zahlen" , Chelsea, reprint (1953) |
| [2] | H. Minkowski, "Diophantische Approximationen" , Chelsea, reprint (1957) |
| [3] | H. Hancock, "Development of the Minkowski geometry of numbers" , Macmillan (1939) |
| [4] | J.W.S. Cassels, "An introduction to the geometry of numbers" , Springer (1959) |
| [5] | P.M. Gruber, C.G. Lekkerkerker, "Geometry of numbers" , North-Holland (1987) (Updated reprint) |
| [6] | L. Fejes Toth, "Lagerungen in der Ebene, auf der Kugel und im Raum" , Springer (1972) |
| [7] | C.A. Rogers, "Packing and covering" , Cambridge Univ. Press (1964) |
| [8] | O.-H. Keller, "Geometrie der Zahlen" , Enzyklopaedie der math. Wissenschaften mit Einschluss ihrer Anwendungen , 12 (1954) (Heft 11, Teil III) |
| [9] | E. Hlawka, "Grundbegriffe der Geometrie der Zahlen" Jahresber. Deutsch. Math.-Verein , 57 (1954) pp. 37–55 |
| [10] | E.P. Baranovskii, "Packings, coverings, partitionings and certain other distributions in spaces of constant curvature" Progress Math. , 9 (1971) pp. 209–253 Itogi Nauk. Algebra Topol. Geom. 1967 (1969) pp. 189–225 |
| [11] | J.F. Koksma, "Diophantische Approximationen" , Springer (1936) |
| [12] | A.M. Macbeath, C.A. Rogers, "Siegel's mean value theorem in the geometry of numbers" Proc. Cambridge Philos. Soc. (2) , 54 (1958) pp. 139–151 |
| [13] | W. Schmidt, "On the Minkowski–Hlawka theorem" Illinois J. Math. , 7 (1963) pp. 18–23; 714 |
| [14] | A.M. Markov, "On binary quadratic forms with positive determinant" Uspekhi Mat. Nauk , 3 : 5 (1948) pp. 7–51 (In Russian) |
| [15] | K. Rogers, H.P.F. Swinnerton-Dyer, "The geometry of numbers over algebraic number fields" Trans. Amer. Math. Soc. , 88 (1958) pp. 227–242 |
Comments
A distance function 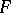 is a non-negative real-valued function on an
is a non-negative real-valued function on an  -dimensional Euclidean space such that
-dimensional Euclidean space such that 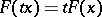 for
for  . If
. If  it is called symmetric, and if
it is called symmetric, and if 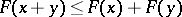 it is called convex. If
it is called convex. If 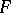 is convex, it is required moreover that
is convex, it is required moreover that 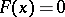 for
for 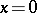 only.
only.
The critical determinant of a lattice is also called the lattice constant. An arrangement is also called a set lattice. The inequality (3) is usually called the Minkowski–Hlawka theorem.
In recent years the geometry of numbers has become more geometric in character. The covering and packing problems have been intensively studied, in particular the ball packing problem with its many relations to other areas such as coding, quantization of data, biology, metallurgy. Tilings have also attracted much interest; in particular Dirichlet–Voronoi (and Delone) tilings, which are of interest, for example, in geography, crystallography and computational geometry.
A tiling, or tesselation, of  is a family of sets (called tiles) such that their union covers
is a family of sets (called tiles) such that their union covers 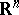 and their interiors are mutually disjoint. A Dirichlet–Voronoi tiling is a tiling with as tiles sets of the form
and their interiors are mutually disjoint. A Dirichlet–Voronoi tiling is a tiling with as tiles sets of the form
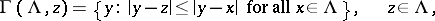 |
where  is a discrete point set in
is a discrete point set in 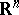 . Cf. [5].
. Cf. [5].
Other modern areas of the geometry of numbers are the theory of the zeta-function on lattices and (computational) reduction theory of quadratic forms and lattices.
References
| [a1] | P.M. Gruber, "Geometry of numbers" J. Tölke (ed.) J.M. Wills (ed.) , Contributions to geometry , Birkhäuser (1979) pp. 186–225 |
| [a2] | J.H. Conway, N.J.A. Sloane, "Sphere packing, lattices and groups" , Springer (1987) |
| [a3] | P. Erdös, P.M. Gruber, J. Hammer, "Lattice points" , Longman (1989) |
| [a4] | S.S. Ryskov, "The geometry of positive quadratic forms" , Amer. Math. Soc. (1982) |
| [a5] | A.B. Malyshev, Yu.G. Teterina, "Investigations in number theory" , 9 , Leningrad (1986) (In Russian) |
| [a6] | T.M. Thompson, "From error-correcting codes through sphere packing to simple groups" , Math. Assoc. Amer. (1983) |
| [a7] | G. Fejes Toth, "New results in the theory of packing and covering" P.M. Gruber (ed.) J.M. Wills (ed.) , Convexity and its applications , Birkhäuser (1983) pp. 318–359 |
| [a8] | G.F. Voronoi, "Collected works" , 1–3 , Kiev (1952) (In Russian) |
| [a9] | J.H.H. Chalk, "Algebraic lattices" P.M. Gruber (ed.) J.M. Wills (ed.) , Convexity and its applications , Birkhäuser (1983) pp. 97–110 |
| [a10] | H. Minkowski, "Gesammelte Abhandlungen" , 1–2 , Teubner (1911) |
Geometry of numbers. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Geometry_of_numbers&oldid=11821