Gauss law
The name often given to the normal distribution. It is connected with the role played by this distribution in Gauss' theory of errors (cf. Errors, theory of). The densities
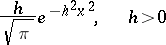 | (*) |
(these were originally known as "Gauss' law" ) first appeared in 1809 in C.F. Gauss' work Theoria motus corporum coelestium; the following principle was formulated in Book 2, Part 3, Section 177: "If some magnitude is determined on the strength of numerous direct observations, carried out under the same conditions and with the same accuracy, then the arithmetic mean of all the experimental values will be the most probable value …" [1]. This statement can be interpreted as follows: Let  be the true value of the observed magnitude, and let the probability density of obtaining the result
be the true value of the observed magnitude, and let the probability density of obtaining the result  be
be 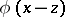 . Then, for any
. Then, for any  and any
and any  , the common density
, the common density 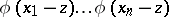 attains its maximum, as a function of
attains its maximum, as a function of  , if
, if
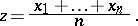 |
From this it can be easily deduced that the ratio 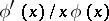 is independent of
is independent of  and that
and that  has the form (*). It should be noted that this principle was criticized on several occasions.
has the form (*). It should be noted that this principle was criticized on several occasions.
References
| [1] | C.F. Gauss, "Theoria motus corporum coellestium" , Werke , 7 , K. Gesellschaft Wissenschaft. Göttingen (1809) (English translation: C.H. Davis (ed.), Dover, 1963) |
| [2] | H. Poincaré, "Calcul des probabilités" , Gauthier-Villars (1912) |
Gauss law. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Gauss_law&oldid=11782