|
|
| Line 1: |
Line 1: |
| | + | <!--This article has been texified automatically. Since there was no Nroff source code for this article, |
| | + | the semi-automatic procedure described at https://encyclopediaofmath.org/wiki/User:Maximilian_Janisch/latexlist |
| | + | was used. |
| | + | If the TeX and formula formatting is correct, please remove this message and the {{TEX|semi-auto}} category. |
| | + | |
| | + | Out of 167 formulas, 149 were replaced by TEX code.--> |
| | + | |
| | + | {{TEX|semi-auto}}{{TEX|partial}} |
| | ''lattice-valued topology, point-set lattice-theoretic topology, poslat topology'' | | ''lattice-valued topology, point-set lattice-theoretic topology, poslat topology'' |
| | | | |
| | A branch of mathematics encompassing any sort of topology using lattice-valued subsets. The following description is based on the standardization of this discipline undertaken in [[#References|[a9]]], especially [[#References|[a10]]], [[#References|[a11]]]; much additional information is given in the references below. | | A branch of mathematics encompassing any sort of topology using lattice-valued subsets. The following description is based on the standardization of this discipline undertaken in [[#References|[a9]]], especially [[#References|[a10]]], [[#References|[a11]]]; much additional information is given in the references below. |
| | | | |
| − | Let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f1302901.png" /> be a set and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f1302902.png" /> any complete quasi-monoidal lattice (a cqml; i.e., <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f1302903.png" /> is a [[Complete lattice|complete lattice]] with bottom element <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f1302904.png" /> and top element <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f1302905.png" />, and the [[Tensor product|tensor product]] <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f1302906.png" /> is isotone in both arguments with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f1302907.png" />). Examples of complete quasi-monoidal lattices are: | + | Let $X$ be a set and $( L , \leq , \otimes )$ any complete quasi-monoidal lattice (a cqml; i.e., $( L , \leq )$ is a [[Complete lattice|complete lattice]] with bottom element $\perp$ and top element $\top$, and the [[Tensor product|tensor product]] $\otimes : L \times L \rightarrow L$ is isotone in both arguments with $\top \otimes \top = \top $). Examples of complete quasi-monoidal lattices are: |
| | | | |
| − | complete lattices with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f1302908.png" /> (binary meet); | + | complete lattices with $\otimes = \wedge$ (binary meet); |
| | | | |
| − | <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f1302909.png" /> with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029010.png" /> any of the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029012.png" />-norms <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029013.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029014.png" />, or <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029015.png" />; or
| + | $L = [ 0,1 ]$ with $\otimes$ any of the $t$-norms $T _ { \operatorname { min } } ( a , b ) = a \wedge b$, $T _ { \text { prod } } ( a , b ) = a \cdot b$, or $T _ { m } ( a , b ) = ( a + b - 1 ) \vee 0$; or |
| | | | |
| − | <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029016.png" /> with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029017.png" /> any of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029018.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029019.png" />, etc.
| + | $L = [ 0,1 ] \times [ 0,1 ]$ with $\otimes$ any of $T_{\text{min}} \times T_{\text{prod}}$, $T _ { \operatorname{prod} } \times T _ { m }$, etc. |
| | | | |
| − | <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029021.png" />-subsets of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029022.png" /> comprise the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029024.png" />-powerset <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029025.png" />, a complete quasi-monoidal lattice via the lifting of the structure of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029026.png" />. A subfamily <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029027.png" /> is an <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029029.png" />-topology on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029030.png" />, and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029031.png" /> is an <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029033.png" />-topological space, if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029034.png" /> is closed under <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029035.png" /> and arbitrary <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029036.png" /> and contains the constant mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029037.png" />. A function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029038.png" /> is an <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029040.png" />-fuzzy topology on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029041.png" />, and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029042.png" /> is an <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029044.png" />-fuzzy topological space, if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029045.png" /> satisfies (reading <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029046.png" /> as "for each" ):
| + | $L$-subsets of $X$ comprise the $L$-powerset $L ^ { X } = \{ a : X \rightarrow L , a \ \text {a function } \}$, a complete quasi-monoidal lattice via the lifting of the structure of $L$. A subfamily $\tau \subset L ^ { X }$ is an $L$-topology on $X$, and $( X , \tau )$ is an $L$-topological space, if $\tau$ is closed under $\otimes$ and arbitrary $\vee$ and contains the constant mapping $\underline{ \top } $. A function ${\cal T} : L ^ { X } \rightarrow L$ is an $L$-fuzzy topology on $X$, and $( X , \mathcal{T} )$ is an $L$-fuzzy topological space, if $\mathcal{T}$ satisfies (reading $\forall$ as "for each" ): |
| | | | |
| − | 1) <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029047.png" /> set <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029048.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029049.png" />, | + | 1) $\forall$ set $J$, $\forall \{ u_j : j \in J \} \subset L ^ { X }$, |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029050.png" /></td> </tr></table>
| + | \begin{equation*} \bigwedge _ { j \in J } {\cal T} ( u _ { j } ) \leq {\cal T} \left( \underset { j \in J } { \vee } u _ { j } \right). \end{equation*} |
| | | | |
| − | 2) <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029051.png" /> two-element set <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029052.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029053.png" />, | + | 2) $\forall$ two-element set $J$, $\forall \{ u_j : j \in J \} \subset L ^ { X }$, |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029054.png" /></td> </tr></table>
| + | \begin{equation*} \bigotimes _ { j \in J } \mathcal{T} ( u _ { j } ) \leq \mathcal{T} \left( \bigotimes _ { j \in J } u _ { j } \right) . \end{equation*} |
| | | | |
| − | 3) <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029055.png" />. | + | 3) $\mathcal{T} ( \underline { \top } ) = \top $. |
| | | | |
| − | The member <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029056.png" /> of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029057.png" /> is interpreted as the "degree of openness" of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029058.png" />. | + | The member $\mathcal{T} ( u )$ of $L$ is interpreted as the "degree of openness" of $u$. |
| | | | |
| − | Important examples of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029059.png" />-topological and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029060.png" />-fuzzy topological spaces can be found in [[#References|[a5]]], Chap. 11; [[#References|[a6]]], Kubiak's paper; [[#References|[a8]]]; [[#References|[a9]]], Chaps. 6, 8, 10; [[#References|[a9]]], Chap. 7, Sect. 2.15–2.16; [[#References|[a10]]], Sect. 7; [[#References|[a11]]], Sect. 7. | + | Important examples of $L$-topological and $L$-fuzzy topological spaces can be found in [[#References|[a5]]], Chap. 11; [[#References|[a6]]], Kubiak's paper; [[#References|[a8]]]; [[#References|[a9]]], Chaps. 6, 8, 10; [[#References|[a9]]], Chap. 7, Sect. 2.15–2.16; [[#References|[a10]]], Sect. 7; [[#References|[a11]]], Sect. 7. |
| | | | |
| − | For a complete quasi-monoidal lattice <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029061.png" /> and function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029062.png" />, one defines the powerset operators <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029063.png" /> (the image operator) and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029064.png" /> (the pre-image operator) by | + | For a complete quasi-monoidal lattice $L$ and function $f : X \rightarrow Y$, one defines the powerset operators $f _ { L } ^ { \rightarrow } : L ^ { X } \rightarrow L ^ { Y }$ (the image operator) and $f _ { L } ^ { \leftarrow } : L ^ { Y } \rightarrow L ^ { X }$ (the pre-image operator) by |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029065.png" /></td> </tr></table>
| + | \begin{equation*} f _ { L } ^ { \rightarrow } ( a ) ( y ) = \vee \{ a ( x ) : f ( x ) = y \}, \end{equation*} |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029066.png" /></td> </tr></table>
| + | \begin{equation*} f _ { L } ^ { \leftarrow } ( b ) = b \circ f. \end{equation*} |
| | | | |
| − | It is well-known that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029067.png" /> and that these operators generalize the traditional operators <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029068.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029069.png" />. Given <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029070.png" />-topological spaces <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029071.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029072.png" />, a mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029073.png" /> is <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029076.png" />-continuous from <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029077.png" /> to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029078.png" /> if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029079.png" />; and given <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029080.png" />-fuzzy topological spaces <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029081.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029082.png" />, a mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029083.png" /> is <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029086.png" />-fuzzy continuous from <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029087.png" /> to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029088.png" /> if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029089.png" /> on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029090.png" />. The [[Category|category]] <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029093.png" />-<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029094.png" /> comprises <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029095.png" />-topological spaces, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029096.png" />-continuous mappings, and the composition and identities from the category <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029097.png" /> (cf. also [[Sets, category of|Sets, category of]]); and the category <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290100.png" />-<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290101.png" /> comprises <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290102.png" />-fuzzy topological spaces, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290103.png" />-fuzzy continuous mappings, and the composition and identities from the category <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290104.png" />. It is a theorem that for all complete quasi-monoidal lattices <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290105.png" />, the categories <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290106.png" />-<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290107.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290108.png" />-<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290109.png" /> are topological categories over <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290110.png" />, in the sense of [[#References|[a3]]] and [[#References|[a11]]], Sect. 1, and hence topological constructs. | + | It is well-known that $f _ { L } ^ {\rightarrow} \dashv f _ { L } ^ { \leftarrow }$ and that these operators generalize the traditional operators $f^\rightarrow$ and $f ^ { \leftarrow }$. Given $L$-topological spaces $( X , \tau )$ and $( Y , \sigma )$, a mapping $f : X \rightarrow Y$ is $L$-continuous from $( X , \tau )$ to $( Y , \sigma )$ if $f | _ { \sigma } ^ { \leftarrow } : \tau \leftarrow \sigma$; and given $L$-fuzzy topological spaces $( X , \mathcal{T} )$ and $( Y , \mathcal{S} )$, a mapping $f : X \rightarrow Y$ is $L$-fuzzy continuous from $( X , \mathcal{T} )$ to $( Y , \mathcal{S} )$ if ${\cal T} \circ f ^ { \leftarrow } \geq \cal S$ on $L ^ { Y }$. The [[Category|category]] $L$-$\mathbf{TOP}$ comprises $L$-topological spaces, $L$-continuous mappings, and the composition and identities from the category <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f13029097.png"/> (cf. also [[Sets, category of|Sets, category of]]); and the category $L$-$\textbf{FTOP}$ comprises $L$-fuzzy topological spaces, $L$-fuzzy continuous mappings, and the composition and identities from the category <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290104.png"/>. It is a theorem that for all complete quasi-monoidal lattices $L$, the categories $L$-$\mathbf{TOP}$ and $L$-$\textbf{FTOP}$ are topological categories over <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290110.png"/>, in the sense of [[#References|[a3]]] and [[#References|[a11]]], Sect. 1, and hence topological constructs. |
| | | | |
| − | The above briefly describes "fixed-basis topology" — topology where the complete quasi-monoidal lattice <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290111.png" />, viewed as the lattice-theoretic base of powersets <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290112.png" /> and spaces <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290113.png" /> or <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290114.png" />, is fixed relative to the spaces and mappings of the category <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290115.png" />-<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290116.png" /> or <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290117.png" />-<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290118.png" />. "Variable-basis topology" permits the base to change within a category, so that each space has its own lattice-theoretic base. | + | The above briefly describes "fixed-basis topology" — topology where the complete quasi-monoidal lattice $L$, viewed as the lattice-theoretic base of powersets $L ^ { X }$ and spaces $( X , \tau )$ or $( X , \mathcal{T} )$, is fixed relative to the spaces and mappings of the category $L$-$\mathbf{TOP}$ or $L$-$\textbf{FTOP}$. "Variable-basis topology" permits the base to change within a category, so that each space has its own lattice-theoretic base. |
| | | | |
| − | To outline variable-basis topology, note that all complete quasi-monoidal lattices form a category, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290120.png" />, in which morphisms are mappings preserving <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290121.png" />, arbitrary <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290122.png" />, and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290123.png" />; and also note that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290124.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290125.png" /> embed into <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290126.png" />. One then considers <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290127.png" />, with objects the same as those of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290128.png" />, now called localic quasi-monoidal lattices, but morphisms reversed from those of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290129.png" />; and one notes that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290130.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290131.png" /> embed into <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290132.png" />. | + | To outline variable-basis topology, note that all complete quasi-monoidal lattices form a category, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290120.png"/>, in which morphisms are mappings preserving $\otimes$, arbitrary $\vee$, and $\top$; and also note that $\mathbf{FRM}$ and $\textbf{SFRM}$ embed into <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290126.png"/>. One then considers <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290127.png"/>, with objects the same as those of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290128.png"/>, now called localic quasi-monoidal lattices, but morphisms reversed from those of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290129.png"/>; and one notes that $\mathbf{LOC}$ and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290131.png"/> embed into <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290132.png"/>. |
| | | | |
| − | Now, let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290133.png" />. The category <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290134.png" />-<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290135.png" /> for variable-basis topology and the category <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290136.png" />-<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290137.png" /> for variable-basis fuzzy topology are both "concrete" categories over <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290138.png" /> as a "ground" or "base" category. For a <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290139.png" /> morphism <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290140.png" />, the pre-image operator <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290141.png" /> is defined by <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290142.png" />. An image operator <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290143.png" /> is also available which, if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290144.png" /> preserves arbitrary <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290145.png" />, satisfies <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290146.png" />; and if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290147.png" />, these operators reduce to their fixed-basis counterparts. | + | Now, let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290133.png"/>. The category $\mathbf{C}$-$\mathbf{TOP}$ for variable-basis topology and the category $\mathbf{C}$-$\textbf{FTOP}$ for variable-basis fuzzy topology are both "concrete" categories over <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290138.png"/> as a "ground" or "base" category. For a <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290139.png"/> morphism $( f , \phi ) : ( X , L ) \rightarrow ( Y , M )$, the pre-image operator $( f , \phi ) ^ { \leftarrow } : L ^ { X } \leftarrow M ^ { Y }$ is defined by $( f , \phi ) ^ { \leftarrow } ( b ) = \phi ^ { \text{op} } \circ b \circ f$. An image operator $( f , \phi ) ^ { \rightarrow }$ is also available which, if $\phi ^ { \operatorname{op} }$ preserves arbitrary $\wedge$, satisfies $( f , \phi )^{ \rightarrow} \dashv ( f , \phi )^{ \leftarrow}$; and if $L = M , \phi ^ { \operatorname{op} } = \operatorname{id} _ { L }$, these operators reduce to their fixed-basis counterparts. |
| | | | |
| − | Data for the category <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290148.png" />-<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290149.png" /> include: | + | Data for the category $\mathbf{C}$-$\mathbf{TOP}$ include: |
| | | | |
| − | objects are topological spaces <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290150.png" /> (cf. also [[Topological space|Topological space]]), where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290151.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290152.png" />; | + | objects are topological spaces $( X , L , \tau )$ (cf. also [[Topological space|Topological space]]), where $( X , L ) \in | \mathbf{SET} \times \mathbf{C}|$ and $( X , \tau ) \in | L \square \mathbf{TOP} |$; |
| | | | |
| | morphisms are continuous mappings | | morphisms are continuous mappings |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290153.png" /></td> </tr></table>
| + | \begin{equation*} ( f , \phi ) : ( X , L , \tau ) \rightarrow ( Y , M , \sigma ) \end{equation*} |
| | | | |
| − | (cf. also [[Continuous function|Continuous function]]), where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290154.png" /> is in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290155.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290156.png" />. | + | (cf. also [[Continuous function|Continuous function]]), where $( f , \phi ) : ( X , L ) \rightarrow ( Y , M )$ is in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290155.png"/> and $( f , \phi ) ^ { \leftarrow } | _ { \sigma } : \tau \leftarrow \sigma$. |
| | | | |
| − | Data for the category <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290157.png" />-<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290158.png" /> include: | + | Data for the category $\mathbf{C}$-$\textbf{FTOP}$ include: |
| | | | |
| − | objects are fuzzy topological spaces <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290159.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290160.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290161.png" />; | + | objects are fuzzy topological spaces $( X , L , \mathcal{T} )$, where $( X , L ) \in | \mathbf{SET} \times \mathbf{C}|$ and $( X , \mathcal{T} ) \in | L \square \mathbf{FTOP}|$; |
| | | | |
| − | morphisms are fuzzy continuous mappings <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290162.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290163.png" /> is in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290164.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290165.png" /> on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290166.png" />. | + | morphisms are fuzzy continuous mappings $( f , \phi ) : ( X , L , \mathcal{T} ) \rightarrow ( Y , M , \mathcal{S} )$, where $( f , \phi ) : ( X , L ) \rightarrow ( Y , M )$ is in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290164.png"/> and $\mathcal{T} \circ ( f , \phi ) ^ { \leftarrow } \geq \phi ^ { \operatorname{op} } \circ \mathcal{S}$ on $M ^ { Y }$. |
| | | | |
| − | In both categories, compositions and identities are those of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290167.png" />. | + | In both categories, compositions and identities are those of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290167.png"/>. |
| | | | |
| − | It is a theorem that for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290168.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290169.png" />-<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290170.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290171.png" />-<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290172.png" /> are topological over the ground <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290173.png" /> in the sense of [[#References|[a3]]] and [[#References|[a11]]], Sect. 1. Further, these frameworks unify all the fixed-basis categories for topology given above and hence unify all important examples (referenced above) over different lattice-theoretic bases (e.g. two fuzzy real lines <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290174.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290175.png" />). Moreover, all purely lattice-theoretic or point-free approaches to topology — locales, topological molecular lattices, uniform lattices, etc. (see [[#References|[a1]]], [[#References|[a2]]], [[#References|[a8]]]) — categorically embed into <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290176.png" />-<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290177.png" /> or <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290178.png" />-<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290179.png" /> (for appropriate <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290180.png" />) as subcategories of singleton spaces; e.g. <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290181.png" /> embeds as singleton spaces into <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290182.png" />-<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290183.png" />. Thus, the variable-basis approach categorically unifies topology and fuzzy topology as a discipline. | + | It is a theorem that for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290168.png"/>, $\mathbf{C}$-$\mathbf{TOP}$ and $\mathbf{C}$-$\textbf{FTOP}$ are topological over the ground <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/f/f130/f130290/f130290173.png"/> in the sense of [[#References|[a3]]] and [[#References|[a11]]], Sect. 1. Further, these frameworks unify all the fixed-basis categories for topology given above and hence unify all important examples (referenced above) over different lattice-theoretic bases (e.g. two fuzzy real lines $\mathbf{R} ( L )$ and $\mathbf{R} ( M )$). Moreover, all purely lattice-theoretic or point-free approaches to topology — locales, topological molecular lattices, uniform lattices, etc. (see [[#References|[a1]]], [[#References|[a2]]], [[#References|[a8]]]) — categorically embed into $\mathbf{C}$-$\mathbf{TOP}$ or $\mathbf{C}$-$\textbf{FTOP}$ (for appropriate $\mathbf{C}$) as subcategories of singleton spaces; e.g. $\mathbf{LOC}$ embeds as singleton spaces into $\mathbf{LOC}$-$\mathbf{TOP}$. Thus, the variable-basis approach categorically unifies topology and fuzzy topology as a discipline. |
| | | | |
| | ====References==== | | ====References==== |
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> P.T. Johnstone, "Stone spaces" , Cambridge Univ. Press (1982)</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> G.-J. Wang, "Theory of L-fuzzy topological spaces" , Shanxi Normal Univ. Publ. House (1988) (In Chinese)</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> J. Adamek, H. Herrlich, G.E. Strecker, "Abstract and concrete categories" , Wiley (1990)</TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> U. (ed.) Höhle, "Mathematical aspects of fuzzy set theory" ''Fuzzy Sets and Syst.'' , '''40''' : 2 (1991) (Special Memorial Volume–Second Issue)</TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> "Applications of category theory to fuzzy subsets" S.E. Rodabaugh (ed.) E.P. Klement (ed.) U. Höhle (ed.) , Kluwer Acad. Publ. (1992)</TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> U. Höhle, S.E. Rodabaugh, A. (eds.) Šostak, "Special issue on fuzzy topology" ''Fuzzy Sets and Syst.'' , '''73''' : 1 (1995)</TD></TR><TR><TD valign="top">[a7]</TD> <TD valign="top"> W. (ed.) Kotzé, "Special issue" ''Quaestiones Math.'' , '''20''' : 3 (1997)</TD></TR><TR><TD valign="top">[a8]</TD> <TD valign="top"> Liu Ying–Ming, Luo Mao–Kang, "Fuzzy topology" , World Sci. (1997)</TD></TR><TR><TD valign="top">[a9]</TD> <TD valign="top"> "Mathematics of fuzzy sets: Logic, topology, and measure theory" U. Höhle (ed.) S.E. Rodabaugh (ed.) , ''The Handbooks of Fuzzy Sets'' , '''3''' , Kluwer Acad. Publ. (1999)</TD></TR><TR><TD valign="top">[a10]</TD> <TD valign="top"> U. Höhle, A. Šostak, "Axiomatic foundations of fixed-basis fuzzy topology" U. Höhle (ed.) S.E. Rodabaugh (ed.) , ''Mathematics of Fuzzy Sets: Logic, Topology, and Measure Theory'' , ''The Handbooks of Fuzzy Sets'' , '''3''' , Kluwer Acad. Publ. (1999) pp. 123–272</TD></TR><TR><TD valign="top">[a11]</TD> <TD valign="top"> S.E. Rodabaugh, "Categorical foundations of variable-basis fuzzy topology" U. Höhle (ed.) S.E. Rodabaugh (ed.) , ''Mathematics of Fuzzy Sets: Logic, Topology, and Measure Theory'' , ''The Handbooks of Fuzzy Sets'' , '''3''' , Kluwer Acad. Publ. (1999) pp. 273–388</TD></TR></table> | + | <table><tr><td valign="top">[a1]</td> <td valign="top"> P.T. Johnstone, "Stone spaces" , Cambridge Univ. Press (1982)</td></tr><tr><td valign="top">[a2]</td> <td valign="top"> G.-J. Wang, "Theory of L-fuzzy topological spaces" , Shanxi Normal Univ. Publ. House (1988) (In Chinese)</td></tr><tr><td valign="top">[a3]</td> <td valign="top"> J. Adamek, H. Herrlich, G.E. Strecker, "Abstract and concrete categories" , Wiley (1990)</td></tr><tr><td valign="top">[a4]</td> <td valign="top"> U. (ed.) Höhle, "Mathematical aspects of fuzzy set theory" ''Fuzzy Sets and Syst.'' , '''40''' : 2 (1991) (Special Memorial Volume–Second Issue)</td></tr><tr><td valign="top">[a5]</td> <td valign="top"> "Applications of category theory to fuzzy subsets" S.E. Rodabaugh (ed.) E.P. Klement (ed.) U. Höhle (ed.) , Kluwer Acad. Publ. (1992)</td></tr><tr><td valign="top">[a6]</td> <td valign="top"> U. Höhle, S.E. Rodabaugh, A. (eds.) Šostak, "Special issue on fuzzy topology" ''Fuzzy Sets and Syst.'' , '''73''' : 1 (1995)</td></tr><tr><td valign="top">[a7]</td> <td valign="top"> W. (ed.) Kotzé, "Special issue" ''Quaestiones Math.'' , '''20''' : 3 (1997)</td></tr><tr><td valign="top">[a8]</td> <td valign="top"> Liu Ying–Ming, Luo Mao–Kang, "Fuzzy topology" , World Sci. (1997)</td></tr><tr><td valign="top">[a9]</td> <td valign="top"> "Mathematics of fuzzy sets: Logic, topology, and measure theory" U. Höhle (ed.) S.E. Rodabaugh (ed.) , ''The Handbooks of Fuzzy Sets'' , '''3''' , Kluwer Acad. Publ. (1999)</td></tr><tr><td valign="top">[a10]</td> <td valign="top"> U. Höhle, A. Šostak, "Axiomatic foundations of fixed-basis fuzzy topology" U. Höhle (ed.) S.E. Rodabaugh (ed.) , ''Mathematics of Fuzzy Sets: Logic, Topology, and Measure Theory'' , ''The Handbooks of Fuzzy Sets'' , '''3''' , Kluwer Acad. Publ. (1999) pp. 123–272</td></tr><tr><td valign="top">[a11]</td> <td valign="top"> S.E. Rodabaugh, "Categorical foundations of variable-basis fuzzy topology" U. Höhle (ed.) S.E. Rodabaugh (ed.) , ''Mathematics of Fuzzy Sets: Logic, Topology, and Measure Theory'' , ''The Handbooks of Fuzzy Sets'' , '''3''' , Kluwer Acad. Publ. (1999) pp. 273–388</td></tr></table> |
lattice-valued topology, point-set lattice-theoretic topology, poslat topology
A branch of mathematics encompassing any sort of topology using lattice-valued subsets. The following description is based on the standardization of this discipline undertaken in [a9], especially [a10], [a11]; much additional information is given in the references below.
Let $X$ be a set and $( L , \leq , \otimes )$ any complete quasi-monoidal lattice (a cqml; i.e., $( L , \leq )$ is a complete lattice with bottom element $\perp$ and top element $\top$, and the tensor product $\otimes : L \times L \rightarrow L$ is isotone in both arguments with $\top \otimes \top = \top $). Examples of complete quasi-monoidal lattices are:
complete lattices with $\otimes = \wedge$ (binary meet);
$L = [ 0,1 ]$ with $\otimes$ any of the $t$-norms $T _ { \operatorname { min } } ( a , b ) = a \wedge b$, $T _ { \text { prod } } ( a , b ) = a \cdot b$, or $T _ { m } ( a , b ) = ( a + b - 1 ) \vee 0$; or
$L = [ 0,1 ] \times [ 0,1 ]$ with $\otimes$ any of $T_{\text{min}} \times T_{\text{prod}}$, $T _ { \operatorname{prod} } \times T _ { m }$, etc.
$L$-subsets of $X$ comprise the $L$-powerset $L ^ { X } = \{ a : X \rightarrow L , a \ \text {a function } \}$, a complete quasi-monoidal lattice via the lifting of the structure of $L$. A subfamily $\tau \subset L ^ { X }$ is an $L$-topology on $X$, and $( X , \tau )$ is an $L$-topological space, if $\tau$ is closed under $\otimes$ and arbitrary $\vee$ and contains the constant mapping $\underline{ \top } $. A function ${\cal T} : L ^ { X } \rightarrow L$ is an $L$-fuzzy topology on $X$, and $( X , \mathcal{T} )$ is an $L$-fuzzy topological space, if $\mathcal{T}$ satisfies (reading $\forall$ as "for each" ):
1) $\forall$ set $J$, $\forall \{ u_j : j \in J \} \subset L ^ { X }$,
\begin{equation*} \bigwedge _ { j \in J } {\cal T} ( u _ { j } ) \leq {\cal T} \left( \underset { j \in J } { \vee } u _ { j } \right). \end{equation*}
2) $\forall$ two-element set $J$, $\forall \{ u_j : j \in J \} \subset L ^ { X }$,
\begin{equation*} \bigotimes _ { j \in J } \mathcal{T} ( u _ { j } ) \leq \mathcal{T} \left( \bigotimes _ { j \in J } u _ { j } \right) . \end{equation*}
3) $\mathcal{T} ( \underline { \top } ) = \top $.
The member $\mathcal{T} ( u )$ of $L$ is interpreted as the "degree of openness" of $u$.
Important examples of $L$-topological and $L$-fuzzy topological spaces can be found in [a5], Chap. 11; [a6], Kubiak's paper; [a8]; [a9], Chaps. 6, 8, 10; [a9], Chap. 7, Sect. 2.15–2.16; [a10], Sect. 7; [a11], Sect. 7.
For a complete quasi-monoidal lattice $L$ and function $f : X \rightarrow Y$, one defines the powerset operators $f _ { L } ^ { \rightarrow } : L ^ { X } \rightarrow L ^ { Y }$ (the image operator) and $f _ { L } ^ { \leftarrow } : L ^ { Y } \rightarrow L ^ { X }$ (the pre-image operator) by
\begin{equation*} f _ { L } ^ { \rightarrow } ( a ) ( y ) = \vee \{ a ( x ) : f ( x ) = y \}, \end{equation*}
\begin{equation*} f _ { L } ^ { \leftarrow } ( b ) = b \circ f. \end{equation*}
It is well-known that $f _ { L } ^ {\rightarrow} \dashv f _ { L } ^ { \leftarrow }$ and that these operators generalize the traditional operators $f^\rightarrow$ and $f ^ { \leftarrow }$. Given $L$-topological spaces $( X , \tau )$ and $( Y , \sigma )$, a mapping $f : X \rightarrow Y$ is $L$-continuous from $( X , \tau )$ to $( Y , \sigma )$ if $f | _ { \sigma } ^ { \leftarrow } : \tau \leftarrow \sigma$; and given $L$-fuzzy topological spaces $( X , \mathcal{T} )$ and $( Y , \mathcal{S} )$, a mapping $f : X \rightarrow Y$ is $L$-fuzzy continuous from $( X , \mathcal{T} )$ to $( Y , \mathcal{S} )$ if ${\cal T} \circ f ^ { \leftarrow } \geq \cal S$ on $L ^ { Y }$. The category $L$-$\mathbf{TOP}$ comprises $L$-topological spaces, $L$-continuous mappings, and the composition and identities from the category 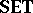 (cf. also Sets, category of); and the category $L$-$\textbf{FTOP}$ comprises $L$-fuzzy topological spaces, $L$-fuzzy continuous mappings, and the composition and identities from the category
(cf. also Sets, category of); and the category $L$-$\textbf{FTOP}$ comprises $L$-fuzzy topological spaces, $L$-fuzzy continuous mappings, and the composition and identities from the category 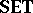 . It is a theorem that for all complete quasi-monoidal lattices $L$, the categories $L$-$\mathbf{TOP}$ and $L$-$\textbf{FTOP}$ are topological categories over
. It is a theorem that for all complete quasi-monoidal lattices $L$, the categories $L$-$\mathbf{TOP}$ and $L$-$\textbf{FTOP}$ are topological categories over 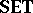 , in the sense of [a3] and [a11], Sect. 1, and hence topological constructs.
, in the sense of [a3] and [a11], Sect. 1, and hence topological constructs.
The above briefly describes "fixed-basis topology" — topology where the complete quasi-monoidal lattice $L$, viewed as the lattice-theoretic base of powersets $L ^ { X }$ and spaces $( X , \tau )$ or $( X , \mathcal{T} )$, is fixed relative to the spaces and mappings of the category $L$-$\mathbf{TOP}$ or $L$-$\textbf{FTOP}$. "Variable-basis topology" permits the base to change within a category, so that each space has its own lattice-theoretic base.
To outline variable-basis topology, note that all complete quasi-monoidal lattices form a category,  , in which morphisms are mappings preserving $\otimes$, arbitrary $\vee$, and $\top$; and also note that $\mathbf{FRM}$ and $\textbf{SFRM}$ embed into
, in which morphisms are mappings preserving $\otimes$, arbitrary $\vee$, and $\top$; and also note that $\mathbf{FRM}$ and $\textbf{SFRM}$ embed into 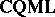 . One then considers
. One then considers 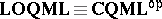 , with objects the same as those of
, with objects the same as those of  , now called localic quasi-monoidal lattices, but morphisms reversed from those of
, now called localic quasi-monoidal lattices, but morphisms reversed from those of 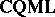 ; and one notes that $\mathbf{LOC}$ and
; and one notes that $\mathbf{LOC}$ and 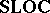 embed into
embed into 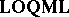 .
.
Now, let 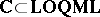 . The category $\mathbf{C}$-$\mathbf{TOP}$ for variable-basis topology and the category $\mathbf{C}$-$\textbf{FTOP}$ for variable-basis fuzzy topology are both "concrete" categories over
. The category $\mathbf{C}$-$\mathbf{TOP}$ for variable-basis topology and the category $\mathbf{C}$-$\textbf{FTOP}$ for variable-basis fuzzy topology are both "concrete" categories over 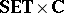 as a "ground" or "base" category. For a
as a "ground" or "base" category. For a 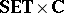 morphism $( f , \phi ) : ( X , L ) \rightarrow ( Y , M )$, the pre-image operator $( f , \phi ) ^ { \leftarrow } : L ^ { X } \leftarrow M ^ { Y }$ is defined by $( f , \phi ) ^ { \leftarrow } ( b ) = \phi ^ { \text{op} } \circ b \circ f$. An image operator $( f , \phi ) ^ { \rightarrow }$ is also available which, if $\phi ^ { \operatorname{op} }$ preserves arbitrary $\wedge$, satisfies $( f , \phi )^{ \rightarrow} \dashv ( f , \phi )^{ \leftarrow}$; and if $L = M , \phi ^ { \operatorname{op} } = \operatorname{id} _ { L }$, these operators reduce to their fixed-basis counterparts.
morphism $( f , \phi ) : ( X , L ) \rightarrow ( Y , M )$, the pre-image operator $( f , \phi ) ^ { \leftarrow } : L ^ { X } \leftarrow M ^ { Y }$ is defined by $( f , \phi ) ^ { \leftarrow } ( b ) = \phi ^ { \text{op} } \circ b \circ f$. An image operator $( f , \phi ) ^ { \rightarrow }$ is also available which, if $\phi ^ { \operatorname{op} }$ preserves arbitrary $\wedge$, satisfies $( f , \phi )^{ \rightarrow} \dashv ( f , \phi )^{ \leftarrow}$; and if $L = M , \phi ^ { \operatorname{op} } = \operatorname{id} _ { L }$, these operators reduce to their fixed-basis counterparts.
Data for the category $\mathbf{C}$-$\mathbf{TOP}$ include:
objects are topological spaces $( X , L , \tau )$ (cf. also Topological space), where $( X , L ) \in | \mathbf{SET} \times \mathbf{C}|$ and $( X , \tau ) \in | L \square \mathbf{TOP} |$;
morphisms are continuous mappings
\begin{equation*} ( f , \phi ) : ( X , L , \tau ) \rightarrow ( Y , M , \sigma ) \end{equation*}
(cf. also Continuous function), where $( f , \phi ) : ( X , L ) \rightarrow ( Y , M )$ is in  and $( f , \phi ) ^ { \leftarrow } | _ { \sigma } : \tau \leftarrow \sigma$.
and $( f , \phi ) ^ { \leftarrow } | _ { \sigma } : \tau \leftarrow \sigma$.
Data for the category $\mathbf{C}$-$\textbf{FTOP}$ include:
objects are fuzzy topological spaces $( X , L , \mathcal{T} )$, where $( X , L ) \in | \mathbf{SET} \times \mathbf{C}|$ and $( X , \mathcal{T} ) \in | L \square \mathbf{FTOP}|$;
morphisms are fuzzy continuous mappings $( f , \phi ) : ( X , L , \mathcal{T} ) \rightarrow ( Y , M , \mathcal{S} )$, where $( f , \phi ) : ( X , L ) \rightarrow ( Y , M )$ is in 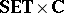 and $\mathcal{T} \circ ( f , \phi ) ^ { \leftarrow } \geq \phi ^ { \operatorname{op} } \circ \mathcal{S}$ on $M ^ { Y }$.
and $\mathcal{T} \circ ( f , \phi ) ^ { \leftarrow } \geq \phi ^ { \operatorname{op} } \circ \mathcal{S}$ on $M ^ { Y }$.
In both categories, compositions and identities are those of 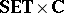 .
.
It is a theorem that for all 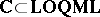 , $\mathbf{C}$-$\mathbf{TOP}$ and $\mathbf{C}$-$\textbf{FTOP}$ are topological over the ground
, $\mathbf{C}$-$\mathbf{TOP}$ and $\mathbf{C}$-$\textbf{FTOP}$ are topological over the ground 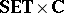 in the sense of [a3] and [a11], Sect. 1. Further, these frameworks unify all the fixed-basis categories for topology given above and hence unify all important examples (referenced above) over different lattice-theoretic bases (e.g. two fuzzy real lines $\mathbf{R} ( L )$ and $\mathbf{R} ( M )$). Moreover, all purely lattice-theoretic or point-free approaches to topology — locales, topological molecular lattices, uniform lattices, etc. (see [a1], [a2], [a8]) — categorically embed into $\mathbf{C}$-$\mathbf{TOP}$ or $\mathbf{C}$-$\textbf{FTOP}$ (for appropriate $\mathbf{C}$) as subcategories of singleton spaces; e.g. $\mathbf{LOC}$ embeds as singleton spaces into $\mathbf{LOC}$-$\mathbf{TOP}$. Thus, the variable-basis approach categorically unifies topology and fuzzy topology as a discipline.
in the sense of [a3] and [a11], Sect. 1. Further, these frameworks unify all the fixed-basis categories for topology given above and hence unify all important examples (referenced above) over different lattice-theoretic bases (e.g. two fuzzy real lines $\mathbf{R} ( L )$ and $\mathbf{R} ( M )$). Moreover, all purely lattice-theoretic or point-free approaches to topology — locales, topological molecular lattices, uniform lattices, etc. (see [a1], [a2], [a8]) — categorically embed into $\mathbf{C}$-$\mathbf{TOP}$ or $\mathbf{C}$-$\textbf{FTOP}$ (for appropriate $\mathbf{C}$) as subcategories of singleton spaces; e.g. $\mathbf{LOC}$ embeds as singleton spaces into $\mathbf{LOC}$-$\mathbf{TOP}$. Thus, the variable-basis approach categorically unifies topology and fuzzy topology as a discipline.
References
| [a1] | P.T. Johnstone, "Stone spaces" , Cambridge Univ. Press (1982) |
| [a2] | G.-J. Wang, "Theory of L-fuzzy topological spaces" , Shanxi Normal Univ. Publ. House (1988) (In Chinese) |
| [a3] | J. Adamek, H. Herrlich, G.E. Strecker, "Abstract and concrete categories" , Wiley (1990) |
| [a4] | U. (ed.) Höhle, "Mathematical aspects of fuzzy set theory" Fuzzy Sets and Syst. , 40 : 2 (1991) (Special Memorial Volume–Second Issue) |
| [a5] | "Applications of category theory to fuzzy subsets" S.E. Rodabaugh (ed.) E.P. Klement (ed.) U. Höhle (ed.) , Kluwer Acad. Publ. (1992) |
| [a6] | U. Höhle, S.E. Rodabaugh, A. (eds.) Šostak, "Special issue on fuzzy topology" Fuzzy Sets and Syst. , 73 : 1 (1995) |
| [a7] | W. (ed.) Kotzé, "Special issue" Quaestiones Math. , 20 : 3 (1997) |
| [a8] | Liu Ying–Ming, Luo Mao–Kang, "Fuzzy topology" , World Sci. (1997) |
| [a9] | "Mathematics of fuzzy sets: Logic, topology, and measure theory" U. Höhle (ed.) S.E. Rodabaugh (ed.) , The Handbooks of Fuzzy Sets , 3 , Kluwer Acad. Publ. (1999) |
| [a10] | U. Höhle, A. Šostak, "Axiomatic foundations of fixed-basis fuzzy topology" U. Höhle (ed.) S.E. Rodabaugh (ed.) , Mathematics of Fuzzy Sets: Logic, Topology, and Measure Theory , The Handbooks of Fuzzy Sets , 3 , Kluwer Acad. Publ. (1999) pp. 123–272 |
| [a11] | S.E. Rodabaugh, "Categorical foundations of variable-basis fuzzy topology" U. Höhle (ed.) S.E. Rodabaugh (ed.) , Mathematics of Fuzzy Sets: Logic, Topology, and Measure Theory , The Handbooks of Fuzzy Sets , 3 , Kluwer Acad. Publ. (1999) pp. 273–388 |
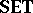 (cf. also Sets, category of); and the category $L$-$\textbf{FTOP}$ comprises $L$-fuzzy topological spaces, $L$-fuzzy continuous mappings, and the composition and identities from the category
(cf. also Sets, category of); and the category $L$-$\textbf{FTOP}$ comprises $L$-fuzzy topological spaces, $L$-fuzzy continuous mappings, and the composition and identities from the category 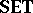 . It is a theorem that for all complete quasi-monoidal lattices $L$, the categories $L$-$\mathbf{TOP}$ and $L$-$\textbf{FTOP}$ are topological categories over
. It is a theorem that for all complete quasi-monoidal lattices $L$, the categories $L$-$\mathbf{TOP}$ and $L$-$\textbf{FTOP}$ are topological categories over 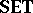 , in the sense of [a3] and [a11], Sect. 1, and hence topological constructs.
, in the sense of [a3] and [a11], Sect. 1, and hence topological constructs.
 , in which morphisms are mappings preserving $\otimes$, arbitrary $\vee$, and $\top$; and also note that $\mathbf{FRM}$ and $\textbf{SFRM}$ embed into
, in which morphisms are mappings preserving $\otimes$, arbitrary $\vee$, and $\top$; and also note that $\mathbf{FRM}$ and $\textbf{SFRM}$ embed into 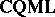 . One then considers
. One then considers 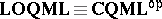 , with objects the same as those of
, with objects the same as those of  , now called localic quasi-monoidal lattices, but morphisms reversed from those of
, now called localic quasi-monoidal lattices, but morphisms reversed from those of 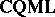 ; and one notes that $\mathbf{LOC}$ and
; and one notes that $\mathbf{LOC}$ and 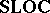 embed into
embed into 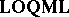 .
.
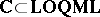 . The category $\mathbf{C}$-$\mathbf{TOP}$ for variable-basis topology and the category $\mathbf{C}$-$\textbf{FTOP}$ for variable-basis fuzzy topology are both "concrete" categories over
. The category $\mathbf{C}$-$\mathbf{TOP}$ for variable-basis topology and the category $\mathbf{C}$-$\textbf{FTOP}$ for variable-basis fuzzy topology are both "concrete" categories over 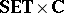 as a "ground" or "base" category. For a
as a "ground" or "base" category. For a 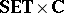 morphism $( f , \phi ) : ( X , L ) \rightarrow ( Y , M )$, the pre-image operator $( f , \phi ) ^ { \leftarrow } : L ^ { X } \leftarrow M ^ { Y }$ is defined by $( f , \phi ) ^ { \leftarrow } ( b ) = \phi ^ { \text{op} } \circ b \circ f$. An image operator $( f , \phi ) ^ { \rightarrow }$ is also available which, if $\phi ^ { \operatorname{op} }$ preserves arbitrary $\wedge$, satisfies $( f , \phi )^{ \rightarrow} \dashv ( f , \phi )^{ \leftarrow}$; and if $L = M , \phi ^ { \operatorname{op} } = \operatorname{id} _ { L }$, these operators reduce to their fixed-basis counterparts.
morphism $( f , \phi ) : ( X , L ) \rightarrow ( Y , M )$, the pre-image operator $( f , \phi ) ^ { \leftarrow } : L ^ { X } \leftarrow M ^ { Y }$ is defined by $( f , \phi ) ^ { \leftarrow } ( b ) = \phi ^ { \text{op} } \circ b \circ f$. An image operator $( f , \phi ) ^ { \rightarrow }$ is also available which, if $\phi ^ { \operatorname{op} }$ preserves arbitrary $\wedge$, satisfies $( f , \phi )^{ \rightarrow} \dashv ( f , \phi )^{ \leftarrow}$; and if $L = M , \phi ^ { \operatorname{op} } = \operatorname{id} _ { L }$, these operators reduce to their fixed-basis counterparts.
 and $( f , \phi ) ^ { \leftarrow } | _ { \sigma } : \tau \leftarrow \sigma$.
and $( f , \phi ) ^ { \leftarrow } | _ { \sigma } : \tau \leftarrow \sigma$.
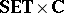 and $\mathcal{T} \circ ( f , \phi ) ^ { \leftarrow } \geq \phi ^ { \operatorname{op} } \circ \mathcal{S}$ on $M ^ { Y }$.
and $\mathcal{T} \circ ( f , \phi ) ^ { \leftarrow } \geq \phi ^ { \operatorname{op} } \circ \mathcal{S}$ on $M ^ { Y }$.
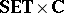 .
.
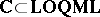 , $\mathbf{C}$-$\mathbf{TOP}$ and $\mathbf{C}$-$\textbf{FTOP}$ are topological over the ground
, $\mathbf{C}$-$\mathbf{TOP}$ and $\mathbf{C}$-$\textbf{FTOP}$ are topological over the ground 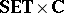 in the sense of [a3] and [a11], Sect. 1. Further, these frameworks unify all the fixed-basis categories for topology given above and hence unify all important examples (referenced above) over different lattice-theoretic bases (e.g. two fuzzy real lines $\mathbf{R} ( L )$ and $\mathbf{R} ( M )$). Moreover, all purely lattice-theoretic or point-free approaches to topology — locales, topological molecular lattices, uniform lattices, etc. (see [a1], [a2], [a8]) — categorically embed into $\mathbf{C}$-$\mathbf{TOP}$ or $\mathbf{C}$-$\textbf{FTOP}$ (for appropriate $\mathbf{C}$) as subcategories of singleton spaces; e.g. $\mathbf{LOC}$ embeds as singleton spaces into $\mathbf{LOC}$-$\mathbf{TOP}$. Thus, the variable-basis approach categorically unifies topology and fuzzy topology as a discipline.
in the sense of [a3] and [a11], Sect. 1. Further, these frameworks unify all the fixed-basis categories for topology given above and hence unify all important examples (referenced above) over different lattice-theoretic bases (e.g. two fuzzy real lines $\mathbf{R} ( L )$ and $\mathbf{R} ( M )$). Moreover, all purely lattice-theoretic or point-free approaches to topology — locales, topological molecular lattices, uniform lattices, etc. (see [a1], [a2], [a8]) — categorically embed into $\mathbf{C}$-$\mathbf{TOP}$ or $\mathbf{C}$-$\textbf{FTOP}$ (for appropriate $\mathbf{C}$) as subcategories of singleton spaces; e.g. $\mathbf{LOC}$ embeds as singleton spaces into $\mathbf{LOC}$-$\mathbf{TOP}$. Thus, the variable-basis approach categorically unifies topology and fuzzy topology as a discipline.