Fourier-Bessel series
From Encyclopedia of Mathematics
The expansion of a function  in a series
in a series
 | (*) |
where 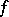 is a function given on the interval
is a function given on the interval 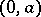 ,
, 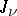 is the Bessel function of order
is the Bessel function of order 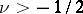 (cf. Bessel functions), and the
(cf. Bessel functions), and the 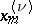 are the positive zeros of
are the positive zeros of  taken in increasing order; the coefficients
taken in increasing order; the coefficients  have the following values:
have the following values:
 |
If 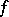 is a piecewise-continuous function given on an interval
is a piecewise-continuous function given on an interval 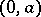 and if the integral
and if the integral
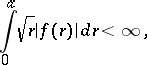 |
then the Fourier–Bessel series converges and its sum is equal to  at each interior point
at each interior point  of
of 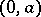 at which
at which  locally has bounded variation.
locally has bounded variation.
How to Cite This Entry:
Fourier-Bessel series. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Fourier-Bessel_series&oldid=19087
Fourier-Bessel series. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Fourier-Bessel_series&oldid=19087
This article was adapted from an original article by L.N. Karmazina (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article