Equivalence of categories
An extension of the concept of an isomorphism of categories brought about, first of all, by the presence of classes of isomorphic objects.
Two categories  and
and  are called equivalent if there are one-place covariant functors
are called equivalent if there are one-place covariant functors  and
and  such that the product
such that the product  is naturally equivalent to the identity functor
is naturally equivalent to the identity functor  and the product
and the product  to the functor
to the functor  ; in other words, the categories
; in other words, the categories  and
and 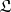 are equivalent if there are functors
are equivalent if there are functors  and
and  "almost" inverse to one another. Two categories are equivalent if and only if their skeletons are isomorphic (see Skeleton of a category).
"almost" inverse to one another. Two categories are equivalent if and only if their skeletons are isomorphic (see Skeleton of a category).
Pontryagin's duality theorem establishes the equivalence of the category of Abelian groups and the category that is dual to that of topological Abelian groups; the category of Boolean algebras is equivalent to the category that is dual to that of Boolean spaces; the category of binary relations over the category of sets is equivalent to the Kleisli category for the triple defined by the functor of taking the set of subsets.
Comments
Cf. Triple for that notion, and the editorial comments to Category for the notion of a Kleisli category of a triple.
References
| [a1] | B. Mitchell, "Theory of categories" , Acad. Press (1965) |
Equivalence of categories. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Equivalence_of_categories&oldid=12063