Difference between revisions of "Elimination theory"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
||
| Line 51: | Line 51: | ||
etc. This makes it possible to describe explicitly the result of eliminating <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e035/e035380/e03538072.png" /> from (5). | etc. This makes it possible to describe explicitly the result of eliminating <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e035/e035380/e03538072.png" /> from (5). | ||
| − | The elimination of unknowns from arbitrary systems of algebraic equations can be done successively (i.e. first one eliminates one unknown, then the second, etc.) using the | + | The elimination of unknowns from arbitrary systems of algebraic equations can be done successively (i.e. first one eliminates one unknown, then the second, etc.) using the "elementary transformations" described below. Since after eliminating a single unknown one obtains a constructible set instead of an algebraic set, the problem of projecting an arbitrary constructible set in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e035/e035380/e03538073.png" /> on the coordinate space of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e035/e035380/e03538074.png" /> must be considered. |
Every constructible set <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e035/e035380/e03538075.png" /> can be represented as the union of sets of solutions of a collection of systems of algebraic equations and inequalities. (An algebraic inequality is of the form <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e035/e035380/e03538076.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e035/e035380/e03538077.png" /> is a polynomial.) An elementary transformation of such a collection is a transformation of one of the following kinds: in one of the systems one adds to one of the equations another equation, multiplied by a polynomial; in a system containing an inequality <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e035/e035380/e03538078.png" />, one of the equations is multiplied by <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e035/e035380/e03538079.png" />; in one of the systems, one adds to one of the inequalities an equation, multiplied by a polynomial; one of the systems is divided into two systems, determined by adding the equation <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e035/e035380/e03538080.png" />, respectively the inequality <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e035/e035380/e03538081.png" />, to it (<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e035/e035380/e03538082.png" /> is an arbitrary polynomial); or, in one of the systems, two inequalities are replaced by their product. | Every constructible set <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e035/e035380/e03538075.png" /> can be represented as the union of sets of solutions of a collection of systems of algebraic equations and inequalities. (An algebraic inequality is of the form <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e035/e035380/e03538076.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e035/e035380/e03538077.png" /> is a polynomial.) An elementary transformation of such a collection is a transformation of one of the following kinds: in one of the systems one adds to one of the equations another equation, multiplied by a polynomial; in a system containing an inequality <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e035/e035380/e03538078.png" />, one of the equations is multiplied by <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e035/e035380/e03538079.png" />; in one of the systems, one adds to one of the inequalities an equation, multiplied by a polynomial; one of the systems is divided into two systems, determined by adding the equation <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e035/e035380/e03538080.png" />, respectively the inequality <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e035/e035380/e03538081.png" />, to it (<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e035/e035380/e03538082.png" /> is an arbitrary polynomial); or, in one of the systems, two inequalities are replaced by their product. | ||
| Line 60: | Line 60: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> W.V.D. Hodge, D. Pedoe, "Methods of algebraic geometry" , '''1''' , Cambridge Univ. Press (1947) {{MR|0028055}} {{ZBL|0796.14002}} {{ZBL|0796.14003}} {{ZBL|0796.14001}} {{ZBL|0157.27502}} {{ZBL|0157.27501}} {{ZBL|0055.38705}} {{ZBL|0048.14502}} </TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> B.L. van der Waerden, "Algebra" , '''1–2''' , Springer (1967–1971) (Translated from German) {{MR|1541390}} {{ZBL|1032.00002}} {{ZBL|1032.00001}} {{ZBL|0903.01009}} {{ZBL|0781.12003}} {{ZBL|0781.12002}} {{ZBL|0724.12002}} {{ZBL|0724.12001}} {{ZBL|0569.01001}} {{ZBL|0534.01001}} {{ZBL|0997.00502}} {{ZBL|0997.00501}} {{ZBL|0316.22001}} {{ZBL|0297.01014}} {{ZBL|0221.12001}} {{ZBL|0192.33002}} {{ZBL|0137.25403}} {{ZBL|0136.24505}} {{ZBL|0087.25903}} {{ZBL|0192.33001}} {{ZBL|0067.00502}} </TD></TR></table> |
| Line 68: | Line 68: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> D. Mumford, "Algebraic geometry" , '''1. Complex projective varieties''' , Springer (1976) {{MR|0453732}} {{ZBL|0356.14002}} </TD></TR></table> |
Revision as of 21:51, 30 March 2012
The theory of eliminating unknowns from systems of algebraic equations. More precisely, suppose one is given a system of equations
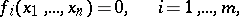 | (1) |
where 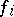 are polynomials with coefficients in a given field
are polynomials with coefficients in a given field 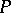 . The problem of eliminating
. The problem of eliminating  from (1) (the inhomogeneous problem in elimination theory) can be formulated as follows: Find the projection of the set of solutions of (1) on the coordinate space of
from (1) (the inhomogeneous problem in elimination theory) can be formulated as follows: Find the projection of the set of solutions of (1) on the coordinate space of 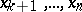 . If all equations are homogeneous with respect to the set of variables
. If all equations are homogeneous with respect to the set of variables  , one also consider the homogeneous problem in elimination theory (the inhomogeneous problem is trivial in this case): Find the projection on the coordinate space of
, one also consider the homogeneous problem in elimination theory (the inhomogeneous problem is trivial in this case): Find the projection on the coordinate space of 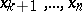 of the set of those solutions of (1) in which not all unknowns
of the set of those solutions of (1) in which not all unknowns  are zero.
are zero.
The inhomogeneous problem can also be regarded as the problem of finding conditions on the coefficients of the system of algebraic equations ensuring that this system is compatible; the homogeneous problem is then that of finding conditions on the coefficients of a system of homogeneous algebraic equations ensuring that this system has a non-zero solution.
The fundamental result of elimination theory is that if  is an algebraically closed field, then the solution of the homogeneous problem is an algebraic set, i.e. the set of solutions of a system of algebraic equations, while the solution of the inhomogeneous problem is a constructible set in the sense of algebraic geometry, i.e. a finite union of sets of the form
is an algebraically closed field, then the solution of the homogeneous problem is an algebraic set, i.e. the set of solutions of a system of algebraic equations, while the solution of the inhomogeneous problem is a constructible set in the sense of algebraic geometry, i.e. a finite union of sets of the form 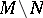 , where
, where 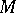 and
and  are algebraic sets (cf. also Constructible subset). An explicit solution to problems in elimination theory is known in some of the simplest cases.
are algebraic sets (cf. also Constructible subset). An explicit solution to problems in elimination theory is known in some of the simplest cases.
1) Consider a system of equations that are linear and homogeneous in  , i.e. of the form
, i.e. of the form
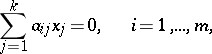 | (2) |
where  are polynomials in
are polynomials in 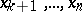 . For given values of
. For given values of 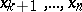 the system (2) has a non-zero solution if and only if the rank of the matrix
the system (2) has a non-zero solution if and only if the rank of the matrix 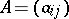 is less than
is less than  (cf. Linear equation). The solution of the homogeneous problem now is the set in the coordinate space of
(cf. Linear equation). The solution of the homogeneous problem now is the set in the coordinate space of 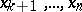 described by the vanishing of all minors of rank
described by the vanishing of all minors of rank 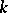 of
of 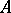 .
.
2) Consider a system of equations that are linear in  , i.e. of the form
, i.e. of the form
 | (3) |
where 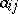 ,
, 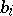 are polynomials in
are polynomials in 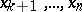 . Let
. Let  be the matrix obtained from
be the matrix obtained from  by addition of the column
by addition of the column 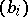 . For given values of
. For given values of 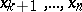 the system (3) is compatible if and only if the rank of
the system (3) is compatible if and only if the rank of 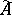 equals the rank of
equals the rank of  . Hence, the result of eliminating
. Hence, the result of eliminating  from (3) is
from (3) is
 |
where  is the set of points
is the set of points 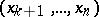 at which the rank of
at which the rank of  is at most
is at most  , while
, while 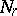 is the set of points at which the rank of
is the set of points at which the rank of 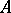 is less than
is less than  (both
(both 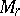 and
and 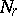 are algebraic sets).
are algebraic sets).
3) Let  , let
, let 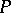 be an algebraically closed field and consider a system of two equations that are homogeneous in
be an algebraically closed field and consider a system of two equations that are homogeneous in  ,
,  :
:
 | (4) |
where 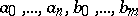 are polynomials in
are polynomials in  . For given values of
. For given values of  the system (4) has a non-zero solution if and only if the resultant
the system (4) has a non-zero solution if and only if the resultant 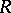 vanishes. Here
vanishes. Here 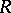 is given by
is given by
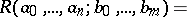 |
 |
(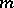 rows of
rows of  ;
;  rows of
rows of  ). This also gives a solution of the homogeneous problem in the case considered.
). This also gives a solution of the homogeneous problem in the case considered.
4) Let  , let
, let 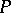 be an algebraically closed field and consider the system of two equations
be an algebraically closed field and consider the system of two equations
 | (5) |
where 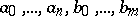 are polynomials in
are polynomials in  . For given values of
. For given values of  at which either
at which either  or
or 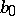 does not vanish, (5) is a compatible system if and only if
does not vanish, (5) is a compatible system if and only if
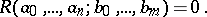 |
If 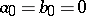 , one must consider
, one must consider
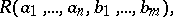 |
etc. This makes it possible to describe explicitly the result of eliminating 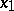 from (5).
from (5).
The elimination of unknowns from arbitrary systems of algebraic equations can be done successively (i.e. first one eliminates one unknown, then the second, etc.) using the "elementary transformations" described below. Since after eliminating a single unknown one obtains a constructible set instead of an algebraic set, the problem of projecting an arbitrary constructible set in 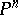 on the coordinate space of
on the coordinate space of  must be considered.
must be considered.
Every constructible set 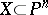 can be represented as the union of sets of solutions of a collection of systems of algebraic equations and inequalities. (An algebraic inequality is of the form
can be represented as the union of sets of solutions of a collection of systems of algebraic equations and inequalities. (An algebraic inequality is of the form 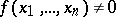 , where
, where 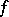 is a polynomial.) An elementary transformation of such a collection is a transformation of one of the following kinds: in one of the systems one adds to one of the equations another equation, multiplied by a polynomial; in a system containing an inequality
is a polynomial.) An elementary transformation of such a collection is a transformation of one of the following kinds: in one of the systems one adds to one of the equations another equation, multiplied by a polynomial; in a system containing an inequality 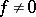 , one of the equations is multiplied by
, one of the equations is multiplied by 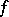 ; in one of the systems, one adds to one of the inequalities an equation, multiplied by a polynomial; one of the systems is divided into two systems, determined by adding the equation
; in one of the systems, one adds to one of the inequalities an equation, multiplied by a polynomial; one of the systems is divided into two systems, determined by adding the equation 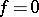 , respectively the inequality
, respectively the inequality 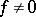 , to it (
, to it (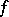 is an arbitrary polynomial); or, in one of the systems, two inequalities are replaced by their product.
is an arbitrary polynomial); or, in one of the systems, two inequalities are replaced by their product.
The elementary transformations do not change the union of the sets of solutions of the systems in the original collection. By applying elementary transformations, any collection of algebraic equations and inequalities in unknowns  can be reduced to a form in which each of the systems contains at most one equation or inequality containing
can be reduced to a form in which each of the systems contains at most one equation or inequality containing 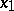 , and, moreover, together with this equation,
, and, moreover, together with this equation,  , or inequality,
, or inequality,  , the inequality
, the inequality 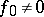 is contained in it, where
is contained in it, where  , a polynomial in
, a polynomial in  , is the leading coefficient of the expansion of
, is the leading coefficient of the expansion of 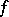 in powers of
in powers of 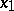 . If
. If 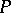 is an algebraically closed field, then by disregarding in the systems the equations and inequalities in
is an algebraically closed field, then by disregarding in the systems the equations and inequalities in 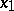 thus obtained, one obtains a collection of systems of algebraic and inequalities in
thus obtained, one obtains a collection of systems of algebraic and inequalities in  . This gives the projection of the set
. This gives the projection of the set 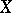 on the coordinate space of
on the coordinate space of  .
.
Sequential elimination of unknowns by elementary transformations allows one to reduce, in principle, the solution of any system of algebraic equations in  unknowns to the solution of a number of algebraic equations in one unknown.
unknowns to the solution of a number of algebraic equations in one unknown.
References
| [1] | W.V.D. Hodge, D. Pedoe, "Methods of algebraic geometry" , 1 , Cambridge Univ. Press (1947) MR0028055 Zbl 0796.14002 Zbl 0796.14003 Zbl 0796.14001 Zbl 0157.27502 Zbl 0157.27501 Zbl 0055.38705 Zbl 0048.14502 |
| [2] | B.L. van der Waerden, "Algebra" , 1–2 , Springer (1967–1971) (Translated from German) MR1541390 Zbl 1032.00002 Zbl 1032.00001 Zbl 0903.01009 Zbl 0781.12003 Zbl 0781.12002 Zbl 0724.12002 Zbl 0724.12001 Zbl 0569.01001 Zbl 0534.01001 Zbl 0997.00502 Zbl 0997.00501 Zbl 0316.22001 Zbl 0297.01014 Zbl 0221.12001 Zbl 0192.33002 Zbl 0137.25403 Zbl 0136.24505 Zbl 0087.25903 Zbl 0192.33001 Zbl 0067.00502 |
Comments
For a non-constructive approach to elimination theory see [a1], § 2C.
References
| [a1] | D. Mumford, "Algebraic geometry" , 1. Complex projective varieties , Springer (1976) MR0453732 Zbl 0356.14002 |
Elimination theory. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Elimination_theory&oldid=15892