Eigen vector
of an operator  acting on a vector space
acting on a vector space  over a field
over a field 
A non-zero vector  which is mapped by
which is mapped by  to a vector proportional to it, that is
to a vector proportional to it, that is
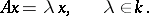 |
The coefficient  is called an eigen value of
is called an eigen value of  .
.
If  is a linear operator, then the set
is a linear operator, then the set  of all eigen vectors corresponding to an eigen value
of all eigen vectors corresponding to an eigen value  , together with the zero vector, forms a linear subspace. It is called the eigen space of
, together with the zero vector, forms a linear subspace. It is called the eigen space of  corresponding to the eigen value
corresponding to the eigen value 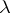 and it coincides with the kernel
and it coincides with the kernel 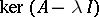 of the operator
of the operator 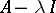 (that is, with the set of vectors mapped to 0 by this operator). If
(that is, with the set of vectors mapped to 0 by this operator). If  is a topological vector space and
is a topological vector space and 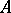 a continuous operator, then
a continuous operator, then  is closed for any
is closed for any 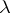 . Eigen spaces need not, in general, be finite-dimensional, but if
. Eigen spaces need not, in general, be finite-dimensional, but if  is completely continuous (compact), then
is completely continuous (compact), then 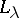 is finite-dimensional for any non-zero
is finite-dimensional for any non-zero  .
.
In fact, the existence of an eigen vector for operators on infinite-dimensional spaces is a fairly rare occurrence, although operators of special classes which are important in applications (such as integral and differential operators) often have large families of eigen vectors.
Generalizations of the concepts of an eigen vector and an eigen space are those of a root vector and a root subspace. In the case of normal operators on a Hilbert space (in particular, self-adjoint or unitary operators), every root vector is an eigen vector and the eigen spaces corresponding to different eigen values are mutually orthogonal.
References
| [1] | K. Yosida, "Functional analysis" , Springer (1980) pp. Chapt. 8, §1 |
| [2] | L.A. [L.A. Lyusternik] Lusternik, "Elements of functional analysis" , Hindushtan Publ. Comp. (1974) (Translated from Russian) |
| [3] | L.V. Kantorovich, G.P. Akilov, "Functional analysis" , Pergamon (1982) pp. Chapt. 13, §3 (Translated from Russian) |
Comments
It is also quite common to write eigenvector, eigenspace, etc., i.e. not two words but one.
Eigen vectors are sometimes also called characteristic vectors, eigen elements, eigen functions, or proper vectors; root vectors are usually called principal vectors in the Western literature. [a1] and [a2] are good general Western references.
Various notions of generalized eigen vectors (or improper eigen functions) exist in the literature; e.g. see [a3] and [a4] for generalized eigen vectors and eigen function expansions in the context of rigged Hilbert spaces (Gel'fand triplets; see also Rigged Hilbert space).
References
| [a1] | N. Dunford, J.T. Schwartz, "Linear operators. Spectral theory" , 2 , Interscience (1963) pp. Chapt. 10, §3 |
| [a2] | A.E. Taylor, D.C. Lay, "Introduction to functional analysis" , Wiley (1980) pp. Chapt. 5 |
| [a3] | I.M. Gel'fand, N.Ya. Vilenkin, "Generalized functions. Applications of harmonic analysis" , 4 , Acad. Press (1964) pp. Chapt. 1, §4 (Translated from Russian) |
| [a4] | Yu.M. [Yu.M. Berezanskii] Berezanskiy, "Expansion in eigenfunctions of selfadjoint operators" , Amer. Math. Soc. (1968) pp. Chapt. 5, §2 (Translated from Russian) |
| [a5] | S. Lang, "Linear algebra" , Addison-Wesley (1973) |
Eigen vector. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Eigen_vector&oldid=13516