Double-layer potential

An expression of the type

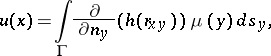(1)

where 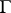is the boundary of an arbitrary bounded 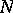-dimensional domain 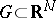, , and is the exterior normal to the boundary 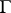of at a point ; is the potential density, which is a function defined on 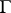; is a fundamental solution of the Laplace equation:

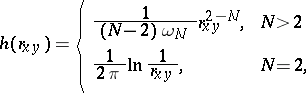(2)

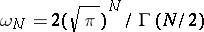is the area of the surface of the 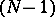-dimensional unit sphere, and 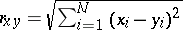is the distance between two points and 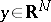. The boundary 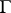is of class 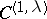; it is a Lyapunov surface or a Lyapunov arc (cf. Lyapunov surfaces and curves).

Expression (1) may be interpreted as the potential produced by dipoles located on 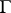, the direction of which at any point 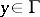coincides with that of the exterior normal , while its intensity is equal to .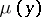

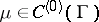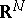If , then is defined on (in particular, on 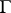) and displays the following properties.

1) The function has derivatives of all orders 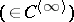everywhere in and satisfies the Laplace equation, and the derivatives with respect to the coordinates of a point may be computed by differentiation of the integrand.

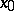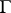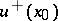2) On passing through the boundary the function undergoes a break. Let be an arbitrary point on ; let and 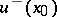be the interior and exterior boundary values; then 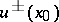exist and are equal to

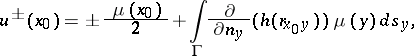(3)

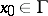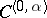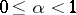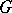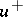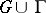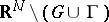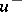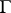and the integral in formula (3) as a function of belongs to for any ; also, the function equal to in and to on is continuous on , while the function equal to in and equal to on is continuous in .

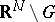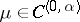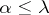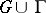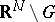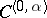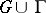3) If the density and if , then , extended as in (2) on or , is of class in or in .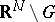

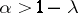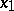4) If , and and 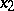are two points on the normal issuing from a point 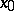and lying symmetric about 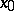, then

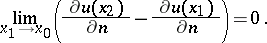(4)

In particular, if one of the derivatives , 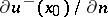exists, then the other derivative also exists and 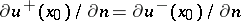. This is also true if 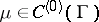and .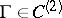

The above properties can be generalized in various ways. The density 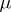may belong to 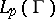, . Then 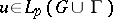, 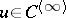outside 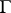and it satisfies the Laplace equation, formula (3) and (4) apply for almost-all and the integral in (3) belongs to .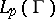

The properties of double-layer potentials, regarded as integrals with respect to an arbitrary measure defined on 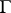, have also been studied:

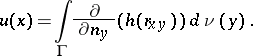Here, too, 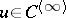outside 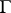and it satisfies the Laplace equation. Formulas (3) and (4) apply for almost-all 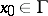with respect to the Lebesgue measure after 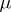has been replaced by the density 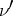. In definition (1) the fundamental solution of the Laplace equation may be replaced by an arbitrary Lewy function for a general elliptic operator of the second order with variable coefficients, while 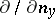is replaced by the derivative with respect to the conormal. The properties listed above remain valid [2].

The double-layer potential plays an important role in solving boundary value problems of elliptic equations. The representation of the solution of the (first) boundary value problem is sought as a double-layer potential with unknown density 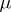and an application of property (2) leads to a Fredholm equation of the second kind on 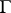in order to determine the function 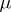[1], [2]. In solving boundary value problems for parabolic equations use is made of the concept of the thermal double layer potential, i.e. of an integral of the type

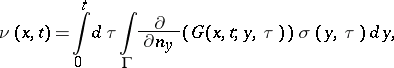where is a fundamental solution of the thermal conductance (or heat) equation in an 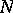-dimensional space:

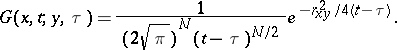Here, 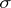is the potential density. The function and its generalization to the case of an arbitrary parabolic equation of the second order have properties which are similar to those described above for [3], [4], [5].

References

[1]	N.M. Günter, "Potential theory and its applications to basic problems of mathematical physics" , F. Ungar (1967) (Translated from French)
[2]	C. Miranda, "Partial differential equations of elliptic type" , Springer (1970) (Translated from Italian)
[3]	A.N. [A.N. Tikhonov] Tichonoff, A.A. Samarskii, "Differentialgleichungen der mathematischen Physik" , Deutsch. Verlag Wissenschaft. (1959) (Translated from Russian)
[4]	V.I. Smirnov, "A course of higher mathematics" , 4 , Addison-Wesley (1964) (Translated from Russian)
[5]	A. Friedman, "Partial differential equations of parabolic type" , Prentice-Hall (1964)

Comments

See [a1] for an introduction to double-layer potentials for more general open sets in .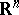

References

[a1]	J. Král, "Integral operators in potential theory" , Lect. notes in math. , 823 , Springer (1980)