Distribution of prime numbers
A branch of number theory studying distribution laws of prime numbers among natural numbers. The central problem is that of finding the best asymptotic, as 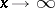 , expression for the function
, expression for the function  , which is the number of prime numbers not exceeding
, which is the number of prime numbers not exceeding  , and for the function
, and for the function  , which is the number of prime numbers not exceeding
, which is the number of prime numbers not exceeding  in the arithmetic progression
in the arithmetic progression 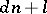 ,
, 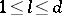 ,
, 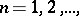 for values of
for values of 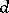 increasing along with
increasing along with  .
.
The fundamental theorem of arithmetic: Any natural number 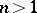 is either a prime number or a unique (up to permutation of factors) product
is either a prime number or a unique (up to permutation of factors) product
 |
(the so-called canonical representation of  ), where
), where  are different prime numbers and
are different prime numbers and  are natural numbers. Thus, the prime numbers form a multiplicative basis for the set of natural numbers. This, though, says nothing about the value of
are natural numbers. Thus, the prime numbers form a multiplicative basis for the set of natural numbers. This, though, says nothing about the value of 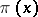 .
.
A method used for finding the prime numbers between 1 and  is that of the sieve of Eratosthenes, known since the 3rd century B.C. (cf. Eratosthenes, sieve of). The sieve of Eratosthenes is the simplest procedure for obtaining the sequence of prime numbers. But the analytic formula of the sieve,
is that of the sieve of Eratosthenes, known since the 3rd century B.C. (cf. Eratosthenes, sieve of). The sieve of Eratosthenes is the simplest procedure for obtaining the sequence of prime numbers. But the analytic formula of the sieve,
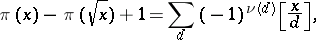 |
where 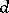 takes the values of the divisors of the product of all prime numbers
takes the values of the divisors of the product of all prime numbers 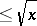 ;
; 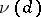 is the number of prime divisors of
is the number of prime divisors of 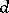 and
and  is the integer part of
is the integer part of  , is not very well suited for studying
, is not very well suited for studying  as
as 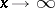 .
.
The examination of the sequence of prime numbers between 1 and  :
:
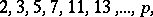 | (1) |
shows that they get ever sparser on the average as  increases. There are arbitrarily long segments of the natural series containing no prime number. For instance, the
increases. There are arbitrarily long segments of the natural series containing no prime number. For instance, the 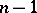 numbers of the form
numbers of the form 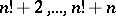 are all composite for any
are all composite for any 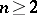 . At the same time, prime numbers that differ by 2, like 8004119 and 8004121, are encountered in (1) (twins). The behaviour of
. At the same time, prime numbers that differ by 2, like 8004119 and 8004121, are encountered in (1) (twins). The behaviour of 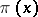 as
as 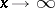 is one of the most difficult and inspiring problems of number theory.
is one of the most difficult and inspiring problems of number theory.
The first result about 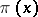 is Euclid's theorem:
is Euclid's theorem: 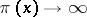 as
as 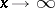 . L. Euler (1737, 1749, see [1]) introduced the function
. L. Euler (1737, 1749, see [1]) introduced the function
 | (2) |
and showed that
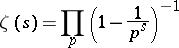 | (3) |
for 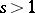 . Here the series extends over all natural numbers, and the product over all prime numbers. The identity (3) and its generalizations play a fundamental role in the theory of the distribution of prime numbers. Based on the identity, Euler proved that the series
. Here the series extends over all natural numbers, and the product over all prime numbers. The identity (3) and its generalizations play a fundamental role in the theory of the distribution of prime numbers. Based on the identity, Euler proved that the series 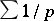 and the product
and the product 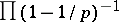 diverge over the sequence of prime numbers. This is another proof that the set of prime numbers is infinite. Moreover, Euler proved that
diverge over the sequence of prime numbers. This is another proof that the set of prime numbers is infinite. Moreover, Euler proved that
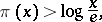 |
which shows that there are "many" prime numbers, although almost-all natural numbers are composite in the sense that
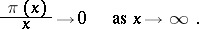 |
A significant success was subsequently obtained by P.L. Chebyshev (1851–1852, see [2]). He proved that
1) for any 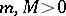 there are sequences
there are sequences 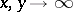 such that
such that
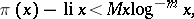 |
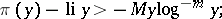 |
2) if the quotient 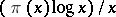 converges as
converges as 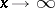 , then the limit is equal to 1.
, then the limit is equal to 1.
This solved for the first time the question of the existence of a simple function
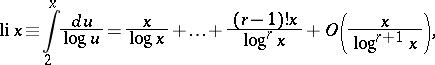 |
which approximates 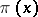 optimally.
optimally.
Then Chebyshev established the true order of growth of 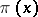 , that is, the existence of constants
, that is, the existence of constants  and
and 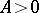 such that
such that
 | (4) |
notably, 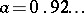 ,
, 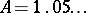 for
for 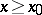 . He also proved that for any
. He also proved that for any 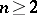 the interval
the interval 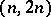 contains at least one prime number (Bertrand's postulate). The proof of (4) is based on Chebyshev's identity
contains at least one prime number (Bertrand's postulate). The proof of (4) is based on Chebyshev's identity
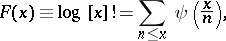 | (5) |
where the function 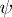 , introduced by Chebyshev, is defined by the sum
, introduced by Chebyshev, is defined by the sum
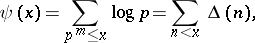 |
taken over all powers  ,
, 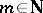 , of prime numbers
, of prime numbers 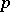 . Namely, if
. Namely, if 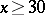 , the following combination of
, the following combination of 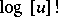 :
:
 |
owing to (5), gives the identity
 |
which implies
 |
By virtue of Stirling's asymptotic formula for 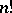 this implies an analogue of (4) for
this implies an analogue of (4) for  from which (4) is obtained by partial summation. Chebyshev's function
from which (4) is obtained by partial summation. Chebyshev's function 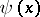 proved to be more convenient for studying the distribution of prime numbers than
proved to be more convenient for studying the distribution of prime numbers than 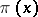 , since the best approximation for it is the very argument
, since the best approximation for it is the very argument  . Therefore
. Therefore 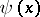 is usually considered first and then the corresponding result for
is usually considered first and then the corresponding result for  is obtained by partial summation.
is obtained by partial summation.
Riemann's principle.
In 1859–1860 B. Riemann [3] considered the function 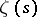 , introduced by Euler for
, introduced by Euler for 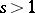 , as a function of a complex variable
, as a function of a complex variable 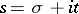 , where
, where 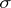 and
and  are real variables, defined by (2) for
are real variables, defined by (2) for  (see Zeta-function) and found that this function is extremely important in the theory of the distribution of prime numbers. In particular, he found an expression for the difference
(see Zeta-function) and found that this function is extremely important in the theory of the distribution of prime numbers. In particular, he found an expression for the difference 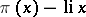 in terms of
in terms of  and those zeros of
and those zeros of 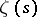 which belong to the strip
which belong to the strip 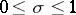 ; they are called the non-trivial zeros of
; they are called the non-trivial zeros of 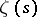 .
.
Instead of Riemann's formula, its simpler finite analogue for 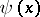 , proved (along with Riemann's formula) by H. (von) Mangoldt in 1895, is often used. Namely, for
, proved (along with Riemann's formula) by H. (von) Mangoldt in 1895, is often used. Namely, for 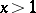 :
:
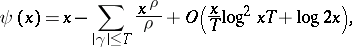 | (6) |
where 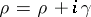 runs through the non-trivial zeros of
runs through the non-trivial zeros of 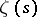 and
and 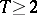 is arbitrary.
is arbitrary.
Since
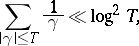 |
(6) shows that the difference  is primarily defined by
is primarily defined by 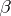 (the real part of the right-most zeros
(the real part of the right-most zeros 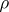 ). In particular, the following asymptotic expressions are valid for
). In particular, the following asymptotic expressions are valid for 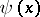 and
and 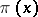 :
:
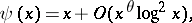 |
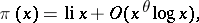 |
provided that  to the right of a vertical
to the right of a vertical 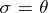 ,
, 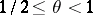 . Conversely, it follows from these relations that
. Conversely, it follows from these relations that 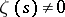 if
if 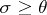 . If the Riemann hypothesis holds, that is, if all non-trivial zeros of
. If the Riemann hypothesis holds, that is, if all non-trivial zeros of 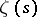 lie on the line
lie on the line  , then the asymptotic distribution law for prime numbers must have the form
, then the asymptotic distribution law for prime numbers must have the form
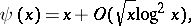 |
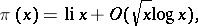 |
and these relations cannot be substantially strengthened. The latter means that there are sequences  such that
such that
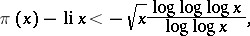 |
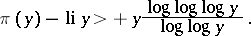 |
Thus, by Riemann's principle, the problem about the asymptotic expressions for 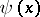 and
and 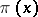 reduces to that about bounds for the real part of the non-trivial zeros of
reduces to that about bounds for the real part of the non-trivial zeros of 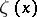 . Till now (1983), however, no constant
. Till now (1983), however, no constant 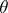 ,
, 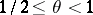 , has been found with the condition
, has been found with the condition 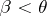 . The desired bound for
. The desired bound for 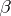 turns out to be connected with the imaginary part
turns out to be connected with the imaginary part 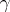 of zeros
of zeros 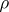 in such a way that the line
in such a way that the line 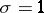 is its asymptote.
is its asymptote.
The methods of Hadamard and de la Vallée-Poussin.
In its simplest form
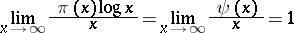 |
the asymptotic distribution law for prime numbers was independently obtained in 1896 by J. Hadamard and Ch.J. de la Vallée-Poussin, who proved that 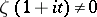 , that is, there is no zero of
, that is, there is no zero of 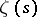 on the line
on the line  . In 1899 de la Vallée-Poussin showed that
. In 1899 de la Vallée-Poussin showed that  in the domain
in the domain  . It was thereby proved that
. It was thereby proved that
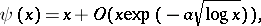 |
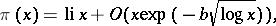 |
where 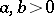 are constants.
are constants.
Further extensions of the region containing no zeros of 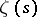 were also obtained (see [4]–[12]).
were also obtained (see [4]–[12]).
The method of Weyl–Littlewood.
There is a certain connection between the growth of the modulus of  and its zeros near the line
and its zeros near the line 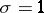 . In particular, if
. In particular, if  for
for 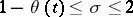 , where
, where  and
and 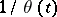 are positive non-decreasing functions of
are positive non-decreasing functions of 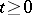 such that
such that 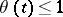 ,
, 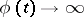 as
as 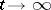 and
and 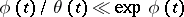 , then there is a constant
, then there is a constant 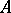 such that
such that  in the region
in the region
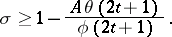 |
The estimate for 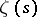 is here obtained with the help of the partial sums of the series (2). This reduces the problem to the estimation of trigonometric sums of the form
is here obtained with the help of the partial sums of the series (2). This reduces the problem to the estimation of trigonometric sums of the form
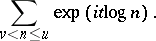 |
By estimating such sums by the Weyl method, J.E. Littlewood showed in 1921 that 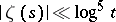 if
if  and
and 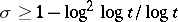 , so that
, so that 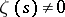 in the region
in the region
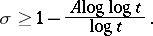 |
It follows that
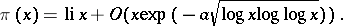 |
The method of Vinogradov.
A further advance in the estimation of  and
and 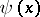 is connected with development by I.M. Vinogradov of a new and substantially more powerful method for the estimation of trigonometric sums (see Vinogradov method). Using this method, he showed in 1938 that
is connected with development by I.M. Vinogradov of a new and substantially more powerful method for the estimation of trigonometric sums (see Vinogradov method). Using this method, he showed in 1938 that  if
if
 |
and consequently that
 |
In 1958 Vinogradov and others (see [6]–[11]) showed that 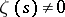 if
if
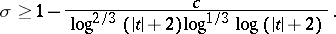 |
So far (1983) this is the best result concerning bounds on the non-trivial zeros of 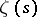 , to which the following best result on the distribution of prime numbers corresponds:
, to which the following best result on the distribution of prime numbers corresponds:
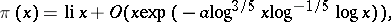 |
 |
The asymptotics for the  -th prime number,
-th prime number, 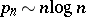 , follow from the asymptotics of
, follow from the asymptotics of 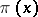 . It was also shown (see [21]) that
. It was also shown (see [21]) that 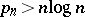 for all
for all 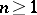 and that for
and that for 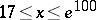 ,
,  ,
,
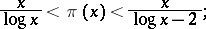 |
and for 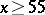 ,
,
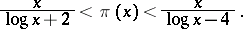 |
Elementary methods.
This is the name for methods for studying the asymptotic distribution of prime numbers that are not based on Riemann's principle (zeros of the zeta-function) and, in general, on any principles from the theory of functions of a complex variable whatsoever. Such a method was first discovered by A. Selberg [16] and P. Erdös [17]. It is based on the elementary formula of Selberg
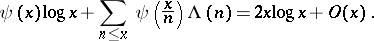 | (7) |
A further problem consists in obtaining asymptotics for 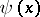 from the averaged asymptotics for
from the averaged asymptotics for 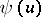 given by (7). It may be done in different ways but all of them employ the fact that
given by (7). It may be done in different ways but all of them employ the fact that  slowly oscillates. In 1962 E. Bombieri and E. Wirsing proved that
slowly oscillates. In 1962 E. Bombieri and E. Wirsing proved that
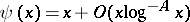 |
for any fixed 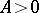 . In 1970, H.G. Diamond and J. Steinig [19] substantially perfected the principles and techniques of estimation by means of an elementary method. They proved that for
. In 1970, H.G. Diamond and J. Steinig [19] substantially perfected the principles and techniques of estimation by means of an elementary method. They proved that for 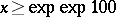 ,
,
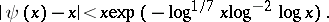 |
Finally, in 1973 A.F. Lavrik and A.Sh. Sobirov [20] showed that an elementary method enables one to prove the following theorem: For 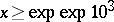 ,
,
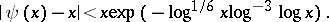 |
This result is so far the best achievement of the elementary method as regards the distribution of prime numbers. Although it is somewhat weaker than the result obtained by the analytic method, in principle they are close.
The difference between prime numbers.
There are many questions in the distribution of prime numbers that are concerned with differences between prime numbers. Standing out among them are questions about the behaviour of the difference 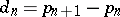 between adjacent prime numbers; the problem on the number of prime twins or, more generally, pairs of prime numbers that differ by
between adjacent prime numbers; the problem on the number of prime twins or, more generally, pairs of prime numbers that differ by 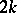 or, in the most general form, the problem about the number of systems
or, in the most general form, the problem about the number of systems 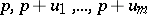 of
of 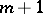 prime numbers within the segment
prime numbers within the segment 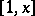 .
.
Using Riemann's hypothesis it was proved that  , and heuristic considerations suggest that probably
, and heuristic considerations suggest that probably 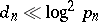 . By 1983, the best estimate
. By 1983, the best estimate 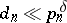 , where
, where 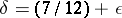 ,
, 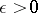 , was obtained by M.N. Huxley (1973) using the method of the large sieve. As for pairs of prime numbers whose difference is equal to 2 (twins) or to
, was obtained by M.N. Huxley (1973) using the method of the large sieve. As for pairs of prime numbers whose difference is equal to 2 (twins) or to 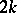 ,
, 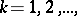 it is still (1983) unknown whether the number of such pairs is finite or not. Let
it is still (1983) unknown whether the number of such pairs is finite or not. Let 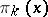 be the number of prime numbers that do not exceed
be the number of prime numbers that do not exceed  and differ by
and differ by 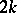 . In 1919 V. Brun found a sieve method (see Brun sieve) that permitted one to obtain the expected upper bound for
. In 1919 V. Brun found a sieve method (see Brun sieve) that permitted one to obtain the expected upper bound for 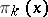 :
:
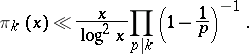 |
In addition to that, it was shown by the circle method (see [13]) with the help of Vinogradov's estimates for trigonometric sums with prime numbers (see Vinogradov method) that for any fixed 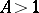 ,
, 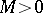 ,
,
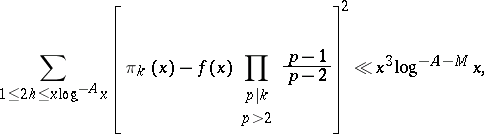 |
where
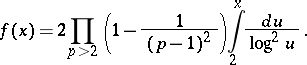 |
This implies, in particular, that for 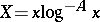 the following asymptotic expression for
the following asymptotic expression for 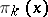 holds for all
holds for all  except for at most
except for at most 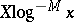 values of
values of 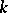 in the range
in the range  :
:
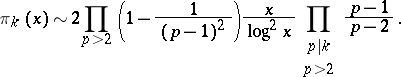 |
Similar results are obtained for sets of prime numbers 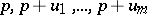 for all
for all 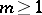 .
.
Prime numbers in arithmetic progressions.
The first method (of Euclid) for proving the infinitude of prime numbers can also be applied to certain arithmetic progressions. But the proof by this method that every arithmetic progression 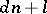 , where the first number
, where the first number 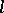 and the difference
and the difference  are relatively prime, contains infinitely many prime numbers has not yet (1983) been given. The problem was solved by another method by P. Dirichlet (1837–1840), who extended an idea of Euler in showing that
are relatively prime, contains infinitely many prime numbers has not yet (1983) been given. The problem was solved by another method by P. Dirichlet (1837–1840), who extended an idea of Euler in showing that 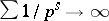 as
as 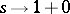 for prime numbers
for prime numbers 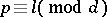 . To do this he introduced certain arithmetical functions — characters
. To do this he introduced certain arithmetical functions — characters 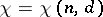 (see Dirichlet character) and functions
(see Dirichlet character) and functions 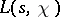 (see Dirichlet
(see Dirichlet 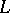 -function), which, like
-function), which, like 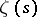 for
for 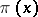 and
and  , serve as a basic means of studying
, serve as a basic means of studying 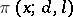 and its analogue
and its analogue
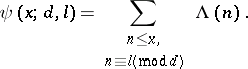 |
For a fixed value of 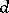 ,
, 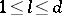 ,
, 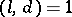 , the majority of results indicated above for
, the majority of results indicated above for  and
and 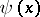 carry over to
carry over to 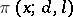 and
and 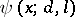 . However, of special interest here are the results for values of
. However, of special interest here are the results for values of 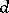 that increase with
that increase with  , as these are important in additive number theory and elsewhere. In this case, other significant difficulties arise, connected mostly with estimating
, as these are important in additive number theory and elsewhere. In this case, other significant difficulties arise, connected mostly with estimating 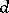 , the largest real zero of
, the largest real zero of 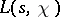 modulo
modulo 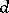 . It can be proved using the Page theorem that for
. It can be proved using the Page theorem that for 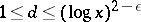 ,
, 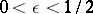 ,
,
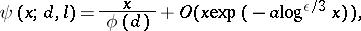 |
and as a result of C.L. Siegel's bound (1935), for any fixed 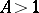 , for
, for 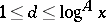 ,
,
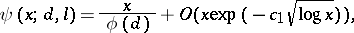 |
where  is the Euler
is the Euler 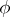 -function,
-function,  is a positive constant and
is a positive constant and 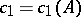 is an ineffective constant
is an ineffective constant 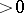 , that is,
, that is, 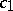 cannot be computed for given
cannot be computed for given 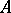 .
.
If the extended Riemann hypothesis (cf. Riemann hypotheses) is true, then for 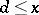 ,
,
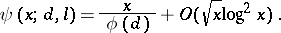 |
Thus, up till 1983 it has been proved that the prime numbers are uniformly distributed over all 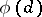 progressions
progressions 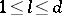 ,
, 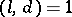 , of difference
, of difference  only for
only for 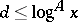 . As far as segments of progressions
. As far as segments of progressions 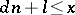 are concerned, for example with
are concerned, for example with 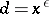 for any
for any 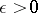 , it does not even follow from the above that such a progression contains at least one prime number.
, it does not even follow from the above that such a progression contains at least one prime number.
The sieve method of Brun, like the modification suggested by Selberg in 1947, shows that for all 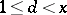 ,
,  ,
, 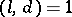 , there is an upper bound
, there is an upper bound
 |
with absolute constant  , but no lower bounds for
, but no lower bounds for 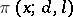 are obtainable by these methods.
are obtainable by these methods.
By the Riemann principle for a zero 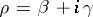 of
of 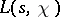 lying in the strip
lying in the strip 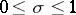 ,
,  has the form
has the form
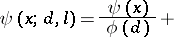 | (8) |
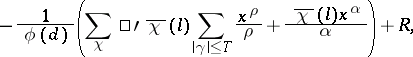 |
where the prime on the 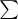 indicates summation over complex
indicates summation over complex 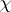
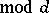 ,
, 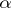 is the real zero of
is the real zero of 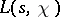 if it exists and is greater than
if it exists and is greater than  , and
, and
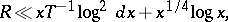 |
for any 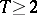 .
.
From (8) one sees that if one disregards the term corresponding to 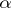 , the remainder term is determined by the double sum and depends also on the real part of
, the remainder term is determined by the double sum and depends also on the real part of 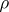 and on all the
and on all the  with
with 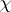
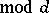 having a zero
having a zero 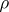 with
with 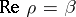 . If, for
. If, for 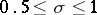 ,
, 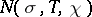 denotes the number of zeros of
denotes the number of zeros of 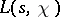 in the rectangle
in the rectangle 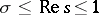 ,
,  , then the problem of estimating the remainder term for
, then the problem of estimating the remainder term for 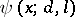 and its mean value is reduced to the problem of estimating the density of the distribution of the zeros of
and its mean value is reduced to the problem of estimating the density of the distribution of the zeros of 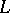 -functions:
-functions:
 | (9) |
Thus, the reduction of various estimates for prime numbers is concerned not so much with the lack of zeros of 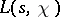 in the critical strip, but with their comparatively rare distribution there.
in the critical strip, but with their comparatively rare distribution there.
The realization of this idea has been one of the central directions of research on the distribution of prime numbers over the last forty years. A start was made by Yu.V. Linnik with his discovery in 1941 of the method of the large sieve (see [22], and also [14], [24]). Especially important are the theorems on the least prime number in an arithmetic progression, the behaviour of 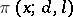 and
and 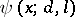 on the average for
on the average for  , and on the double averaging of these functions over
, and on the double averaging of these functions over 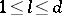 ,
, 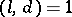 , and over
, and over 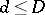 .
.
Namely, in 1944 Linnik [23] showed that as 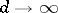 the sum
the sum 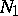 in (9) has the bound
in (9) has the bound 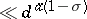 , where
, where  is a constant; from this he deduced the existence of a constant
is a constant; from this he deduced the existence of a constant  such that any arithmetic progression
such that any arithmetic progression 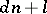 ,
,  ,
, 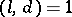 , contains a prime number less than
, contains a prime number less than 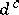 . The latest estimate of Linnik's constant is
. The latest estimate of Linnik's constant is 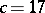 ; but if the density hypothesis is correct, i.e. if
; but if the density hypothesis is correct, i.e. if 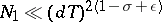 , then
, then  .
.
In 1965, A.I. Vinogradov and Bombieri independently obtained strong estimates for 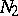 in (9). A more complete method of estimating these sums was found by H. Montgomery (1969). One of the results on estimating
in (9). A more complete method of estimating these sums was found by H. Montgomery (1969). One of the results on estimating  is the following result on the average distribution of prime numbers in arithmetic progressions:
is the following result on the average distribution of prime numbers in arithmetic progressions:
 |
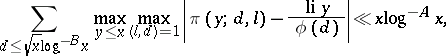 |
for any constant 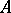 and
and 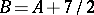 . These estimates cannot be substantially improved, because the extended Riemann hypothesis implies that
. These estimates cannot be substantially improved, because the extended Riemann hypothesis implies that 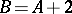 .
.
Other problems on the distribution of prime numbers are as follows. Let 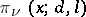 be the number of integers of the form
be the number of integers of the form 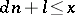 which are products of
which are products of  prime numbers. H. Richert (1953) proved that
prime numbers. H. Richert (1953) proved that
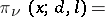 |
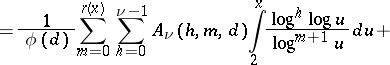 |
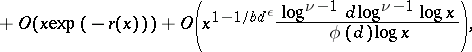 |
where  ,
, 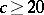 ,
, 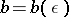 ,
, 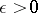 , and the
, and the 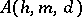 are determined by series depending on
are determined by series depending on 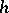 ,
, 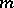 ,
, 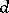 , and
, and  .
.
E. Landau (1903–1918) carried over certain results on the distribution of prime numbers to algebraic number fields. Let 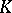 be an algebraic number field of degree
be an algebraic number field of degree  and let
and let 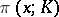 be the number of prime ideals in
be the number of prime ideals in 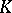 with norm
with norm 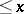 . Then
. Then
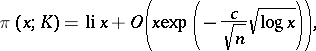 |
where  is an absolute positive constant, and
is an absolute positive constant, and
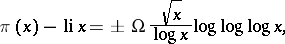 |
where  is the negation of the symbol
is the negation of the symbol  (small).
(small).
Much studied is the function 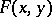 denoting the amount of natural numbers
denoting the amount of natural numbers 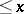 not containing prime divisors less than
not containing prime divisors less than  . For
. For 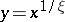 and
and 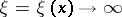 as
as  , such numbers are called quasi-prime numbers. By applying the sieve method to these numbers there arose a fairly complete theory of their distribution, similar to that expected from the distribution of prime numbers. The distribution of numbers with small prime divisors has also been considered (see [15]).
, such numbers are called quasi-prime numbers. By applying the sieve method to these numbers there arose a fairly complete theory of their distribution, similar to that expected from the distribution of prime numbers. The distribution of numbers with small prime divisors has also been considered (see [15]).
References
| [1] | L. Euler, "Einleitung in die Analysis des Unendlichen" , Springer (1983) (Translated from Latin) |
| [2] | P.L. Chebyshev, "Oeuvres de P.L. Tchebycheff" , 1–2 , Chelsea (1961) (Translated from Russian) |
| [3] | B. Riemann, "Werke" , Teubner (1892) |
| [4] | A.E. Ingham, "The distribution of prime numbers" , Cambridge Univ. Press (1932) |
| [5] | A.I. Vinogradov, "Selected works" , Springer (1985) (Translated from Russian) |
| [6] | I.M. Vinogradov, "A new estimate of the function  " Izv. Akad. Nauk SSSR Ser. Mat. , 22 : 2 (1958) pp. 161–164 (In Russian) " Izv. Akad. Nauk SSSR Ser. Mat. , 22 : 2 (1958) pp. 161–164 (In Russian) |
| [7] | I.M. Vinogradov, "The method of trigonometric sums in the theory of numbers" , Interscience (1954) (Translated from Russian) |
| [8] | E.C. Titchmarsh, "The theory of the Riemann zeta-function" , Clarendon Press (1951) |
| [9] | K. Prachar, "Primzahlverteilung" , Springer (1957) |
| [10] | A.A. Karatsuba, "Fundamentals of analytic number theory" , Moscow (1975) (In Russian) |
| [11] | L.-K. Hua, "Abschätzungen von Exponentialsummen und ihre Anwendung in der Zahlentheorie" , Enzyklopaedie der Mathematischen Wissenschaften mit Einschluss ihrer Anwendungen , 1 : 2 (1959) (Heft 13, Teil 1) |
| [12] | N.G. Chudakov, "Introductions to the theory of Dirichlet  -functions" , Moscow-Leningrad (1947) (In Russian) -functions" , Moscow-Leningrad (1947) (In Russian) |
| [13] | A.F. Lavrik, "On the distribution of primes based on I.M. Vinogradov's method of trigonometric sums" Trudy Mat. Inst. Steklov. , 64 (1961) pp. 90–125 (In Russian) |
| [14] | H. Davenport, "Multiplicative number theory" , Springer (1980) |
| [15] | H. Halberstam, H.-E. Richert, "Sieve methods" , Acad. Press (1974) |
| [16] | A. Selberg, "An elementary proof of the prime-number theorem" Ann. of Math. , 50 (1949) pp. 305–313 |
| [17] | P. Erdös, "On a new method in elementary number theory which leads to an elementary proof of the prime number theorem" Proc. Nat. Acad. Sci. USA , 35 (1949) pp. 374–384 |
| [18] | A.F. Lavrik, "Survey address" , Internat. Conf. on Number Theory , Moscow (1981) (In Russian) |
| [19] | H.G. Diamond, J. Steinig, "An elementary proof of the prime number theorem with a remainder term" Indag. Math. , 11 (1970) pp. 199–258 |
| [20] | A.F. Lavrik, A.Sh. Sobirov, "On the remainder term in the elementary proof of the prime number theorem" Soviet Math. Dokl. , 211 (1973) pp. 1063–1066 Dokl. Akad. Nauk SSSR , 211 : 3 (1973) pp. 534–536 |
| [21] | B. Rosser, "The  -th prime is greater than -th prime is greater than  " Proc. London Math. Soc. (2) , 45 (1939) pp. 21–44 " Proc. London Math. Soc. (2) , 45 (1939) pp. 21–44 |
| [22] | Yu.V. Linnik, "The large sieve" Dokl. Akad. Nauk SSSR , 30 : 4 (1941) pp. 292–294 (In Russian) |
| [23] | Yu.V. Linnik, "On the least prime in an arithmetic progression I. The basic theorem" Mat. Sb. , 15 (1944) pp. 139–178 (In Russian) |
| [24] | A.F. Lavrik, "A survey of Linnik's large sieve and the density theory of zeros of 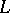 -functions" Russian Math. Surveys , 15 : 2 (1980) pp. 63–76 Uspekhi Mat. Nauk , 35 : 2 (1980) pp. 55–65 -functions" Russian Math. Surveys , 15 : 2 (1980) pp. 63–76 Uspekhi Mat. Nauk , 35 : 2 (1980) pp. 55–65 |
Comments
In formula (7) above, 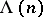 is the (von) Mangoldt function, which has the value
is the (von) Mangoldt function, which has the value  if
if  is a prime power
is a prime power 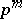 and is zero otherwise.
and is zero otherwise.
Concerning estimates of trigonometric sums see also Trigonometric sums, method of.
Some more facts. Although numerical tables do not suggest it, the function 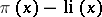 changes sign infinitely often. This was shown by Littlewood. By the work of H. Iwaniec, J. Pintz and C.J. Mozzochi it is now (1986) known that
changes sign infinitely often. This was shown by Littlewood. By the work of H. Iwaniec, J. Pintz and C.J. Mozzochi it is now (1986) known that 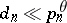 with
with 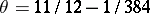 . The largest known prime twin is
. The largest known prime twin is 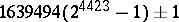 (W. Keller, 1983).
(W. Keller, 1983).
Many interesting facts, both computationally and theoretically, on the distribution of primes can be found in [a5], which is written in an elementary style. For elementary methods one can also consult [a1]. In [a4] one finds a simple proof of the prime number theorem.
The fact that there are infinitely many primes (i.e. 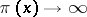 as
as 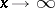 ) is also known as the Euclidean prime number theorem. The prime number theorem states that
) is also known as the Euclidean prime number theorem. The prime number theorem states that 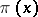 is asymptotically equal to
is asymptotically equal to 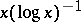 (cf. also de la Vallée-Poussin theorem). The Dirichlet prime number theorem states that there are infinitely many primes in every arithmetic progression (i.e.
(cf. also de la Vallée-Poussin theorem). The Dirichlet prime number theorem states that there are infinitely many primes in every arithmetic progression (i.e. 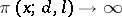 as
as  ).
).
For the result of Bombieri–Vinogradov see also (the references to) Density hypothesis.
References
| [a1] | H.G. Diamond, "Elementary methods in the study of the distribution of prime numbers" Bull. Amer. Math. Soc. , 7 (1982) pp. 553–589 |
| [a2] | W.J. Ellison, M. Mendès France, "Les nombres premiers" , Hermann (1975) |
| [a3] | A. Ivic, "The Riemann zeta-function" , Wiley (1985) |
| [a4] | D.J. Newman, "Simple analytic proof of the prime number theorem" Amer. Math. Monthly , 87 (1980) pp. 693–696 |
| [a5] | H. Riesel, "Prime numbers and computer methods for factorisation" , Birkhäuser (1985) |
| [a6] | E. Bombieri, "La grand crible dans la théorie analytique des nombres" Astérisque , 18 (1974) |
Distribution of prime numbers. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Distribution_of_prime_numbers&oldid=12093