Distribution of power residues and non-residues
The distribution among the numbers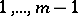 
of those values of 
for which the congruence
 |
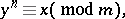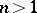, is solvable (or unsolvable) in integers. Questions on the distribution of power residues and non-residues have been studied most fully in the case modulo a prime number 
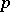. Let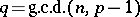 
. Then the congruence 
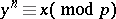is solvable for 
values of 
in the set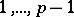 
and unsolvable for the remaining 
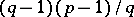values (see Two-term congruence). However, comparatively little is known about how these values are distributed among the numbers 
.
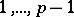The first results about the distribution of power residues were obtained by C.F. Gauss (see [1]) in 1796. From that time until the work of I.M. Vinogradov only isolated special results were obtained on questions concerning the distribution of power residues and non-residues. In 1915, Vinogradov (see [2]) proved a series of general results about the distribution of power residues and non-residues, and about primitive roots (cf. Primitive root) modulo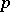 
among the numbers 
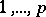. In particular, he obtained the bound
 |
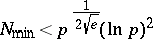for the least quadratic non-residue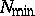 
, and the bound
 |
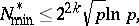where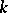 
is the number of distinct prime divisors of 
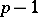, for the least primitive root 
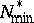modulo 
.
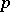In addition, he made a number of conjectures on the distribution of quadratic residues and non-residues (see Vinogradov hypotheses) which stimulated a series of investigations in this area. Thus, Yu.V. Linnik [3] proved that for sufficiently large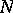 
, the number of prime numbers 
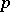in the interval 
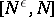for which 
does not exceed a certain constant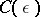 
, depending only on 
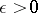. Thus, the prime numbers 
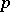for which 
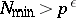, if they exist at all, are met only very rarely. Another significant step in the work on Vinogradov's conjectures was the theorem of D.A. Burgess [4]: For any fixed sufficiently small 
, the maximal distance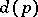 
between neighbouring quadratic non-residues satisfies the inequality
 |
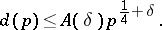In particular, one has
 |
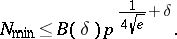In these inequalities, the constants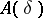 
, 
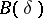depend only on 
and not on 
. The proof of Burgess' theorem is very complicated; it is based on the Hasse–Weil theorem on the number of solutions of the hyper-elliptic congruence
 |
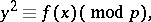the proof of which requires techniques of abstract algebraic geometry. For a simple account of Burgess' theorem see [5], [6].
References
| [1] | C.F. Gauss, "Untersuchungen über höhere Arithmetik" , A. Maser (1889) (Translated from Latin) MR0188045 Zbl 21.0166.04 |
| [2] | I.M. Vinogradov, "Selected works" , Springer (1985) (Translated from Russian) MR0807530 Zbl 0577.01049 |
| [3] | Yu.V. Linnik, Dokl. Akad. Nauk SSSR , 36 (1942) pp. 131 |
| [4] | D.A. Burgess, "The distribution of quadratic residues and non-residues" Mathematika , 4 : 8 (1957) pp. 106–112 MR0093504 Zbl 0081.27101 |
| [5] | S.A. Stepanov, "Constructive methods in the theory of equations over finite fields" Proc. Steklov Inst. Math. , 132 (1975) pp. 271–281 Trudy Mat. Inst. Steklov. , 132 (1973) pp. 237–246 MR0337976 |
| [6] | A.A. Karatsuba, "Character sums and primitive roots in finite fields" Soviet Math.-Dokl. , 9 : 3 (1968) pp. 755–757 Dokl. Akad. Nauk SSSR , 180 : 6 (1968) pp. 1287–1289 Zbl 0182.37501 |
Distribution of power residues and non-residues. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Distribution_of_power_residues_and_non-residues&oldid=14183