Dirac equation
A relativistic wave equation which plays a fundamental role in relativistic quantum mechanics and in quantum field theory. It is used for describing particles with spin 1/2 (in 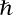 units), i.e. electrons, neutrinos, muons, protons, neutrons, etc., and also positrons and all other anti-particles and hypothetical subparticles — quarks. The Dirac equation is the foundation of the theory of particles with half-integral spin (1/2, 3/2, 5/2, etc.), i.e. fermions which obey the Fermi statistics. Thus, the Rarita–Schwinger equation is a generalization of the Dirac equation for particles with spin 3/2.
units), i.e. electrons, neutrinos, muons, protons, neutrons, etc., and also positrons and all other anti-particles and hypothetical subparticles — quarks. The Dirac equation is the foundation of the theory of particles with half-integral spin (1/2, 3/2, 5/2, etc.), i.e. fermions which obey the Fermi statistics. Thus, the Rarita–Schwinger equation is a generalization of the Dirac equation for particles with spin 3/2.
The Dirac equation is a system of four linear homogeneous partial differential equations of the first order with constant complex coefficients, which is invariant with respect to the general Lorentz group of transformations
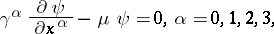 |
where  ,
, 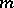 is the rest mass,
is the rest mass, 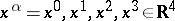 with the pseudo-Euclidean metric
with the pseudo-Euclidean metric 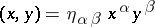 and
and
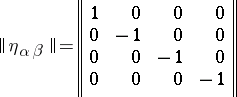 |
is the metric tensor of the Minkowski space with signature  ;
;  is the Dirac spinor (bi-spinor):
is the Dirac spinor (bi-spinor):
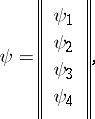 |
and 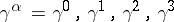 are the Dirac matrices, which satisfy
are the Dirac matrices, which satisfy 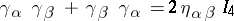 .
.
Under the transformations of the variables from the general Lorentz group 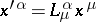 [2] the bi-spinor
[2] the bi-spinor 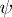 is transformed in accordance with the formula
is transformed in accordance with the formula 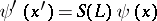 where
where 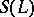 is a non-singular complex matrix of dimension
is a non-singular complex matrix of dimension 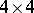 . The matrices
. The matrices  form a special two-valued representation of the group
form a special two-valued representation of the group 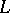 (
(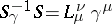 ). The Dirac equation does not change its form with respect to the new variables
). The Dirac equation does not change its form with respect to the new variables 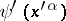 (relativistic invariance):
(relativistic invariance):
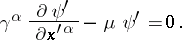 |
The case 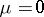 yields the Weyl equation, which describes the neutrino. Here, the Dirac equation is subdivided into two independent equations for spinor functions (van der Waerden spinors)
yields the Weyl equation, which describes the neutrino. Here, the Dirac equation is subdivided into two independent equations for spinor functions (van der Waerden spinors) 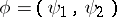 and
and 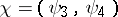 . None of them will be invariant with respect to reflections (a theory in which parity is not preserved).
. None of them will be invariant with respect to reflections (a theory in which parity is not preserved).
Any solution of the Dirac equation satisfies the Klein–Gordon equation, which describes spin-less scalar particles
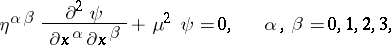 |
but not every solution of this equation satisfies the Dirac equation, which is obtained by factorization of the Klein–Gordon equation.
It follows from the Dirac equation that electrons have intrinsic angular momentum (spin) 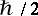 . The Dirac equation is a complete description of the motion of atomic electrons in the field of the nucleus and in other electromagnetic fields, and also of the interaction of an electron with certain elementary particles.
. The Dirac equation is a complete description of the motion of atomic electrons in the field of the nucleus and in other electromagnetic fields, and also of the interaction of an electron with certain elementary particles.
Any relativistically invariant equation can be represented in the form of the Dirac equation:
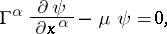 |
where 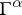 is a generalization of
is a generalization of 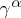 . In the Klein–Gordon equation the function
. In the Klein–Gordon equation the function 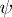 has five components, while
has five components, while  are four five-row matrices which satisfy the relations:
are four five-row matrices which satisfy the relations:
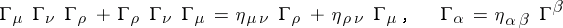 |
(Duffin–Kemmer matrices).
The interaction of fermions with an electromagnetic field is allowed for by exchanging the derivative 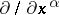 for the compensating derivative
for the compensating derivative 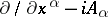 (where
(where 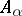 is the
is the 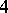 -potential of the electro-magnetic field). In the interaction of fermions with the general gauge field (Yang–Mills field) the compensating derivative is
-potential of the electro-magnetic field). In the interaction of fermions with the general gauge field (Yang–Mills field) the compensating derivative is 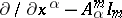 (where
(where 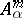 are the
are the 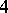 -potentials of the field and the
-potentials of the field and the 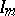 form a basis of the Lie algebra, i.e. are generators of the Lie group). In a similar manner, allowance for the interactions of fermions with the gravitational field, in accordance with the general theory of relativity, results in the generalization of the Dirac equation to a Riemannian space, by introducing a corresponding compensating (covariant) derivative [3]:
form a basis of the Lie algebra, i.e. are generators of the Lie group). In a similar manner, allowance for the interactions of fermions with the gravitational field, in accordance with the general theory of relativity, results in the generalization of the Dirac equation to a Riemannian space, by introducing a corresponding compensating (covariant) derivative [3]:
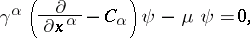 |
where 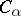 are the spinor connection coefficients, initially defined with the aid of the tetrad formalism, which satisfy the relations
are the spinor connection coefficients, initially defined with the aid of the tetrad formalism, which satisfy the relations
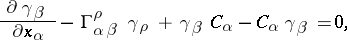 |
or
 |
where  are the Christoffel symbols. The general relativistic generalization of the Dirac equation is indispensable in the study of gravitational collapse, in the description of the predicted effect of particle generation in strong gravitational fields, etc.
are the Christoffel symbols. The general relativistic generalization of the Dirac equation is indispensable in the study of gravitational collapse, in the description of the predicted effect of particle generation in strong gravitational fields, etc.
In a space with torsion, the Dirac equation includes a non-linear increment of cubic type [4] and it becomes the non-linear equation
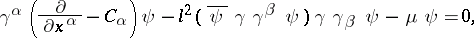 |
where 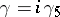 ,
, 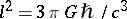 , and
, and 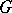 is the gravitation constant.
is the gravitation constant.
By analogy, in a non-metric space-time (Weyl space-time) the Dirac equation also includes a non-linear increment of cubic type [5]:
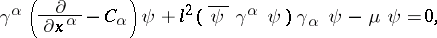 |
where 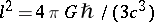 .
.
The equation was introduced in 1928 by P. Dirac.
References
| [1] | P.A.M. Dirac, "The principles of quantum mechanics" , Clarendon Press (1947) |
| [2] | N.N. Bogolyubov, D.V. Shirkov, "Introduction to the theory of quantized fields" , Interscience (1959) (Translated from Russian) |
| [3] | D.R. Brill, J.A. Wheeler, "Neutrinos in the gravitational field" Rev. Modern Physics , 29 (1957) pp. 465 |
| [4] | V.I. Rodichev, "The spinor field in space-time with torsion" Zh. Eksper. Teor. Fiz. , 40 (1969) pp. 1469 (In Russian) |
| [5] | V.G. Krechet, "The spinor field and nonmetricity of space-time" Izv. Voozov. Fiz. , 6 (1980) pp. 52 (In Russian) |
| [6] | J.D. Bjorken, "Relativistic quantum theory" , 1 , McGraw-Hill (1964) |
Comments
References
| [a1] | H. Umezawa, "Quantum field theory" , North-Holland (1956) |
| [a2] | Y. Takahashi, "An introduction to field quantization" , Pergamon (1969) |
| [a3] | R. Roman, "Theory of elementary particles" , North-Holland (1960) |
| [a4] | V.S. Varadarajan, "Geometry of quantum theory" , 1–2 , v. Nostrand (1968) (Translated from Russian) |
| [a5] | S.W. Hawking, G.F.R. Ellis, "The large scale structure of spacetime" , Cambridge Univ. Press (1973) |
Dirac equation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Dirac_equation&oldid=19106