Differential-geometric structure
One of the fundamental concepts in modern differential geometry including the specific structures studied in classical differential geometry. It is defined for a given differentiable manifold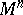 
as a differentiable section in a fibre space 
with base 
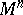associated with a certain principal bundle 
or, according to another terminology, as a differentiable field of geometric objects on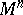 
. Here 
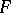is some differentiable 
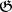-space where 
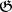is the structure Lie group of the principal bundle 
or, in another terminology, the representation space of the Lie group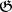 
.
If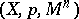 
is the principal bundle of frames in the tangent space to 
, 
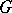is some closed subgroup in 
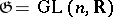, and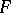 
is the homogeneous space 
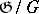, the corresponding differential-geometric structure on 
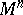is called a 
-structure or an infinitesimal structure of the first order. For example, if 
consists of those linear transformations (elements of 
) which leave an 
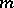-dimensional space in 
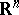invariant, the corresponding 
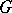-structure defines a distribution of 
-dimensional subspaces on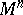 
. If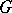 
is the orthogonal group 
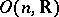— the subgroup of elements of 
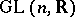which preserve the scalar product in 
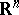—, then the 
-structure is a Riemannian metric on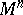 
, i.e. the field of a positive-definite symmetric tensor 
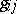. In a similar manner, almost-complex and complex structures are special cases of 
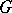-structures on 
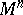. A generalization of the concept of a 
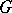-structure is an infinitesimal structure of order 
, 
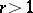(or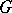 
-structure of a higher order); here 
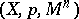is the principal bundle of frames of the order 
on 
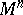, and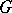 
is a closed subgroup of its structure group 
.
All kinds of connections (cf. Connection) are important special cases of differential-geometric structures. For instance, a connection in a principal bundle is obtained if the role of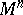 
is played by the space 
of some principal bundle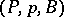 
, and the 
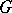-structure on 
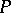is the distribution of 
-dimensional,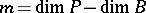 
, subspaces complementary to the tangent spaces of the fibres which is invariant with respect to the action on 
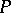of the structure group of the bundle. Connections on a manifold 
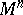are special cases of differential-geometric structures on 
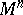, but more general ones than 
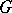-structures on 
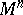. For instance, an affine connection on 
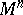, definable by a field of connection objects 
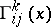, is obtained as the differential-geometric structure on 
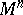for which 
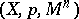is the principal bundle of frames of second order, 
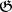is its structure group 
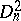, and the representation space 
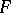of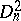 
is the space 
with coordinates 
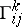, where the representation is defined by the formulas
 |
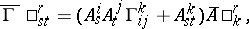where
 |
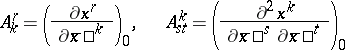are the coordinates of an element of the group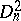 
, and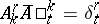 
. In the case of a projective connection on 
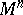one deals with a certain representation of 
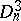in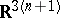 
, while in cases of connections of a higher order, one deals with representations of 
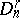. By this approach the theory of differential-geometric structures becomes closely related to the theory of geometric objects (Cf. Geometric objects, theory of).
References
| [1] | O. Veblen, J.H.C. Whitehead, "The foundations of differential geometry" , Cambridge Univ. Press (1932) (Appendix by V.V. Vagner in the Russian translation) |
| [2] | G.F. Laptev, "Differential geometry of imbedded manifolds. Group-theoretical method of differential geometric investigation" Trudy Moskov. Mat. Obshch. , 2 (1953) pp. 275–382 (In Russian) |
| [3] | D. Husemoller, "Fibre bundles" , McGraw-Hill (1966) |
| [4] | S. Sternberg, "Lectures on differential geometry" , Prentice-Hall (1964) |
Comments
References
| [a1] | M. Spivak, "A comprehensive introduction to differential geometry" , 1979 , Publish or Perish (1972–1975) pp. 1–5 |
Differential-geometric structure. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Differential-geometric_structure&oldid=46661