Derived automorphism
in ergodic theory
A transformation  defined by using an automorphism
defined by using an automorphism 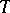 of a measure space
of a measure space  and a measurable subset
and a measurable subset 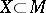 of positive measure such that almost-all points of
of positive measure such that almost-all points of  return to
return to 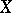 under the action of iterates of
under the action of iterates of 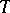 . For every such point
. For every such point  its image
its image 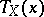 is defined as that point of the trajectory
is defined as that point of the trajectory 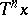 at which this trajectory returns to
at which this trajectory returns to  for the first time after
for the first time after  (according to the Poincaré recurrence theorem, cf. Poincaré return theorem, the condition for almost-all points of
(according to the Poincaré recurrence theorem, cf. Poincaré return theorem, the condition for almost-all points of  to return to
to return to  at some time is automatically satisfied if
at some time is automatically satisfied if 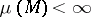 ). The transformation
). The transformation  turns out to be an automorphism (more precisely, an automorphism modulo
turns out to be an automorphism (more precisely, an automorphism modulo  ) of the space
) of the space 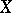 with the measure induced on it (this measure is the measure
with the measure induced on it (this measure is the measure  considered only on subsets of
considered only on subsets of  ; if
; if 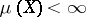 then this measure is usually normalized).
then this measure is usually normalized).
Conversely, if 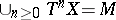 (this condition is automatically satisfied if the automorphism
(this condition is automatically satisfied if the automorphism 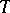 is ergodic), then the original automorphism
is ergodic), then the original automorphism 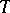 can be recovered (up to conjugation by means of an isomorphism of measure spaces) from
can be recovered (up to conjugation by means of an isomorphism of measure spaces) from  and the time of first return
and the time of first return
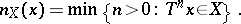 |
Namely, 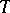 is the special automorphism constructed from
is the special automorphism constructed from  and
and  .
.
Comments
For automorphism of a measure space cf. Measure-preserving transformation.
In the literature also induced or derivative automorphism are used. See [a1] or [a2].
References
| [a1] | S. Kakutani, "Induced measure preserving transformations" Proc. Japan. Acad. , 19 (1943) pp. 635–641 |
| [a2] | K. Petersen, "Ergodic theory" , Cambridge Univ. Press (1983) pp. 39 |
Derived automorphism. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Derived_automorphism&oldid=13035