Darboux sum
A sum of special type. Let a real function 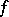 be defined and bounded on a segment
be defined and bounded on a segment 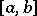 , let
, let 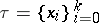 be a decomposition of
be a decomposition of 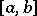 :
:
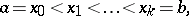 |
and set
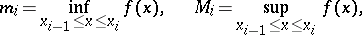 |
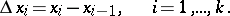 |
The sums
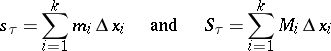 |
are known, respectively, as the lower and upper Darboux sums. For any two decompositions  and
and  of
of 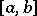 the inequality
the inequality  is valid, i.e. any lower Darboux sum is no larger than an upper. If
is valid, i.e. any lower Darboux sum is no larger than an upper. If
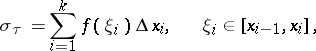 |
is a Riemann sum, then
 |
The geometric meaning of the lower and upper Darboux sums is that they are equal to the planar areas of stepped figures consisting of rectangles whose base widths are 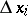 and with respective heights
and with respective heights 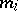 and
and  (see Fig.) if
(see Fig.) if 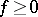 . These figures approximate, from the inside and outside, the curvilinear trapezium formed by the graph of
. These figures approximate, from the inside and outside, the curvilinear trapezium formed by the graph of 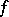 , the abscissa axis and the rectilinear segments
, the abscissa axis and the rectilinear segments  and
and 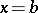 (which may degenerate into points).
(which may degenerate into points).
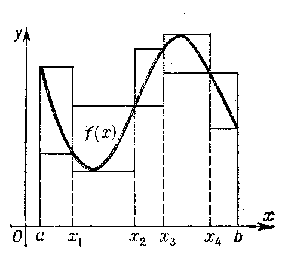
Figure: d030160a
The numbers
 | (1) |
are called, respectively, the lower and the upper Darboux integrals of 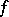 . They are the limits of the lower and the upper Darboux sums:
. They are the limits of the lower and the upper Darboux sums:
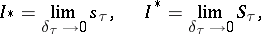 |
where
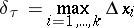 |
is the fineness (mesh) of the decomposition  . The condition
. The condition
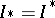 | (2) |
is necessary and sufficient for a function 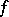 to be Riemann integrable on the segment
to be Riemann integrable on the segment 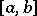 . Here, if condition (2) is met, the value of the lower and the upper Darboux integrals becomes identical with the Riemann integral
. Here, if condition (2) is met, the value of the lower and the upper Darboux integrals becomes identical with the Riemann integral
 |
With the aid of Darboux sums, condition (2) may be formulated in the following equivalent form: For each 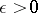 there exists a decomposition
there exists a decomposition  such that
such that
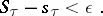 |
The condition
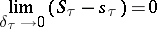 |
is also necessary and sufficient for the Riemann integrability of 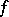 on
on 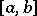 . Here
. Here
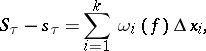 |
where  is the oscillation (cf. Oscillation of a function) of
is the oscillation (cf. Oscillation of a function) of  on
on
 |
The concept of lower and upper Darboux sums may be generalized to the case of functions of several variables which are measurable in the sense of some positive measure 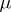 . Let
. Let  be a measurable (for example, Jordan or Lebesgue) subset of the
be a measurable (for example, Jordan or Lebesgue) subset of the  -dimensional space,
-dimensional space, 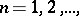 and suppose
and suppose 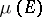 is finite. Let
is finite. Let  be a decomposition of
be a decomposition of 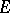 , i.e. a system of measurable subsets of
, i.e. a system of measurable subsets of 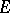 such that
such that
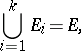 | (3) |
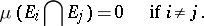 | (4) |
Let a function 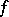 be bounded on
be bounded on 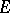 and let
and let
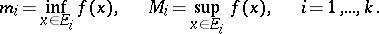 | (5) |
The sums
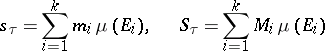 | (6) |
are also said to be, respectively, lower and upper Darboux sums. The lower 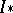 and the upper
and the upper 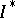 integrals are defined by formulas (1). For Jordan measure, their equality is a sufficient and necessary condition for the function to be Riemann integrable, and their common value coincides with the Riemann integral. For Lebesgue measure, on the other hand, the equality
integrals are defined by formulas (1). For Jordan measure, their equality is a sufficient and necessary condition for the function to be Riemann integrable, and their common value coincides with the Riemann integral. For Lebesgue measure, on the other hand, the equality
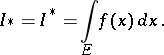 |
is always valid for bounded Lebesgue-measurable functions.
In general, if 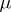 is a complete
is a complete 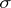 -additive bounded measure, defined on a
-additive bounded measure, defined on a 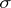 -algebra
-algebra  , if
, if  is a bounded measurable real-valued function on
is a bounded measurable real-valued function on 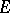 , if
, if 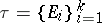 is a decomposition of a set
is a decomposition of a set 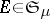 into
into 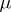 -measurable sets
-measurable sets 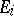 which satisfy the conditions (3) and (4), and if the Darboux sums
which satisfy the conditions (3) and (4), and if the Darboux sums 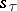 and
and 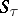 are defined by formulas (5) and (6), while the integrals
are defined by formulas (5) and (6), while the integrals 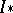 and
and 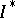 are defined by the formulas (1), in which
are defined by the formulas (1), in which 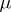 is always understood to mean the measure under consideration, then
is always understood to mean the measure under consideration, then
 |
A generalization of the Darboux sums to unbounded 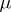 -measurable functions
-measurable functions  defined on sets
defined on sets 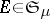 are the series (if they are absolutely convergent)
are the series (if they are absolutely convergent)
 | (7) |
where 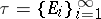 is a decomposition of
is a decomposition of  (this decomposition consists, generally speaking, of an infinite number of
(this decomposition consists, generally speaking, of an infinite number of 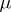 -measurable sets
-measurable sets 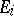 which satisfy condition (4) and are, of course, such that
which satisfy condition (4) and are, of course, such that 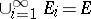 ), while
), while 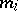 and
and  are defined by (5). In (7) (as in (6) above) it is assumed that
are defined by (5). In (7) (as in (6) above) it is assumed that 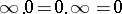 . If
. If 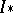 and
and 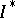 are again defined according to (1) and
are again defined according to (1) and 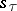 and
and 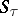 are now defined in the sense of (7) and exist for each
are now defined in the sense of (7) and exist for each  , then
, then  . If the value
. If the value 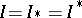 is finite, then
is finite, then 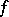 is integrable with respect to
is integrable with respect to  and
and  .
.
Named after G. Darboux [1].
References
| [1] | G. Darboux, Ann. Sci. Ecole Norm. Sup. Ser. 2 , 4 (1875) pp. 57–112 |
| [2] | V.A. Il'in, E.G. Poznyak, "Fundamentals of mathematical analysis" , 1–2 , MIR (1982) (Translated from Russian) |
| [3] | L.D. Kudryavtsev, "Mathematical analysis" , 1–2 , Moscow (1973) (In Russian) |
| [4] | S.M. Nikol'skii, "A course of mathematical analysis" , 1–2 , MIR (1977) (Translated from Russian) |
Darboux sum. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Darboux_sum&oldid=18687