Curvilinear integral

line integral

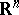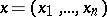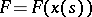An integral along a curve. In -dimensional Euclidean space consider a given rectifiable curve , , where is the arc length; let be a function defined on . The curvilinear integral

is defined by the equality

(1)

(the integral on the right is an integral over a real interval), and is called a line integral of the first kind, or a line integral with respect to arc length. It is the limit of suitable integral sums, which can be described in terms related to the curve. For example, if is Riemann-integrable (see Riemann integral), 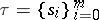is a partition of 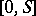, 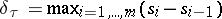is its mesh, , 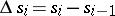is the length of the section of 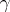from the point 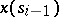to the point 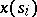, , 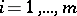and

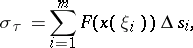then

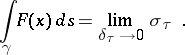If the rectifiable curve 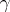is given parametrically by , , 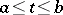and is a function defined on 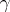, then the integral

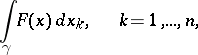is defined by

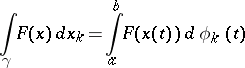(2)

(the integral on the right is a Stieltjes integral), and is called a line integral of the second kind or a line integral with respect to the coordinate 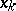. It is also the limit of suitably constructed Riemann sums: If 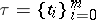is a partition of 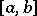, , , , 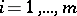and

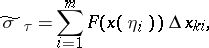then

If 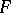is a continuous function on 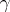, then the curvilinear integrals (1) and (2) always exist. If 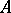is the initial point and 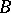the end point of 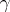, then the curvilinear integrals (1) and (2) are denoted by

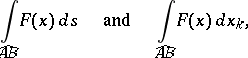respectively.

Line integrals of the first kind are independent of the orientation of the curve:

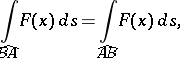but line integrals of the second kind change sign when the orientation is reversed:

If 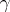is a continuously differentiable curve given by a continuously differentiable representation 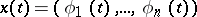, , 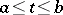and 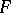is a continuous function on 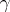, then

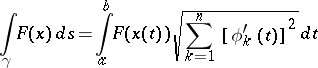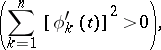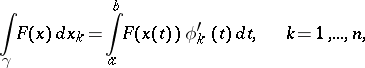and hence the integrals on the right of these equalities are independent of the choice of the parameter on . If 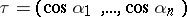is a unit tangent vector to the curve , then the line integral of the second kind may be expressed in terms of a line integral of the first kind via the formula

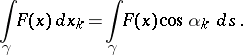If 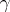is given in vector notation 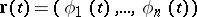and 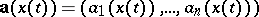is a vector function defined on , then, by definition,

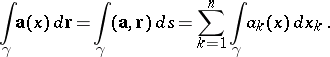The relationship between line integrals and integrals of other types is established by the Green formulas and the Stokes formula.

Line integrals may be used to calculate the area of plane domains: If a finite plane domain 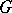is bounded by a simple rectifiable curve 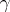, then its area is

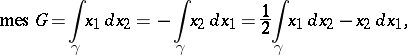where the contour is oriented in the counter-clockwise sense.

If 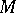is a mass distributed over 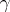with linear density 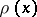, then

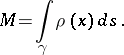If 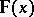is the intensity of a force field (i.e. the force acting on a unit mass), then

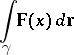is equal to the work performed by the field in moving a unit mass along .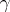

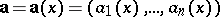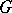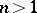Line integrals are used in the theory of vector fields. If is a continuous vector field defined on some -dimensional domain , , then the following three properties are equivalent:

1) For any closed rectifiable curve ,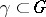

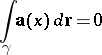(a vector field possessing this property is called a potential field).

2) For any pair of points 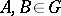and any two rectifiable curves 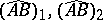with initial point and end point :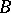

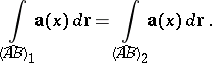3) There exists in 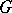a function 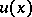(called a potential function of the field 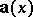), such that 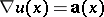, i.e. 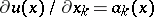, , 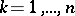and moreover, for any 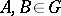and any curve ,

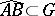If 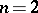or and 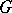is a simply-connected domain (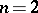) or a simply-connected surface (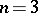), while the field 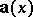is continuously differentiable, then the properties 1)–3) are equivalent to the following property:

4) The rotation of the vector field vanishes in :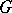

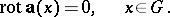If 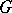is not simply connected, then 4) need not be equivalent to 1)–3). For example, for the field

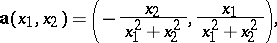defined on the plane punctured at the origin one has 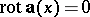, , 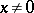but

References

[1]	V.A. Il'in, E.G. Poznyak, "Fundamentals of mathematical analysis" , 2 , MIR (1982) (Translated from Russian)
[2]	L.D. Kudryavtsev, "A course in mathematical analysis" , 2 , Moscow (1981) (In Russian)
[3]	S.M. Nikol'skii, "A course of mathematical analysis" , 2 , MIR (1977) (Translated from Russian)

Comments

Line integrals are a special instance of integrals of differential forms over chains, namely integrals of a 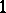-form over a 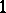-chain (cf. Differential form; Chain and especially Integration on manifolds).

References

[a1]	W. Rudin, "Principles of mathematical analysis" , McGraw-Hill (1976) pp. 107–108
[a2]	M. Spivak, "Calculus on manifolds" , Benjamin/Cummings (1965)