Convex subset
From Encyclopedia of Mathematics
of a partially ordered set
A subset containing with any two elements  and
and  the entire interval
the entire interval 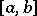 (cf. Interval and segment).
(cf. Interval and segment).
Comments
A definition not involving the notion of interval is: A subset 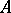 of a partially ordered set is convex if
of a partially ordered set is convex if 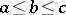 and
and 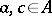 imply
imply 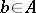 .
.
In the real line (with its usual ordering) the convex subsets are exactly the connected subsets (for the usual topology). This need not hold for more general ordered topological spaces. However, if a partially ordered set is equipped with the interval topology (cf. Order topology), then its connected subsets are convex.
How to Cite This Entry:
Convex subset. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Convex_subset&oldid=12474
Convex subset. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Convex_subset&oldid=12474
This article was adapted from an original article by L.A. Skornyakov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article