Contraction (operator theory)
contracting operator, contractive operator, compression
A bounded linear mapping 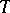 of a Hilbert space
of a Hilbert space 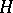 into a Hilbert space
into a Hilbert space 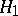 with
with 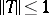 . For
. For 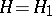 , a contractive operator
, a contractive operator 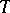 is called completely non-unitary if it is not a unitary operator on any
is called completely non-unitary if it is not a unitary operator on any  -reducing subspace different from
-reducing subspace different from  . Such are, for example, the one-sided shifts (in contrast to the two-sided shifts, which are unitary). Associated with each contractive operator
. Such are, for example, the one-sided shifts (in contrast to the two-sided shifts, which are unitary). Associated with each contractive operator  on
on 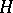 there is a unique orthogonal decomposition,
there is a unique orthogonal decomposition, 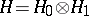 , into
, into  -reducing subspaces such that
-reducing subspaces such that 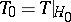 is unitary and
is unitary and  is completely non-unitary.
is completely non-unitary. 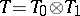 is called the canonical decomposition of
is called the canonical decomposition of 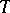 .
.
A dilation of a given contractive operator acting on 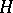 is a bounded operator
is a bounded operator 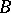 acting on some large Hilbert space
acting on some large Hilbert space  such that
such that 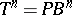 ,
, 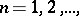 where
where  is the orthogonal projection of
is the orthogonal projection of  onto
onto 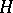 . Every contractive operator in a Hilbert space
. Every contractive operator in a Hilbert space 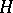 has a unitary dilation
has a unitary dilation 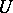 on a space
on a space 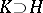 , which, moreover, is minimal in the sense that
, which, moreover, is minimal in the sense that  is the closed linear span of
is the closed linear span of 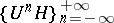 (the Szökefalvi-Nagy theorem). Minimal unitary dilations and functions of them, defined via spectral theory, allow one to construct a functional calculus for contractive operators. This has been done essentially for bounded analytic functions in the open unit disc
(the Szökefalvi-Nagy theorem). Minimal unitary dilations and functions of them, defined via spectral theory, allow one to construct a functional calculus for contractive operators. This has been done essentially for bounded analytic functions in the open unit disc  (the Hardy class
(the Hardy class 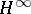 ). A completely non-unitary contractive operator
). A completely non-unitary contractive operator 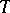 belongs, by definition, to the class
belongs, by definition, to the class 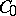 if there is a function
if there is a function 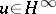 ,
, 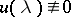 , such that
, such that 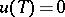 . The class
. The class 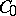 is contained in the class
is contained in the class 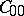 of contractive operators
of contractive operators 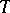 for which
for which  ,
, 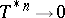 as
as 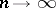 . For every contractive operator of class
. For every contractive operator of class 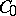 there is the so-called minimal function
there is the so-called minimal function 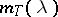 (that is, an inner function
(that is, an inner function  ,
, 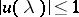 in
in 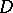 ,
,  almost-everywhere on the boundary of
almost-everywhere on the boundary of  ) such that
) such that 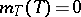 and
and 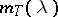 is a divisor of all other inner functions with the same property. The set of zeros of the minimal function
is a divisor of all other inner functions with the same property. The set of zeros of the minimal function 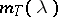 of a contractive operator
of a contractive operator 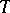 in
in  , together with the complement in the unit circle of the union of the arcs along which
, together with the complement in the unit circle of the union of the arcs along which 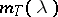 can be analytically continued, coincides with the spectrum
can be analytically continued, coincides with the spectrum 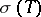 . The notion of a minimal function of a contractive operator
. The notion of a minimal function of a contractive operator 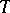 of class
of class 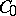 allows one to extend the functional calculus for this class of contractive operators to certain meromorphic functions in
allows one to extend the functional calculus for this class of contractive operators to certain meromorphic functions in 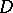 .
.
The theorem on unitary dilations has been obtained not only for individual contractive operators but also for discrete,  ,
,  and continuous,
and continuous, 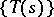 ,
, 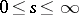 , semi-groups of contractive operators.
, semi-groups of contractive operators.
As for dissipative operators (cf. Dissipative operator), also for contractive operators a theory of characteristic operator-valued functions has been constructed and, on the basis of this, also a functional model, which allows one to study the structure of contractive operators and the relations between the spectrum, the minimal function and the characteristic function (see [1]). By the Cayley transformation
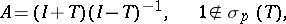 |
a contractive operator 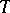 is related to a maximal accretive operator
is related to a maximal accretive operator  , that is,
, that is, 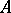 is such that
is such that 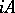 is a maximal dissipative operator. Constructed on this basis is the theory of dissipative extensions
is a maximal dissipative operator. Constructed on this basis is the theory of dissipative extensions 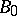 of symmetric operators
of symmetric operators 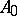 (respectively, Philips dissipative extensions
(respectively, Philips dissipative extensions  of conservative operators
of conservative operators 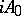 ).
).
The theories of similarity, quasi-similarity and unicellularity have been developed for contractive operators. The theory of contractive operators is closely connected with the prediction theory of stationary stochastic processes and scattering theory. In particular, the Lax–Philips scheme [2] can be considered as a continual analogue of the Szökefalvi-Nagy–Foias theory of contractive operators of class  .
.
References
| [1] | B. Szökefalvi-Nagy, Ch. Foiaş, "Harmonic analysis of operators in Hilbert space" , North-Holland (1970) (Translated from French) |
| [2] | P.D. Lax, R.S. Philips, "Scattering theory" , Acad. Press (1967) |
Comments
A reducing subspace for an operator 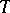 is a closed subspace
is a closed subspace 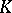 such that there is a complement
such that there is a complement 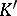 , i.e.
, i.e. 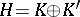 , such that both
, such that both 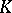 and
and 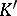 are invariant under
are invariant under 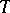 , i.e.
, i.e. 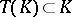 ,
, 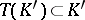 .
.
References
| [a1] | I.C. [I.Ts. Gokhberg] Gohberg, M.G. Krein, "Introduction to the theory of linear nonselfadjoint operators" , Transl. Math. Monogr. , 18 , Amer. Math. Soc. (1969) (Translated from Russian) |
| [a2] | I.C. [I.Ts. Gokhberg] Gohberg, M.G. Krein, "Theory and applications of Volterra operators in Hilbert space" , Amer. Math. Soc. (1970) (Translated from Russian) |
Contraction (operator theory). Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Contraction_(operator_theory)&oldid=13390