Connected sum

of a family of sets

The union of these sets as a single connected set. The notion of a connected sum arose from the need to distinguish this sort of union from the notion of an unconnected or open-closed sum, that is, a union of disjoint sets such that the only connected subsets are those that are connected subsets of the summands in this union.

Comments

There are several obvious ways to implement the vague idea of a connected sum or union of spaces and sets: none particularly canonical. Definitions vary with the kind of objects under consideration.

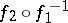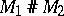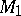The connected sum of two differentiable manifolds in differential topology is defined as follows. Let , be oriented (compact) -manifolds and let be the -dimensional unit disc. Let be an orientation-preserving imbedding, . Now paste together (identify) the boundaries of and by means of to obtain the connected sum of and 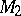. The orientation of 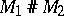is that of and the differentiable structure of is uniquely determined independent of 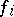. Up to a diffeomorphism, the operation of taking connected sums is associative and commutative. The -dimensional sphere serves as a zero element, i.e. is diffeomorphic to the -dimensional manifold .

References

[a1]	M.W. Hirsch, "Differential topology" , Springer (1976)