Conjugate directions
From Encyclopedia of Mathematics
A pair of directions emanating from a point 
on a surface 
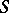such that the straight lines containing them are conjugate diameters of the Dupin indicatrix of 
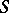at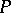 
. In order that the directions 
, 
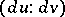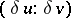at a point 
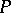on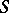 
be conjugate, it is necessary and sufficient that the following condition holds
 |
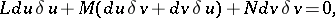where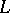 
, 
and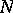 
are the coefficients of the second fundamental form of 
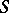evaluated at 
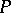. Example: a principal direction.
References
| [1] | A.V. Pogorelov, "Differential geometry" , Noordhoff (1959) (Translated from Russian) |
Comments
References
| [a1] | W. Blaschke, K. Leichtweiss, "Elementare Differentialgeometrie" , 1 , Springer (1973) |
| [a2] | C.C. Hsiung, "A first course in differential geometry" , Wiley (1981) pp. Chapt. 3, Sect. 4 |
How to Cite This Entry:
Conjugate directions. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Conjugate_directions&oldid=11253
Conjugate directions. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Conjugate_directions&oldid=11253
This article was adapted from an original article by E.V. Shikin (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article